REVIEW EXERCISESPrinted Page 9999
In Problems 1–3, evaluate each function.
f(x,y)=exlny
- (a) f(1,1)
- (b) f(x+Δx,y)
- (c) f(x,y+Δy)
(a) 0
(b) ex+Δx ln y
(c) ex ln(y + Δy)
f(x,y)=2x2+6xy−y3
- (a) f(1,1)
- (b) f(x+Δx,y)
- (c) f(x,y+Δy)
f(x,y,z)=exsin−1(y+2z)
- (a) f(ln3,12,14)
- (b) f(1,0,14)
- (c) f(0,0,0)
(a) 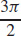
(b) 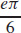
(c) 0
In Problems 4–7, find the domain of each function and graph the domain. Use a solid curve to indicate that the boundary is included and a dashed curve to indicate that the boundary is excluded.
z=f(x,y)=ln(x2−3y)
z=f(x,y)=√9−x2−4y2
{(x, y) | x2 + 4y2 ≤ 9}
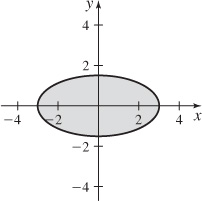
z=f(x,y)=25xy√5−y2
z=f(x,y)=(y−3x)2x+2y
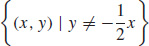
Find the domain of w=f(x,y,z)=y2+z2x2.
Find the domain of w=f(x,y,z)=ex+ylnz.
{(x, y, z) | z > 0}
In Problems 10–13, graph each surface.
z=f(x,y)=x−y+5
z=f(x,y)=sinx
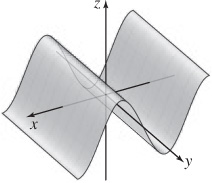
z=f(x,y)=lnx
z=f(x,y)=ey
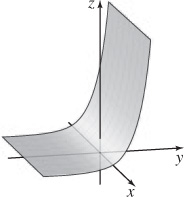
For z=f(x,y)=x2−2y, graph the level curves corresponding to c=−4, −1, 0, 1, 4.
For z=f(x,y)=√x2+y2, graph the level curves corresponding to c=0,1,4,9.
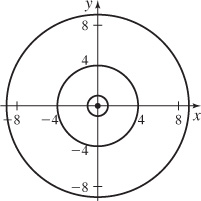
For z=f(x,y)=e4x2+y2, graph the level curves corresponding to c=1, e, e4, e16.
Describe the level surfaces associated with the function w=f(x,y,z)=4x2+y2+z2.
The level surfaces associated with the function w = 4x2 + y2 + z2 are ellipsoids and the point (0, 0, 0).
Describe the level surfaces associated with the function w=f(x,y,z)=x+y+2z.
Find lim.
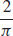
Find \lim\limits_{(x, y)\rightarrow (1, 2)}\dfrac{4x^{2}+y^{2} }{2x+y}.
Let f(x, y)=\dfrac{3xy^{2}}{x^{2}+y^{4}}.
- (a) Show that \lim\limits_{(x, y)\rightarrow (0, 0)}f(x, y)=0 along the lines y=mx.
- (b) Find \lim\limits_{(x, y)\rightarrow (0, 0)}f(x, y) along the parabola x=y^{2}.
- (c) What can you conclude?
(a) See Student Solutions Manual.
(b) 
(c) This limit does not exist.
860
Show that \lim\limits_{(x, y, z)\rightarrow (0, 0, 0)}\dfrac{ 4xy}{x^{2}+y^{2}+z^{2}} does not exist.
Determine where the function f(x,y) =2x^{2}y-y^{2}+3 is continuous.
The function is continuous at all points (x, y) in the plane.
Determine where the function R(x,y) =\dfrac{xy}{ x^{2}-y^{2}} is continuous.
- (a) Determine where the function f(x,y) =\tan ^{-1}\dfrac{1}{x^{2}+y^{2}} is continuous.
- (b) Find \lim\limits_{(x,y) \rightarrow ( 0,1) }\tan ^{-1}\dfrac{1}{x^{2}+y^{2}}.
(a) This function is continuous at all points (x, y) in the plane except the origin, (0, 0).
(b) 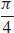
Find f_{x} and f_{y} for z=f(x, y)=e^{x^{2}+y^{2}}\sin (xy).
Find f_{x} and f_{y} for z=f(x, y)=\dfrac{x+y}{y}.
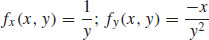
Find \dfrac{\partial z}{\partial x} and \dfrac{\partial z}{ \partial y} for z=f(x, y)=\sqrt{x-2y^{2}}.
Find \dfrac{\partial z}{\partial x} and \dfrac{\partial z}{ \partial y} for z=f(x, y)=e^{x}\ln ( 5x+2y) .
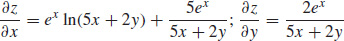
For f(x, y)=\sqrt{x^{2}-y^{2}}, find f_{x}(2,1) and f_{y}(2,-1).
For F(x, y)=e^{x}\sin y, find F_{x}\!\left( 0,\dfrac{\pi }{6} \right) and F_{y}\!\left( 0,\dfrac{\pi }{6}\right) .
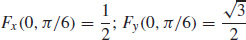
Find symmetric equations for the tangent line to the curve of intersection of the ellipsoid \dfrac{x^{2}}{24}+\dfrac{y^{2}}{12}+\dfrac{ z^{2}}{6}=1, and the plane y=1, where x=4 and z is positive.
Find symmetric equations for the tangent line to the curve of intersection of the surface z=4x^{2}-y^{2}+7:
- (a) With the plane y=-2 at the point (1,-2,7).
- (b) With the plane x=1 at the point (1,-2,7).
(a) y=-2, 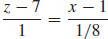
(b) x = 1, 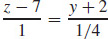
Boyle’s Law The volume V of a gas varies directly with the temperature T and inversely with the pressure P.
- (a) Find the rate of change of the volume V with respect to the temperature T.
- (b) Find the rate of change of the volume V with respect to the pressure P.
- (c) If T and P are functions of t, what is \dfrac{\partial V}{\partial t}?
In Problems 35 and 36, find the second-order partial derivatives f_{xx}, f_{xy}, f_{yx}, and f_{yy}.
z=f(x,y) =( x+y^{2}) e^{3x}
fxx (x, y) = 6e3x + 9(x + y2)e3x; fxy(x, y) = 6ye3x; fyx (x, y) = 6ye3x; fyy(x, y) = 2e3x
z=f(x,y) =\sec (xy)
In Problems 37–40, find f_{x}, f_{y}, and f_{z}.
w=f(x,y,z)=e^{xyz}
fx = yzexyz; fy = xzexyz; fz = xyexyz
w=f(x,y,z)=ze^{xy}
f(x,y,z)=e^{x}\sin y+e^{y}\sin z
fx = ex sin y; fy = ex cos y + ey sin z; fz = ey cos z
w=f(x,y,z)=z\tan ^{-1}\dfrac{y}{x}
For the function z=x^{3}y^{2}-2xy^{4}+3x^{2}y^{3}, show that x\dfrac{\partial z}{\partial x}+y\dfrac{\partial z}{\partial y}=5z.
See Student Solutions Manual.
Find the change \Delta z in z=f(x,y)=xy^{2}+2 from (x_{0},y_{0}) to (x_{0}+\Delta x, y_{0}+\Delta y). Use the answer to calculate the change in z from (1,0) to (0.9,0.2).
Show that the function z=f(x,y)=xy-5y^{2} is differentiable at any point (x,y) in its domain by:
- (a) Finding \Delta z.
- (b) Finding \eta _{1} and \eta _{2} so that \Delta z=f_{x}(x_{0},y_{0})\Delta x+ f_{y}(x_{0},y_{0})\Delta y+\eta _{1}\Delta x+\eta _{2}\Delta y.
- (c) Show that \lim\limits_{(\Delta x, \Delta y)\rightarrow (0, 0)}\eta_{1}=0 and \lim\limits_{(\Delta x, \Delta y)\rightarrow (0, 0)}\eta _{2}=0.
(a) Δz = (y)Δx + (x − 10y)Δy + (Δy)Δx + (−5Δy)Δy
(b) η1 = Δy; η2 = −5Δy
(c) See Student Solutions Manual.
In Problems 44 and 45, find the differential dz of each function.
z=x\sqrt{1+y^{2}}
z=\sin ^{-1}\dfrac{x}{y}, y>0
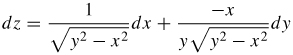
In Problems 46 and 47, find the differential dw of each function.
w=ze^{xy}
w=\ln (xyz)
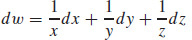
Use differentials to estimate the change in z=x\sqrt{1+y^{2}} from ( 4,0) to ( 4.1,0.1) .
Use differentials to estimate the change in z=\sin ^{-1}\dfrac{x}{y} from ( 0,1) to ( 0.1,1.1) .
\Delta z ≈ 0.1
Use the differential of f(x,y)=y^{2}\cos x to find an approximate value of f(0.05,1.98). (Compare your answer with a calculator result.)
Electricity The electrical resistance R of a wire is R=k \dfrac{L}{D^{2}} where L is the length of the wire, D is the diameter of the wire, and k is a constant. If L has a 1\% error and D has a 2\% error, what is the approximate maximum percentage error in the computation of R?
The approximate maximum percentage error is 5%.
Find \dfrac{dz}{dt} if z=\sin (xy)-x\sin y, where x=e^{t} and y=te^{t}.
Find \dfrac{dw}{dt} if w=\dfrac{x}{y}+\dfrac{y}{z}+\dfrac{z}{ x}, where x=\dfrac{1}{t}, y=\dfrac{1}{t^{2}}, and z=\dfrac{1}{t^{3}}.
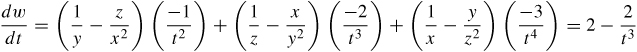
Find \dfrac{\partial w}{\partial u} and \dfrac{\partial w}{ \partial v} if w=xy+yz-xz, where x=u+v, y=uv, and z=v. Express each answer in terms of u and v.
Find \dfrac{\partial u}{\partial r} and \dfrac{\partial u}{ \partial s} if u=\sqrt{x^{2}+y^{2}+z^{2}}, where x=r\cos s, y=r\sin s , and z=\sqrt{r^{2}+s^{2}}. Express each answer in terms of r and s.
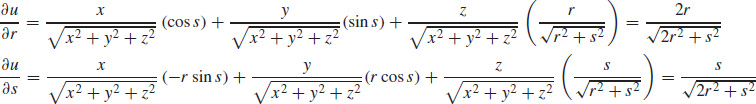
Find \dfrac{\partial z}{\partial x} and \dfrac{\partial z}{ \partial y} if z=f(x,y) is defined implicitly by F(x,y,z)=x^{2}+y^{2}-2xyz=0.
Find \dfrac{\partial z}{\partial x} and \dfrac{\partial z}{ \partial y} if z=f(x,y) is defined implicitly by F(x,y,z)=2x\sin y+2y\sin x+2xyz=0.
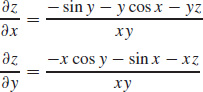
If z=uf( u^{2}+v^{2}) , show that 2v\dfrac{ \partial z}{\partial u}-2u\dfrac{\partial z}{\partial v}=\dfrac{2vz}{u}.