15.6 Assess Your UnderstandingPrinted Page 1025
1025
Concepts and Vocabulary
Multiple Choice A smooth surface has a [(a) normal plane, (b) tangent plane, (c) coordinate plane] at each point.
(b)
Multiple Choice Suppose x=x(u,v), y=y(u,v), z=z(u,v) are functions defined on a region R in the uv-plane. The set of points defined by (x,y,z)=(x(u,v),y(u,v),z(u,v)) is called a(n) [(a) real surface, (b) uniform surface, (c) parametric surface.
(c)
Multiple Choice The parametric equations x=5cos(u)sin(v), y=5sin(u)sin(v), z=5cos(v) define a [(a) plane, (b) circle, (c) sphere, (d) cylinder, (e) paraboloid].
(c)
Multiple Choice The parametrization r(u,v)=(1−u+2v)i+5uj+(2u+3v−7)k parametrizes a [(a) plane, (b) sphere, (c)line, (d) cylinder, (e) hyperboloid].
(a)
Skill Building
In Problems 5–8:
- (a) Identify the coordinate curves for u=0 and v=0 of each parametrization r(u,v)=x(u,v)i+y(u,v)j+z(u,v)k .
- (b) Find a rectangular equation for each parametric surface.
x(u,v)=u−5v,y(u,v)=2u,z(u,v)=−u+v+1
- (a) For u=0: the line r(0,v)=−5vi+(1+v)k; for v=0: the line r(u,0)=ui+2uj+(1−u)k.
- (b) x+2y+5z=5
x(u,v)=u,y(u,v)=v,z(u,v)=9−u2−v2
x(u,v)=ucosv,y(u,v)=usinv,z(u,v)=u;0≤u≤2, 0≤v≤π
- (a) For u=0: the point (0,0,0); for v=0: the line r(u,0)=ui+uk.
- (b) x2+y2=z2, 0≤z≤2
x(u,v)=cosusinv,y(u,v)=sinusinv,z(u,v)=cosv;0≤u≤2π,0≤v≤π2
In Problems 9–12, find a parametrization for each surface.
The part of the plane z=4−x−2y that lies in the first octant
r(u,v)=ui+vj+(4−u−2v)k; 0≤u≤4, 0≤v≤2−12u
The part of the surface z=e−x2+y2 that lies inside the cylinder x2+y2=4
The part of the surface z=sin(x2y) that lies above the region bounded by the graphs of y=x+2 and y=x2
r(u,v)=ui+vj+sin(u2v)k;−1≤u≤2,u2≤v≤u+2
The part of the surface y+exz=5 that lies inside the cylinder x2+z2=1
In Problems 13–16:
- (a) Find an equation of the tangent plane to each surface at the given point.
- (b) Find an equation of the normal line to the tangent plane at the point.
r(u,v)=(3u+2v)i+5u3j+v2k at (7,5,4)
- (a) 10x−2y−5z=40
- (b) r(t)=(7+10t)i+(5−2t)j+(4−5t)k
r(u,v)=(3u−v)i+(2−u−v)j+(1+3v)k at (11,1,−5)
r(u,v)=ui+ucosvj+usinvk at (5,5√22,5√22)
- (a) √2x−y−z=0
- (b) r(t)=(5+√2t)i+(5√22−t)j+(5√22−t)k
r(u,v)=(3cosusinv+1)i+(2sinusinv−1)j+cosvk at (1,0,√32)
In Problems 17–22, find the surface area of each surface S.
S is parameterized by r(u,v)=ucosvi+u3j+usinvk, 0≤u≤1, −π≤v≤π.
16π(3√10+ln(3+√10))
S is parameterized by r(u,v)=(3u2+v)i+u2j+(v−u2)k,0≤u≤2,−1≤v≤1.
S is the part of the plane 2x−y+4z=3 that lies inside the cylinder (x−2)2+y2=4.
√21π
S is the part of the paraboloid z=4−x2−y2 that lies above the xy-plane.
S is the part of the sphere x2+y2+z2=16 that lies above the plane z=2.
16 π
S is the frustum of the cone z=3√x2+y2 that lies between z=3 and z=6.
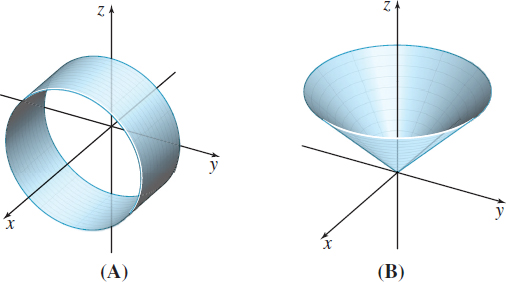
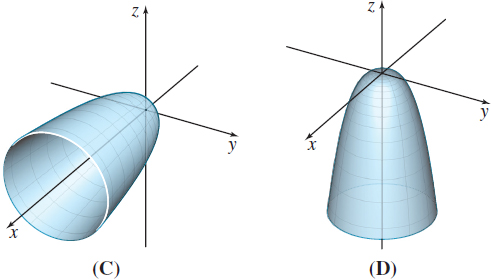
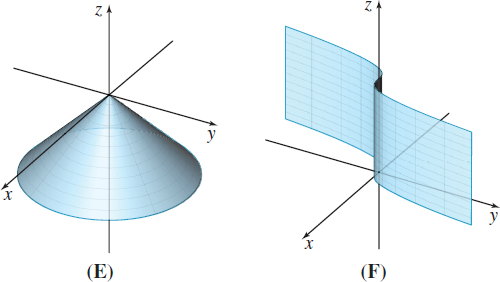
In Problems 23–28, match each parametrization with its parametric surface.
r (u,v)=ucosvi+usinvj−uk,0≤u≤4,0≤v≤2π
(E)
r (u,v)=u3i+usinvj+ucosvk,0≤u≤4,0≤v≤2π
r (u,v)=usinvi+ucosvj+uk,0≤u≤4,0≤v≤2π
(B)
r (u,v)=ui+4sinvj+4cosvk,0≤u≤4,0≤v≤2π
r (u,v)=ucosvi+usinvj−u3k,0≤u≤4,0≤v≤2π
(D)
r (u,v)=vi+v3j+uk,0≤u≤4,−4≤v≤4
1026
Applications and Extensions
Parametrize the part of the cylinder x2+y2=16 that lies above the xy-plane and below z=3.
r(u,v)=4cosui+4sinuj+vk; 0≤u≤2π, 0≤v≤3
Parametrize the sphere x2+y2+z2=25.
Part of the paraboloid z=9−x2−y2 lies inside the cylinder (x−1)2+y2=1.
- (a) Parametrize the surface using rectangular coordinates.
- (b) Parametrize the surface using cylindrical coordinates.
- (a) r(u,v)=ui+vj+(9−u2−v2)k, −√1−(u−1)2≤v≤√1−(u−1)2,0≤u≤2
- (b) r(r,θ)=rcosθi+rsinθj+(9−r2)k; 0≤r≤2,−π2≤θ≤π2
Parametrize the lumpy sphere x2+y2+z2=3√x2+y2+z2+z.
(Hint: Use spherical coordinates.)
In Problems 33 and 34, parametrize each surface:
- (a) Using rectangular coordinates.
- (b) Using cylindrical coordinates.
- (c) Using spherical coordinates.
Give bounds for the parameters, if necessary.
The part of the sphere x2+y2+z2=4 lying in the first octant
- (a) r(u,v)=ui+vj+√4−u2−v2k; 0≤u≤2,0≤v≤√4−u2
- (b) r(r,θ)=rcosθi+rsinθj+√4−r2k; 0≤r≤2, 0≤θ≤π2
- (c) r(θ,ϕ)=2cos(θ)sin(ϕ)i+2sin(θ)sin(ϕ)j+2cos(ϕ)k; 0≤θ≤π2, 0≤ϕ≤π2
The plane x−√3y=0, where x≥0, y≥0
Tangent Plane to a Torus
- (a) Find the tangent plane to the torus parametrized by r(u,v)=cosu(3+cosv)i+sinu(3+cosv)j+sinvk at the point (3√22,3√22,1)
- (b) Find an equation of the normal line to the tangent plane at the same point.
- (a) z=1
- (b) r(t)=3√22i+3√22j+(1+3t)k
Surface Area Find the surface area S of the torus parametrized by r(u,v)=(2+cosv)cosui+(2+cosv)sinuj+sinvk, 0≤u≤2π, 0≤v≤2π.
Surface Area Find the surface area S of the helicoid parametrized by r(u,v)=ucos(2v)i+usin(2v)j+vk, 0≤u≤1, 0≤v≤2π.
√5π+12πln(2+√5)
 Surface Area Find the surface area S of the Möbius strip parametrized by r(u,v)=(2cosu+vcosu2)i+(2sinu+vcosu2)j−vsinu2k, 0≤u≤2π, −0.5≤v≤0.5.
Surface Area Find the surface area S of the Möbius strip parametrized by r(u,v)=(2cosu+vcosu2)i+(2sinu+vcosu2)j−vsinu2k, 0≤u≤2π, −0.5≤v≤0.5.
Ellipsoid
- (a) Find a parametrization of the ellipsoid x2+y29+z24=1. (Hint: Use modified spherical coordinates.)
- (b)
 Graph the parametrized surface.
Graph the parametrized surface. - (c) Set up, but do not find, the double integral for the surface area of the parametrized ellipsoid.
- (a) r(θ,ϕ)=cos(θ)sin(ϕ)i+3sin(θ)sin(ϕ)j+2cos(ϕ)k; 0≤θ≤2π, 0≤ϕ≤π
- (b) See Student Solutions Manual.
- (c) S=∫2π0∫π0√36cos2θsin4ϕ+4sin2θsin4ϕ+9sin2ϕcosϕdϕdθ
 Helicoid
Helicoid- (a) Graph the helicoid defined by the parametric equations x=ucos(2v), y=usin(2v), z=v, 0≤u≤3 and 0≤v≤2π.
- (b) Parametrize the helicoid
- (c) Find an equation of the tangent plane to the helicoid at the point (13,π).
- (d) Find an equation of the normal line to the tangent plane at the point (13,π).
 Dini's Surface
Dini's Surface- (a) Graph Dini's surface defined by the parametric equations x=6cosusinvy=6sinusinv,z=6[cosv+ln(tanv2)]+u 0.01≤v≤1 and 0≤u≤6π.
- (b) Find an equation of the tangent plane to Dini's surface at the point (π3,π4).
- (c) Find an equation of the normal line to the tangent plane at the point (π3,π4).
- (a)
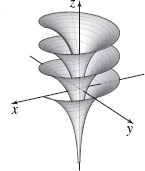
- (b) (9−32√6)x+(9√3+32√2)y−18z=−9+32√6−9√3−1112√2−108ln(√2−1)−6π
- (c) r(t)=(32√2+(9−32√6)t)i+(32√6+(9√3+32√2)t)j+((3√2+6ln(√2−1)+π3)−18t)k
Challenge Problems
Suppose ΔABC is a triangle with vertices A=(a1,a2,a3), B=(b1,b2,b3), and C=(c1,c2,c3) . Parametrize ΔABC.
Torus A torus is formed by rotating a circle about another circle.
- (a) Parametrize the torus obtained by rotating a circle of radius a>0 about a circle of radius b>0.
- (b) Find an implicit rectangular equation for the torus.
- (a) r(u,v)=(b+acosu)cosvi+(b+acosu)sinvj+asinuk
- (b) (b−√x2+y2)2+z2=a2
Surface Area Find the surface area of S, where S is the part of the cylinder (x−1)2+y2=1 outside the sphere x2+y2+z2=4, above the xy-plane, and below z=1.
An Archimedean Ratio Suppose C is a right circular cone, S is an upper hemisphere, and L is a right circular cylinder. Suppose that all three surfaces have radii r and the heights h of the cone and the cylinder are r. Archimedes was able to show that the ratio of the surface areas among these surfaces is √2:2:2. Show this by parametrizing each of these surfaces and deriving the formulas for their surface areas.
See Student Solutions Manual.
Hyperboloid of One Sheet
- (a) Use hyperbolic functions to parametrize the hyperboloid of one sheet: x2+y2−z2=c, c>0.
- (b)
 Graph the parametric surface for c=1.
Graph the parametric surface for c=1.
Hyperboloid of Two Sheets
- (a) Use hyperbolic functions to parametrize the sheet where x>0 in the hyperboloid of two sheets: x2a2−y2b2−z2c2=1.
- (b)
 Graph the parametric surface for a=1, b=2, c=3.
Graph the parametric surface for a=1, b=2, c=3.
- (a) r(u,v)=acoshui+(bsinhucosv)j+(csinhusinv)k
- (b)