Describe the vector field $\mathbf{F} (x,y)=3\mathbf{i+j}$ by drawing some of its vectors.

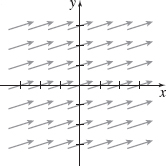
Describe the vector field $\mathbf{F} (x,y)=\sin x\mathbf{i+j}$ by drawing some of its vectors.

Find $\int_{C}\left( \dfrac{dx}{y}+\dfrac{dy}{x}\right)$, where $C$ is the arc of the parabola $y=x^{2}$ from $(1,1)$ to $(2,4)$.

Find $\int_{C}\left[ y\cos (xy) \,dx+x\cos (xy) \,dy\right]$, where $C$ is the curve $x=t^{2}$, $y=t^{3}$, $0\leq t\leq 1$.

Find $\int_{C}[(yz-y-z)\,dx+(xz-x-z)\,dy+(xy-x-y)\,dz]$, where $C$ is the twisted cubic $x=t$, $y=t^{2}$, $z=t^{3}$, $0\leq t\leq 1$.

Find $\int_{C}\mathbf{F}\,{\cdot}\, d\mathbf{r}$ if $\ \mathbf{F}(x,y,z)=xy\,\mathbf{i}+xz\,\mathbf{j}+(y-x)\,\mathbf{k}$ and $C$ is the line segment from $\left( 0,0,0\right)$ to $\left( 1,-2,3\right)$.

Find $\int_{C}[e^{x}\sin y\,dx+e^{x}\cos y\,dy]$, where $C$ is the arc of the parabola $y=x^{2}$ from $(0,0)$ to $(1,1)$.
(Hint: $\boldsymbol{\nabla \,}( e^{x}\sin y) = e^{x}\sin y\mathbf{i}+e^{x}\cos y\mathbf{j.})$

Mass of a Wire Find the mass of a thin piece of wire in the shape of a circular arc $x=\cos t$, $y=\sin t$, $0\leq t\leq \dfrac{3\pi }{4}$, if the variable mass density of the wire is $\rho (x,y)=x+1$.

Find the line integral $\int_{C}( x^{3}dx+y^{3}dy)$

Mass of a Lamina  A lamina is in the shape of the cone $z=\sqrt{ x^{2}+y^{2}},$ $1\leq z\leq 4$. If the mass density of the lamina is $\rho (x,y,z)=x^{2}+y^{2}$, find the mass $M$ of the lamina.

Find $\int_{C}[x^{2}y^{3}\,dx-xy^{4}\,dy]$, where $C$ is the arc of the parabola $y^{2}=x$ from $(0,0)$ to $(1,1)$.

Find $\int_{C}x^{2}y^{2}\,ds; C: x=\cos t, y=\sin t; 0\leq t\leq \pi.$

Work If $\mathbf{F}(x,y)=\dfrac{y}{(x^{2}+y^{2})^{3/2}}\mathbf{ i}-\dfrac{x}{(x^{2}+y^{2})^{3/2}}\mathbf{j}$, find the work done by going around the unit circle against $\mathbf{F}$ from $t=0$ to $t=2\pi$.

Work Find the work done by the force $\mathbf{F}=y\sin x \mathbf{i}+\sin x\mathbf{j}$ in moving an object along the curve $y=\sin x$ from $x=0$ to $x=2\pi$.

Work Find the work done by the force $$\mathbf{F}=\dfrac{x}{x^{2}+y^{2}}\mathbf{i}+\dfrac{y}{x^{2}+y^{2}}\mathbf{j}$$ in moving an object along the curve $\mathbf{r}(t)=t\cos t\mathbf{i}+t\sin t\mathbf{j}$ from $(-\pi ,0)$ to $(2\pi ,0)$.

Area Use Green's Theorem to find the area of the region enclosed by the curves $C_{1}$: $x(t) =t,$ $y(t) =t^{2}+3$ and $C_{2}$: $x(t) =t,$ $y(t) =30-2t^{2}.$

Use Green's Theorem to find the line integral $\int_{C}\left[ \ln \left( 1+y\right) dx+\dfrac{xy}{1+y}dy\right]$ where $C$ is the parallelogram with vertices $(0,0)$, $(2,1) ,$ $( 2,6) ,$ and $( 0,5)$ traversed counterclockwise.

Find $\oint_{C}(y^{2}\,dx-x^{2}\,dy)$, where $C$ is the square with vertices $(0,0),(1,0),(1,1)$, and $(0,1)$ traversed counterclockwise. Do not use Green's Theorem.

Rework Problem 21 using Green's Theorem.

Find $\oint_{C}[(x-y)\,dx+(x+y)\,dy]$, where $C$ is the ellipse $x=2\cos t$, $y=3\sin t$, $0\leq t\leq 2\pi$, without using Green's Theorem.

Rework Problem 23 using Green's Theorem.

Area Use Green's Theorem to find the area of the multiply connected region enclosed by the ellipse $\dfrac{x^{2}}{4}+\dfrac{y^{2}}{16} =1$ which has a small circular hole given by $x^{2}+\left( y-1\right) ^{2}=1$ punched out of its interior.

Find a parametrization of the part of the cylinder $4x^{2}+25y^{2}=100$ that lies above the plane $z=1$ and below the plane $z=6$.

Find the surface area of the part of the paraboloid $\mathbf{r} (u,v) =u\sin v\,\mathbf{i}+u^{2}\,\mathbf{j}+u\cos v\,\mathbf{k,}$ $0\leq u\leq 4,$ $0\leq v\leq 2\pi$.

Find $\iint\limits_{\kern-3ptS}x\,dS,$ where $S$ is the surface parametrized by $\mathbf{r}(u,v)=\cos v\,\mathbf{i}+3\sin u\sin v\,\mathbf{j} +3\cos u\sin v\mathbf{k}$, $0\leq u\leq 2\pi$ and $0\leq v\leq \pi /2$.

Find $\iint\limits_{\kern-3ptS}z^{2}\,dS$, where $S$ is the sphere $x^{2}+y^{2}+z^{2}=4$.

Find the outer unit normal vectors to the surface $S$ that forms the boundary of the solid $z=f(x,y)=\sqrt{25-x^{2}-y^{2}},$ $0\leq x^{2}+y^{2}\leq 25.$

Find $\iint\limits_{\kern-3ptS}x\,dS$, where $S$ is the surface $x^{2}+y^{2}=9$, $-1\leq z\leq 1$.

Find $\iint\limits_{\kern-3ptS}z\,dS$, where $S$ is the surface $z=9-x-y$, $x^{2}+y^{2}\leq 9$.

Find $\iint\limits_{\kern-3ptS}\cos x\,dS$, where $S$ is the portion of the plane $x=y+z,$ $x\leq \pi$, $\ y\geq 0,\,\ z\geq 0$.

A fluid has a constant mass density $\rho .$ Find the mass of fluid flowing across the surface $x^{2}+y^{2}=1,$ $\ 0\leq z\leq 1$ in a unit of time, in the direction outward from the $z$-axis if the velocity of the fluid at any point on the surface is $\mathbf{F}=x^{2}\mathbf{i}+y \mathbf{j}-z\mathbf{k}$.

$\mathbf{F}=z\cos x\mathbf{i}+\sin y\mathbf{j}+e^{x}\mathbf{k}$

$\mathbf{F}=x^{2}\mathbf{i}-3y\mathbf{j}+4z^{2}\mathbf{k}$

$\mathbf{F}=x\mathbf{i}+y\mathbf{j}+z\mathbf{k}$

$\mathbf{F}=xe^{y}\mathbf{i}-ye^{z}\mathbf{j}+ze^{x}\mathbf{k}$

Use the Divergence Theorem to find $\iint\limits_{\kern-3ptS}\mathbf{F}\,{\cdot}\, \mathbf{n}\,dS$, where $S$ is the surface bounded by $x^{2}+y^{2}=1$ and $0\leq z\leq 1,$ and $\mathbf{F}=x^{2} \mathbf{i}+y\,\mathbf{j}-z\mathbf{k}$.

Find $\iint\limits_{\kern-3ptS}(xz\cos \alpha +yz\cos \beta +x^{2}\cos \gamma )\,dS$, where $S$ is the upper half of the unit sphere together with the plane $z=0,$ and $\cos \alpha ,$ $\cos \beta ,$ and $\cos \gamma$ are the direction cosines for the outer unit normal to $S$.

Find $\iiint\limits_{\kern-13ptE}{div}\mathbf{F}\,dV$, where $E$ is the unit ball, $\left\Vert \mathbf{r}\right\Vert \leq 1$, and $\mathbf{F}= {\Vert \mathbf{r\Vert }}^{2}\mathbf{r}$.

Use Stokes' Theorem to find $\int_{C}[(x-y)\,dx+(y-z)\,dy+ (z-x) \,dz]$, where $C$ is the boundary of the portion of the plane $x+y+z=1,$ $x\geq 0,$ $y\geq 0,$ $z\geq 0$ (traversed counterclockwise when viewed from above).

Let $\mathbf{F}(x,y,z)=x^{3}\mathbf{i}+y^{3}\mathbf{j}+z^{3} \mathbf{k}$ be the velocity of a fluid flow in\br space, where the mass density of the fluid is $1$.

Determine if $\mathbf{F}( x,y,z) =yz\mathbf{i}+xy \mathbf{j}+xy\mathbf{k}$ is a conservative vector field.

Let $\mathbf{F}( x,y,z) =2xy^{2}z\,\mathbf{i} +2x^{2}yz\,\mathbf{j}+(x^{2}y^{2}-2z)\mathbf{k}$. Show that $\mathbf{F}$ is a conservative vector field.