REVIEW EXERCISESPrinted Page 9999
In Problems 1–4, state the order and degree of each differential equation. Determine whether the equation is linear or nonlinear.
\(\dfrac{dy}{dx}+x^{2}y=\sin x\)
This is a first-order differential equation of degree 1. The differential equation is linear.
\(\dfrac{d^{2}y}{dx^{2}}-5\dfrac{dy}{dx}+3y=xe^{x}\)
\(\dfrac{d^{3}y}{dx^{3}}+4x\dfrac{d^{2}y}{dx^{2}}+ ( \sin^{2}x) \dfrac{dy}{dx}+3y=\sin x\)
This is a third-order differential equation of degree 1. The differential equation is linear.
\(\left( {\dfrac{dr}{ds}}\right) ^{3}-r=1\)
In Problems 5–8, verify that the given function is a solution of the differential equation.
\(y=e^{x},\quad \dfrac{d^{2}y}{dx^{2}}-y=0\)
See Student Solutions Manual.
\(y=\dfrac{\ln x}{x^{2}},\quad \dfrac{d^{2}y}{dx^{2}}+\dfrac{5}{x}\dfrac{dy}{dx}+\dfrac{4}{x^{2}}y=0\)
\(y =e^{-3x},\quad \dfrac{d^{2}y}{dx^{2}}+2\dfrac{dy}{dx}-3y=0\)
See Student Solutions Manual.
\(y=x^{2}+3x, \quad x\dfrac{dy}{dx}-y=x^{2}\)
In Problems 9–12, determine if each function is homogeneous. If it is, state the degree.
\(f(x,y)=x^{3}+2x^{2}y+2xy^{2}+y^{3}\)
Function \(f\) is a homogeneous function of degree 3.
\(f(x,y)=x^{2}-x^{2}y^{2}+y^{2}\)
\(f(x,y)=xe^{y/x}\)
Function \(f\) is a homogeneous function of degree 1.
\(f(x,y)=\ln \dfrac{y}{x}\)
In Problems 13–24:
- (a) Identify each differential equation as separable, homogeneous, exact, linear, or Bernoulli.
- (b) Solve each differential equation.
\(x(y^{2}+1)\,dx+y(x^{2}-x)\,dy=0\)
- (a) The differential equation is separable.
- (b) \(y = \pm \sqrt {\displaystyle{C \over {(1 - x)^2}} - \mbox{1}}\)
\((2y+e^{2x})\,dx+(2x+e^{2y})\,dy=0\)
\(x\dfrac{dy}{dx}+y=x^{6}\)
- (a) The differential equation is first-order linear and exact.
- (b) \(y = \displaystyle{\mbox{1} \over 7}{x}^{\rm 6} + \displaystyle{\mbox{C} \over x}\)
\(\dfrac{dy}{dx}-2y=e^{3x}\)
\((2xy^{3}+y^{2}\,\cos x-2x)\,dx+(3x^{2}y^{2}+2y\,\sin x)\,dy=0\)
- (a) The differential equation is exact.
- (b) \(x^2y^3 + y^2\;\sin\;x - x^2 + C = 0\)
\(\dfrac{dy}{dx}+x^{2}y-y=x^{2}-1\)
\(y\,\sin xdx-\,\cos x\,dy\,{=}\,0\)
- (a) The differential equation is separable and exact.
- (b) \(y = \displaystyle{C \over {{\rm cos}\;x}}\)
\(( 2x\,\sin y-\ln y)\,dx+\left( x^{2}\,\cos y-\dfrac{x}{y}+3y^{2}\right)dy=0\)
\(\left( x-y\, \tan \dfrac{y}{x}\right)dx+x\,\tan \dfrac{y}{x}\,dy=0\)
- (a) The differential equation is homogeneous of degree 1.
- (b) \(y = x\;\cos ^{ - 1}(Cx)\)
\(x\dfrac{dy}{dx}-2y=x+1\)
\(\dfrac{dy}{dx}+\dfrac{2}{x}y=\dfrac{y^{3}}{x^{2}}\)
- (a) The differential equation is Bernoulli.
- (b) \(y = \pm \sqrt {\displaystyle{{5x} \over {2 + \mbox{C}x^5}}}\)
\(\dfrac{dy}{dx}-5y+2y^{2}=0\)
In Problems 25–34, find the particular solution of each differential equation.
\(x\dfrac{dy}{dx}+2y=6,\quad y(2)=8\)
\(y = 3 + \displaystyle{{20} \over {x^2}}\)
\(xy\,dy=\big( y^{2}+x\sqrt{x^{2}+y^{2}}\big)\,dx,\quad y(1)=1\)
\(\dfrac{dy}{dx}+\dfrac{y}{x}=2,\quad y(1)=0\)
\(y = x - \dfrac{1}{x}\)
\((x^{2}+xy+y^{2})\,dx-x^{2}dy=0,\quad y(1)=1\)
\(\dfrac{dy}{dx}=\dfrac{y-1}{x+3},\quad y(-1)=0\)
\(y = \displaystyle{{ - 1} \over 2} - \dfrac{1}{2}x\)
\(( 1-\cos x)\,\dfrac{dy}{dx}+y\,\sin x=0,\quad y\left( \dfrac{\pi }{4}\right) =1\)
\(\dfrac{dy}{dx}-\dfrac{2y}{x}=x^{2}\,\cos x,\quad y\left( \dfrac{\pi }{2}\right) =3\)
\(y = x^2\sin\;x + \left(\displaystyle{{12} \over {\pi ^2}} - 1\right)x^2\)
\(\left( \dfrac{2x-1}{y}\right)dx+x\left( \dfrac{1-x}{y^{2}}\right)dy=0,\quad y(-1)=3\)
\((x^{2}+y^{2})\,dx-xy\,dy=0,\quad y(1)=2\)
\(y = x\sqrt {4 + 2\;\ln\;x}\)
\(\ y^{2}\sin x\,dx+\left( \dfrac{1}{x}-\dfrac{y}{x}\right)dy=0,\quad y(\pi )=1\)
In Problems 35 and 36, find a power series solution for each differential equation.
\((x-2)y^{\prime} +y=0\)
\(y(x) = a_0 \sum\limits_{k = 0}^\infty {\left( {\displaystyle{x \over 2}} \right)^k}\)
\(y''+y' -2y=0\)
In Problems 37 and 38, (a) Use a Maclaurin series to find a solution for each differential equation.
(b) Use the first five terms of the series to approximate values of \(y=y(x)\) for \(0\leq x\leq 1\).
\(y^{\prime \prime} +( \sin x) y=0,\quad y( 0) =1;\qquad y^{\prime} ( 0) =1\)
- (a) \(y(x) = 1 + x - \displaystyle{1 \over 6}x^3 - \displaystyle{1 \over {12}}x^4 + \displaystyle{1 \over {120}}x^5 + \ldots .\)
- (b)
\(x\) 0 0.1 0.2 0.3 0.4 0.5 0.6 0.7 0.8 0.9 1.0 \(y(x)\) 1.00000 1.09983 1.19854 1.29485 1.38729 1.47422 1.55385 1.62423 1.68326 1.72875 1.75833
\(y^{\prime \prime} +e^{x}y^{\prime} +y=0,\quad y( 0) =1;\qquad y^{\prime} ( 0)=1\)
Population Growth Suppose that the available food supply will support a maximum number \(M\) of bacteria, and that the rate of growth of the bacteria is proportional to the difference between the maximum number and the number present. Find an expression for the number of bacteria as a function of time.
\(y = M - Ce^{ - kt}\)
Flow in Mixtures A large tank contains 1000 L of water in which 500 mg of chlorine are dissolved. Water containing 2 mg of dissolved chlorine per liter flows into the tank at the rate of 100 L/min. The mixture, kept uniform by stirring, runs out of the tank at the rate of 80 L/min. How much chlorine is in the tank after an hour?
Orthogonal Trajectories Find the orthogonal trajectories of the family of hyperbolas \(xy=c\). Graph a member of each family that contains the point \((2,3)\).
The orthogonal trajectories for the family \(xy = c\) is the family \(y^{2}- x^{2}=K\). The graph of xy = 6 and \(y^{2}\) – \(x^{2}\) = 5 is shown.
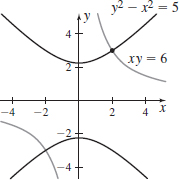
Spread of Rumors After a large chip manufacturer posts poor quarterly earnings, the rumor of widespread layoffs spreads among the employees. The rumor spreads throughout the \(100{,}000\) employees at a rate that is proportional to the product of the number of employees who have heard the rumor and those who have not. If \(10\) employees started the rumor, and it has spread to \(100\) employees after \(1\,\rm{h}\), how long will it take for \(25\%\) of the employees to have heard the rumor?