P.1 Assess Your UnderstandingPrinted Page 12
Concepts and Vocabulary
If f is a function defined by y=f(x), then x is called the _____ variable and y is the _____ variable.
Independent, dependent
True or False The independent variable is sometimes referred to as the argument of the function.
True
True or False If no domain is specified for a function f, then the domain of f is taken to be the set of all real numbers.
False
True or False The domain of the function f(x)=3(x2−1)x−1 is {x|x≠±1}.
False
True or False A function can have more than one y-intercept.
False
A set of points in the xy-plane is the graph of a function if and only if every _____ line intersects the graph in at most one point.
Vertical
If the point (5,−3) is on the graph of f, then f( _____ ) = _____.
5,−3
Find a so that the point (−1,2) is on the graph of f(x)=ax2+4.
−2
Multiple Choice A function f is [(a) increasing, (b) decreasing, (c) nonincreasing, (d) nondecreasing, (e) constant] on an interval I if, for any choice of x1 and x2 in I, with x1<x2, then f(x1)<f(x2).
(a)
Multiple Choice A function f is [(a) even, (b) odd, (c) neither even nor odd] if for every number x in its domain, the number −x is also in the domain and f(−x)=f(x). A function f is [(a) even, (b) odd, (c) neither even nor odd] if for every number x in its domain, the number −x is also in the domain and f(−x)=−f(x).
(a), (b)
13
True or False Even functions have graphs that are symmetric with respect to the origin.
False
The average rate of change of f(x)=2x3−3 from 0 to 2 is _____.
8
Practice Problems
In Problems 13–16, for each function find:
- (a) f(0)
- (b) f(−x)
- (c) −f(x)
- (d) f(x+1)
- (e) f(x+h)
f(x)=3x2+2x−4
- (a) −4
- (b) 3x2−2x−4
- (c) −3x2−2x+4
- (d) 3x2+8x+1
- (e) 3x2+6xh+3h2+2x+2h−4
f(x)=xx2+1
f(x)=|x|+4
- (a) 4
- (b) |x|+4
- (c) −|x|−4
- (d) |x+1|+4
- (e) |x+h|+4
f(x)=√3−x
In Problems 17–22, find the domain of each function.
f(x)=x3−1
(−∞,∞)
f(x)=xx2+1
v(t)=√t2−9
(−∞,−3]∪[3,∞)
g(x)=√2x−1
h(x)=x+2x3−4x
{x|x≠−2,0,2}
s(t)=√t+1t−5
In Problems 23–28, find the difference quotient of f. That is, find f(x+h)−f(x)h, h≠0.
f(x)=−3x+1
−3
f(x)=1x+3
f(x)=√x+7
1√x+h+7+√x+7
f(x)=2√x+7
f(x)=x2+2x
2x+h+2
f(x)=(2x+3)2
In Problems 29–32, determine whether the graph is that of a function by using the Vertical-line Test. If it is, use the graph to find
- (a) the domain and range
- (b) the intercepts, if any
- (c) any symmetry with respect to the x-axis, y-axis, or the origin.
It is not a function.
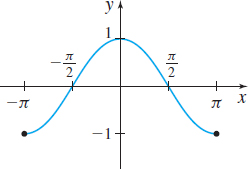
- (a) Domain: [−π,π], range: [−1,1]
- (b) Intercepts: (−π2,0),(π2,0),(0,1)
- (c) Symmetric with respect to the y-axis, but not with respect to the x-axis or the origin.
In Problems 33–36, for each piecewise-defined function:
- (a) Find f(−1), f(0), f(1) and f(8).
- (b) Graph f.
- (c) Find the domain, range, and intercepts of f.
f(x)={x+3if−2≤x<15ifx=1−x+2ifx>1
- (a) f(−1)=2, f(0)=3, f(1)=5, f(8)=−6
- (b)
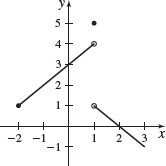
- (c) Domain: [−2,∞), range: (−∞,4)∪{5}, intercepts: (0,3), (2,0)
f(x)={2x+5if−3≤x<0−3ifx=0−5xifx>0
f(x)={1+xifx<0x2ifx≥0
- (a) f(−1)=0, f(0)=0, f(1)=1, f(8)=64
- (b)
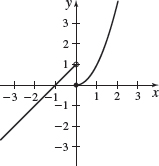
- (c) Domain: (−∞,∞), range: (−∞,∞), intercepts: (−1,0), (0,0)
f(x)={1xifx<03√xifx≥0
In Problems 37–54, use the graph of the function f to answer the following questions.
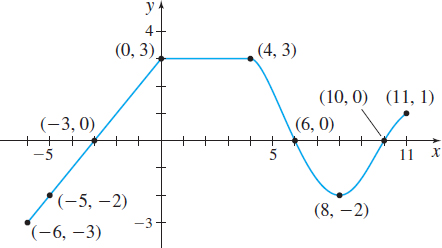
Find f(0) and f(−6).
f(0)=3, f(−6)=−3
Is f(3) positive or negative?
Is f(−4) positive or negative?
Negative
For what values of x is f(x)=0?
For what values of x is f(x)>0?
(−3,6)∪(10,11]
What is the domain of f?
What is the range of f?
[−3,3]
What are the x-intercepts?
What is the y-intercept?
3
How often does the line y=12 intersect the graph?
How often does the line x=5 intersect the graph?
Once
For what values of x does f(x)=3?
For what values of x does f(x)=−2?
−5, 8
On what interval(s) is the function f increasing?
On what interval(s) is the function f decreasing?
(4,8)
On what interval(s) is the function f constant?
On what interval(s) is the function f nonincreasing?
(0,8)
On what interval(s) is the function f nondecreasing?
14
In Problems 55–60, answer the questions about the function g(x)=x+2x−6.
What is the domain of g?
{x|x≠6}
Is the point (3,14) on the graph of g?
If x=4, what is g(x)? What is the corresponding point on the graph of g?
−3, (4,−3)
If g(x)=2, what is x? What is(are) the corresponding point(s) on the graph of g?
List the x-intercepts, if any, of the graph of g.
−2
What is the y-intercept, if there is one, of the graph of g?
In Problems 61–64, determine whether the function is even, odd, or neither. Then determine whether its graph is symmetric with respect to the y-axis, the origin, or neither.
h(x)=xx2−1
Odd; symmetric with respect to the origin, but not with respect to the x-axis or the y-axis.
f(x)=3√3x2+1
G(x)=√x
Neither; not symmetric to the x-axis, y-axis, or the origin.
F(x)=2x|x|
Find the average rate of change of f(x)=−2x2+4:
- (a) From 1 to 2
- (b) From 1 to 3
- (c) From 1 to 4
- (d) From 1 to x, x≠1
- (a) −4
- (b) −8
- (c) −10
- (d) −2(x+1)
Find the average rate of change of s(t)=20−0.8t2:
- (a) From 1 to 4
- (b) From 1 to 3
- (c) From 1 to 2
- (d) From 1 to t, t≠1
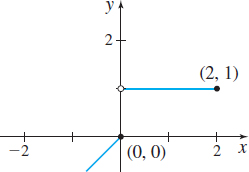
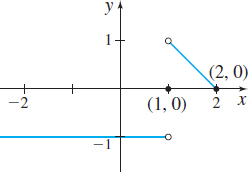
In Problems 67–72, the graph of a piecewise-defined function is given. Write a definition for each piecewise-defined function. Then state the domain and the range of the function.
f(x)={−x if−1≤x<012x if0≤x≤2 domain: [−1,2], range: [0,1]
f(x)={−1 ifx<10 ifx=12−x if1<x≤2 domain: (−∞,2], range: {−1}∪[0,1)
- (a) $250.60
- (b) $2655.40
- (c) Answers will vary.
The monthly cost C, in dollars, of manufacturing x road bikes is given by the function C(x)=0.004x3−0.6x2+250x+100,500
- (a) Find the average rate of change of the cost C of manufacturing from 100 to 101 road bikes.
- (b) Find the average rate of change of the cost C of manufacturing from 500 to 501 road bikes.
- (c) Interpret the results from parts (a) and (b).
The weekly cost in dollars to produce x tons of steel is given by the function C(x)=110x2+5x+1500
- (a) Find the average rate of change of the cost C of producing from 500 to 501 tons of steel.
- (b) Find the average rate of change of the cost C of producing from 1000 to 1001 tons of steel.
- (c) Interpret the results from parts (a) and (b).