P.2 Assess Your UnderstandingPrinted Page 22
Concepts and Vocabulary
Multiple Choice The function f(x)=x2 is [(a) increasing, (b) decreasing, (c) neither] on the interval (0,∞).
(a)
True or False The floor function f(x)=⌊x⌋ is an example of a step function.
True
True or False The cube function is odd and is increasing on the interval (−∞,∞).
True
True or False The cube root function is odd and is decreasing on the interval (−∞,∞).
False
True or False The domain and the range of the reciprocal function are all real numbers.
False
A number r for which f(r)=0 is called a(n) _____ of the function f.
Zero
Multiple Choice If r is a zero of even multiplicity of a function f, the graph of f [(a) crosses, (b) touches, (c) doesn't intersect] the x-axis at r.
(b)
True or False The x-intercepts of the graph of a polynomial function are called zeros of the function.
True
True or False The function f(x)=[x+5√x2−π]2/3 is an algebraic function.
True
True or False The domain of every rational function is the set of all real numbers.
False
Practice Problems
In Problems 11–18, match each graph to its function:
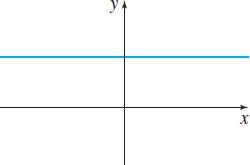
- Constant function
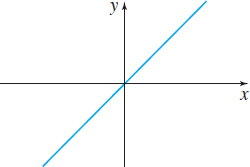
- Identity function
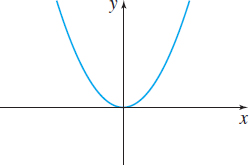
- Square function
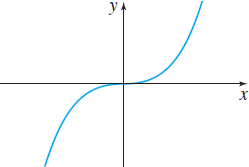
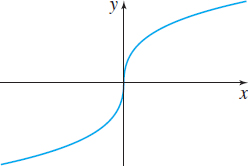
- Cube function
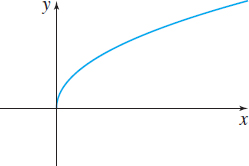
- Square root function
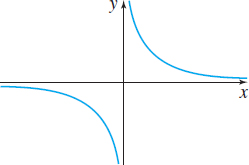
- Reciprocal function
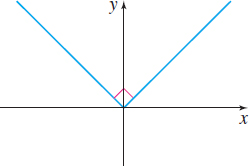
- Absolute value function
- Cube root function
D
F
23
C
G
If f(x)=⌊2x⌋, find
- (a) f(1.2),
- (b) f(1.6),
- (c) f(−1.8).
- (a) 2
- (b) 3
- (c) −4
If f(x)=⌈x2⌉, find
- (a) f(1.2),
- (b) f(1.6),
- (c) f(−1.8).
In Problems 21 and 22, for each polynomial function f:
- (a) List each real zero and its multiplicity.
- (b) Find the x- and y-intercepts of the graph of f.
- (c) Determine whether the graph of f crosses or touches the x-axis at each x-intercept.
f(x)=3(x−7)(x+4)3
- (a) 7 with multiplicity 1; −4 with multiplicity 3
- (b) x-intercepts: 7, −4, y-intercept: −1344
- (c) Crosses at 7 and at −4
f(x)=4x(x2+1)(x−2)3
In Problems 23 and 24, decide which of the polynomial functions in the list might have the given graph. (More than one answer is possible.)
- (a) f(x)=−4x(x−1)(x−2)
- (b) f(x)=x2(x−1)2(x−2)
- (c) f(x)=3x(x−1)(x−2)
- (d) f(x)=x(x−1)2(x−2)2
- (e) f(x)=x3(x−1)(x−2)
- (f) f(x)=−x(1−x)(x−2)
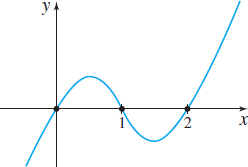
(c), (e), (f)
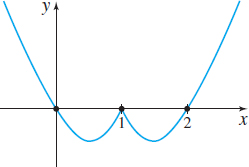
- (a) f(x)=2x3(x−1)(x−2)2
- (b) f(x)=x2(x−1)(x−2)
- (c) f(x)=x3(x−1)2(x−2)
- (d) f(x)=x2(x−1)2(x−2)2
- (e) f(x)=5x(x−1)2(x−2)
- (f) f(x)=−2x(x−1)2(2−x)
In Problems 25–28, find the domain and the intercepts of each rational function.
R(x)=5x2x+3
Domain: {x|x≠−3}, intercept: (0,0)
H(x)=−4x2(x−2)(x+4)
R(x)=3x2−xx2+4
Domain: (−∞,∞), intercepts: (0,0), (13,0)
R(x)=3(x2−x−6)4(x2−9)
Constructing a Model The rectangle shown in the figure has one corner in quadrant I on the graph of y=16−x2, another corner at the origin, and corners on both the positive y-axis and the positive x-axis. As the corner on y=16−x2 changes, a variety of rectangles are obtained.
- (a) Express the area A of the rectangles as a function of x.
- (b) What is the domain of A?
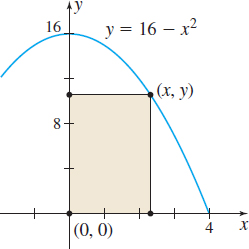
- (a) A(x)=16x−x3
- (b) [0,4]
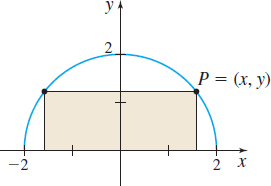
Constructing a Model The rectangle shown in the figure is inscribed in a semicircle of radius 2. Let P=(x,y) be the point in quadrant I that is a vertex of the rectangle and is on the circle. As the point (x,y) on the circle changes, a variety of rectangles are obtained.
- (a) Express the area A of the rectangles as a function of x.
- (b) Express the perimeter p of the rectangles as a function of x.
 Height of a Ball A ballplayer throws a ball at an inclination of 45∘ to the horizontal. The following data represent the height h (in feet) of the ball at the instant that it has traveled x feet horizontally:
Height of a Ball A ballplayer throws a ball at an inclination of 45∘ to the horizontal. The following data represent the height h (in feet) of the ball at the instant that it has traveled x feet horizontally:Distance, x 20 40 60 80 100 120 140 160 180 200 Height, h 25 40 55 65 71 77 77 75 71 64 - (a) Draw a scatter plot of the data. Comment on the type of relation that may exist between the two variables.
- (b) Use technology to verify that the quadratic function of best fit to these data is h(x)=−0.0037x2+1.03x+5.7 Use this function to determine the horizontal distance the ball travels before it hits the ground.
- (c) Approximate the height of the ball when it has traveled 10 feet.
- (a) The pattern in the scatter plot suggests a quadratic relationship.
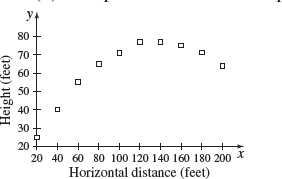
- (b) 283.8 feet
- (c) 15.63 feet
 Educational Attainment The following data represent the percentage of the U.S. population whose age is x (in years) who did not have a high school diploma as of January 2011:
Educational Attainment The following data represent the percentage of the U.S. population whose age is x (in years) who did not have a high school diploma as of January 2011:Age, x 30 40 50 60 70 80 Percentage without a High School Diploma, P 11.6 11.7 10.4 10.4 17.0 24.6 Source: U.S. Census Bureau.
- (a) Draw a scatter plot of the data, treating age as the independent variable. Comment on the type of relation that may exist between the two variables.
- (b) Use technology to verify that the cubic function of best fit to these data is P(x)=0.00026x3−0.0303x2+1.0877x−0.5071
- (c) Use this model to predict the percentage of 35-year-olds who do not have a high school diploma.