2.5 Assess Your UnderstandingPrinted Page 190
Concepts and Vocabulary
True or False ddxcosx=sinx
False
True or False ddxtanx=cotx
False
True or False d2dx2sinx=−sinx
True
True or False ddxsinπ3=cosπ3
False
Skill Building
In Problems 5–8, find the derivative of f at c.
f(x)=x−sinx, c=π
f′(π)=2
f(θ)=2sinθ+cosθ, c=π2
f(θ)=cosθ1+sinθ, c=π3
f′(π/3)=−4+2√3
f(x)=sinx1+cosx, c=5π6
In Problems 9–38, find y′.
y=3sinθ−2cosθ
y′=3cosθ+2sinθ
y=4tanθ+sinθ
y=sinxcosx
y′=cos2x−sin2x=cos2x
y=cotxtanx
y=tcost
y′=cost−tsint
y=t2tant
y=extanx
y′=ex(sec2x+tanx)
y=exsecx
y=πsecutanu
y′=πsecu(sec2u+tan2u)
y=πutanu
y=cotxx
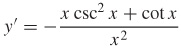
y=cscxx
y=x2sinx
y′=x(xcosx+2sinx)
y=t2tant
y=ttant−√3sect
y′=tsec2t+tant−√3secttant
y=xsecx+√2cotx
y=sinθ1−cosθ
y′=1cosθ−1
y=xcosx
y=sint1+t
y′=(cost+tcost−sint)/(1+t)2
y=tanu1+u
y=sinxex
y′=(cosx−sinx)/ex
y=excosx
y=sinθ+cosθsinθ−cosθ
y′=−2/(sinθ−cosθ)2
y=sinθ−cosθsinθ+cosθ
y=sect1+tsint
y′=(secttant+ttan2t−t−tant)/(1+tsint)2
y=csct1+tcost
y=cscθcotθ
y′=−cscθ(csc2θ+cot2θ)
y=tanθcosθ
y=1+tanx1−tanx
y′=2sec2x/(1−tanx)2
y=cscx−cotxcscx+cotx
In Problems 39–50, find y″.
y=\sin x
y''=-\sin x
y=\cos x
y=\tan \theta
y''=2\tan \theta\sec^{2}\theta
y=\sec \theta
y=t\sin t
y''=2\cos t-t\sin t
y=t\cos t
y=e^{x}\sin x
y''=2e^{x}\cos x
y=e^{x}\cos x
y=2\sin u-3\cos u
y''=3\cos u-2\sin u
y=3\sin u+4\cos u
y=a\sin x+b\cos x
y''=-(a\sin x+b\cos x)
y=a\sec\theta +b\tan \theta
In Problems 51–56:
- (a) Find an equation of the tangent line to the graph of f at the indicated point.
- (b)
 Graph the function and the tangent line on the same screen.
Graph the function and the tangent line on the same screen.
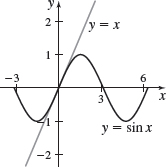
f(x)=\sin x at (0,0)
- (a) y=x
- (b)
f(x)=\cos x at {\left( \dfrac{\pi }{3},\dfrac{1}{2}\right) }
f(x)=\tan x at (0,0)
- (a) y=x
- (b)
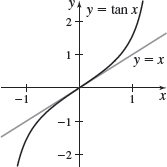
f(x)=\tan x at {\left( \dfrac{\pi }{4},1\right)}
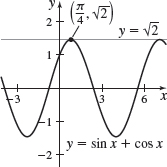
f(x)=\sin x+\cos x at {\left( \dfrac{\pi }{4},\sqrt{2}\right)}
- (a) y=\sqrt{2}
- (b)
f(x)=\sin x-\cos x at {\left( \dfrac{\pi }{4},0\right) }
191
In Problems 57-60:
- (a) Find all points on the graph of f where the tangent line is horizontal.
- (b)
 Graph the function and the horizontal tangent lines on the interval [-2\pi ,2\pi ] on the same screen.
Graph the function and the horizontal tangent lines on the interval [-2\pi ,2\pi ] on the same screen.
f(x)=2\sin x+\cos x
- (a) \big\{\big(\tan^{-1}2 + 2n\pi, \sqrt{5}\big)\big\}, \big\{\big(\tan^{-1}2 + (2n+1)\pi, -\sqrt{5}\big)\big\}, where n is an integer
- (b)
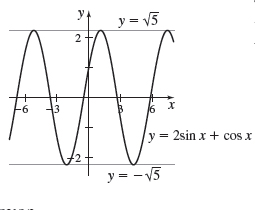
f(x)=\cos x-\sin x
f(x)=\sec x
- (a) \{(2n\pi, 1)\}, \{((2n+1)\pi,-1)\}, where n is an integer
- (b)
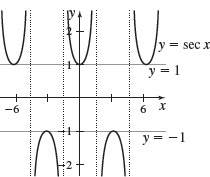
f(x)=\csc x
Applications and Extensions
In Problems 61 and 62, find the nth derivative of each function.
f(x)=\sin x
f^{(n)}(x)=\left\{ \begin{array}{ll} (-1)^{\frac{n}{2}}\sin x&n\ {\rm even}\\[3pt] (-1)^{\frac{n-1}{2}}\cos x&n\ {\rm odd} \end{array} \right.
f(\theta )=\cos \theta
What is {\lim\limits_{h\rightarrow 0}\dfrac{\cos \left( { \dfrac{\pi }{2}}+h\right) -\cos {\dfrac{\pi }{2}}}{h}?}
-1
What is \lim\limits_{h\rightarrow 0}{\dfrac{\sin (\pi +h)-\sin \pi }{h}?}
Simple Harmonic Motion The distance s (in meters) of an object from the origin at time t (in seconds) is modeled by the function s( t) =\dfrac{1}{8}\cos t.
- (a) Find the velocity v of the object.
- (b) When is the speed of the object at a maximum?
- (c) Find the acceleration a of the object.
- (d) When is the acceleration equal to 0?
- (e)
 Graph s, v, and a on the same screen.
Graph s, v, and a on the same screen.
- (a) v(t)=-\dfrac{1}{8}\sin t m/s
- (b) \dfrac{\pi}{2}+n\pi, where n is an integer
- (c) a(t)=-\dfrac{1}{8}\cos t\ {\rm m}/{\rm s}^{2}
- (d) \dfrac{\pi}{2}+n\pi, where n is an integer
- (e)

Simple Harmonic Motion An object attached to a coiled spring is pulled down a distance d= 5~{\text{cm}} from its equilibrium position and then released as shown in the figure. The motion of the object at time t seconds is simple harmonic and is modeled by d(t) =-5\cos t. 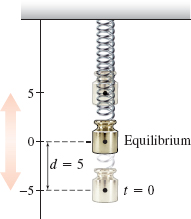
- (a) As t varies from 0 to 2\pi, how does the length of the spring vary?
- (b) Find the velocity v of the object.
- (c) When is the speed of the object at a maximum?
- (d) Find the acceleration a of the object.
- (e) When is the acceleration equal to 0?
- (f)
 Graph d, v, and a on the same screen.
Graph d, v, and a on the same screen.
Rate of Change A large, 8-ft high decorative mirror is placed on a wood floor and leaned against a wall. The weight of the mirror and the slickness of the floor cause the mirror to slip.
- (a) If \theta is the angle between the top of the mirror and the wall, and y is the distance from the floor to the top of the mirror, what is the rate of change of y with respect to \theta ?
- (b) In feet/radian, how fast is the top of the mirror slipping down the wall when \theta =\dfrac{\pi }{4}?
- (a) \dfrac{dy}{d\theta}=-8\sin\theta
- (b) \dfrac{dy}{d\theta} =-4\sqrt{2}\approx 5.657 ft/radian
Rate of Change The sides of an isosceles triangle are sliding outward. See the figure. 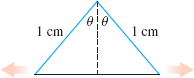
- (a) Find the rate of change of the area of the triangle with respect to \theta.
- (b) How fast is the area changing when \theta =\dfrac{\pi }{6}?
Sea Waves Waves in deep water tend to have the symmetric form of the function f(x)=\sin x. As they approach shore, however, the sea floor creates drag which changes the shape of the wave. The trough of the wave widens and the height of the wave increases, so the top of the wave is no longer symmetric with the trough. This type of wave can be represented by a function such as w(x)=\dfrac{4}{2+\cos x}.
Source: http://www.crd.bc.ca/watersheds/protection/geology-processes/waves.htm
- (a) Graph w=w(x) for 0 ≤ x ≤ 4\pi.
- (b) What is the maximum and the minimum value of w?
- (c) Find the values of x, 0 < x < 4\pi, at which w'(x)=0.
- (d) Evaluate w’ near the peak at \pi, using x=\pi-0.1, and near the trough at 2\pi, using x=2\pi-0.1.
- (e) Explain how these values confirm a non-symmetric wave shape.
- (a)
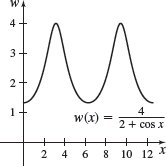
- (b) Max = 4, Min = \dfrac{4}{3}
- (c) x=\pi, 2\pi, 3\pi
- (d) w'(\pi-0.1)\approx 0.395, w'(2\pi-0.1)\approx -0.045
- (e) Answers will vary.
Swinging Pendulum A simple pendulum is a small-sized ball swinging from a light string. As it swings, the supporting string makes an angle \theta with the vertical. See the figure. At an angle \theta, the tension in the string is T=\dfrac{W}{\cos \theta }, where W is the weight of the swinging ball. 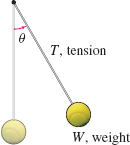
- (a) Find the rate of change of the tension T with respect to \theta when the pendulum is at its highest point (\theta =\theta _{\max }).
- (b) Find the rate of change of the tension T with respect to \theta when the pendulum is at its lowest point.
- (c) What is the tension at the lowest point?
Restaurant Sales A restaurant in Naples, Florida is very busy during the winter months and extremely slow over the summer. But every year the restaurant grows its sales. Suppose over the next two years, the revenue R, in units of $10,000, is projected to follow the model R=R( t) =\sin t+0.3t+1 \qquad 0 ≤ t ≤ 12 where t=0 corresponds to November 1, 2014; t=1 corresponds to January 1, 2015; t=2 corresponds to March 1, 2015; and so on.
- (a) What is the projected revenue for November 1, 2014; March 1, 2015; September 1, 2015; and January 1, 2016?
192
- (b) What is the rate of change of revenue with respect to time?
- (c) What is the rate of change of revenue with respect to time for January 1, 2016?
- (d)
 Graph the revenue function and the derivative function R^\prime = R’(t).
Graph the revenue function and the derivative function R^\prime = R’(t). - (e) Does the graph of R support the facts that every year the restaurant grows its sales and that sales are higher during the winter and lower during the summer? Explain.
- (a) What is the projected revenue for November 1, 2014; March 1, 2015; September 1, 2015; and January 1, 2016?
- (a) $10,000, $25,093, $15,411, $37,570
- (b) R'(t)=\cos t+0.3
- (c) 10,540 dollars/2-months
- (d)
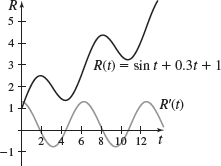
- (e) Answers will vary.
Polarizing Sunglasses Polarizing sunglasses are filters that only transmit light for which the electric field oscillations are in a specific direction. Light gets polarized naturally by scattering off the molecules in the atmosphere and by reflecting off of many (but not all) types of surfaces. If light of intensity I_{0} is already polarized in a certain direction, and the transmission direction of the polarizing filter makes an angle with that direction, then the intensity I of the light after passing through the filter is given by Malus’s Law, I( \theta ) =I_{0}\cos ^{2}\theta . 
- (a) As you rotate a polarizing filter, \theta changes. Find the rate of change of the light intensity I with respect to \theta .
- (b) Find both the intensity I(\theta) and the rate of change of the intensity with respect to \theta , for the angles \theta =0° , 45° , and 90°. (Remember to use radians for \theta.)
If y=\sin x and y^{(n)} is the nth derivative of y with respect to x find the smallest positive integer n for which y^{(n)}=y.
n=4
Use the identity \sin A-\sin B=2\cos \dfrac{A+B}{2}\sin \dfrac{A-B}{2}, with A=x+h and B=x, to prove that \dfrac{d}{dx}\sin x= \lim\limits_{h\rightarrow 0}\dfrac{\sin (x+h)-\sin x}{h}=\cos x
Use the definition of a derivative to prove \dfrac{d }{dx}\cos x=-\sin x.
See Student Solutions Manual.
Derivative of {y=\sec x} Use a derivative rule to show that \dfrac{d}{dx}\sec x=\sec x\tan x.
Derivative of y=csc x Use a derivative rule to show that \dfrac{d}{dx}\csc x=-\csc x\cot x.
See Student Solutions Manual.
Derivative of {y=\cot x} Use a derivative rule to show that \dfrac{d}{dx}\cot x=-\csc ^{2}x.
Let f(x)=\cos x. Show that finding f^\prime (0) is the same as finding \lim\limits_{x\rightarrow 0}{\dfrac{\cos x-1}{x}}.
See Student Solutions Manual.
Let f(x)=\sin x. Show that finding f^\prime (0) is the same as finding \lim\limits_{x\rightarrow 0}{\dfrac{\sin x}{x}}.
If y=A\sin t+B\cos t, where A and B are constants, show that y''+y=0.
See Student Solutions Manual.
Challenge Problem
For a differentiable function f, let f^{\ast } be the function defined by f^{\ast }(x)=\lim\limits_{h\rightarrow 0}\frac{f(x+h)-f(x-h)}{h}
- (a) Find f^{\ast }(x) for f(x)=x^{2}+x.
- (b) Find f^{\ast }(x) for f(x)=\cos x.
- (c) Write an equation that expresses the relationship between the functions f^{\ast } and f^\prime, where f^\prime denotes the derivative of f. Justify your answer.
