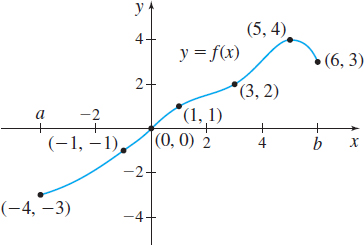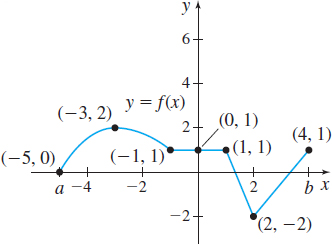5.2 Assess Your UnderstandingPrinted Page 359
Concepts and Vocabulary
If an interval [a,b] is partitioned into n subintervals [x0,x1], [x1,x2], [x2,x3], …, [xn−1,xn], where a=x0<x1<x2<⋯<xn−1<xn=b, then the set of subintervals of the interval [a,b] is called a(n) ________ of [a,b].
Partition
Multiple Choice In a regular partition of [0,40] into 20 subintervals, Δx= [(a) 20 (b) 40 (c) 2 (d) 4].
(c) 2
True or False A function f defined on the closed interval [a,b] has an infinite number of Riemann sums.
True
In the notation for a definite integral ∫baf(x)dx, a is called the _______ _______; b is called the _______ _______; ∫ is called the _________ _________; and f(x) is called the_________.
Lower limit of integration; upper limit of integration; integral sign; integrand
If f(a) is defined, ∫aaf(x)dx= _________.
0
True or False If a function f is integrable over a closed interval [a,b], then ∫baf(x)dx=∫abf(x)dx.
False
True or False If a function f is continuous on a closed interval [a,b], then the definite integral ∫baf(x)dx exists.
True
Multiple Choice Since ∫20(3x−8)dx=−10, then ∫02(3x−8)dx= [(a) −2 (b) 10 (c) 5 (d) 0].
(b) 10
Skill Building
In Problems 9–12, find the Riemann sum for each function f for the partition and the numbers ui listed.
f(x)=x, 0≤x≤2. Partition the interval [0,2] as follows: x0=0,x1=14,x2=12,x3=34,x4=1,x5=2;[0,14],[14,12],[12,34],[34,1],[1,2]
and choose u1=18,u2=38,u3=58,u4=78,u5=98.
138
f(x)=x, 0≤x≤2. Partition the interval [0,2] as follows: [0,12], [12,1], [1,32], [32,2], and choose u1=12, u2=1, u3=32, u4=2.
f(x)=x2, −2≤x≤1. Partition the interval [−2,1] as follows: [−2,−1], [−1,0], [0,1] and choose u1=−32, u2=−12, u3=12.
114
360
f(x)=x2, 1≤x≤2. Partition the interval [1,2] as follows: [1,54], [54,32], [32,74], [74,2] and choose u1=54, u2=32, u3=74, u4=2.
In Problems 13 and 14, the graph of a function f defined on an interval [a,b] is given.
- (a) Partition the interval [a,b] into six subintervals (not necessarily of the same size using the points shown on each graph).
- (b) Approximate ∫baf(x)dx by choosing ui as the left endpoint of each subinterval and using Riemann sums.
- (c) Approximate ∫baf(x)dx by choosing ui as the right endpoint of each subinterval and using Riemann sums.
- (a) [−4,−1],[−1,0],[0,1],[1,3],[3,5],[5,6].
- (b) 0
- (c) 13
In Problems 15–22, write the limit of the Riemann sums as a definite integral. Here ui is in the integral [xi−1,xi], i=1,2,…n.
lim on [0,2]
\int_0^2(e^x+2) dx
\lim\limits_{{\max \Delta x}_{i}\rightarrow 0}\sum\limits_{i=1}^{n}\ln u_{i}\Delta x_{i} on [1,8]
\lim\limits_{{\max \Delta x}_{i} \rightarrow 0}\sum\limits_{i=1}^{n}\cos u_{i}\Delta x_{i} on [0,2\pi]
\int_0^{2\pi} \cos x dx
\lim\limits_{{\max \Delta x}_{i} \rightarrow 0}\sum\limits_{i=1}^{n}\left( \cos u_{i}+\sin u_{i}\right) \Delta x_{i} on [0,\pi]
\lim\limits_{{\max \Delta x}_{i} \rightarrow 0}\sum\limits_{i=1}^{n}{\dfrac{{2}}{{u^{2}_i}}}\Delta x_{i} on [1,4]
\int_1^4 \dfrac{2}{x^2} dx
\lim\limits_{{\max \Delta x}_{i} \rightarrow 0}\sum\limits_{i=1}^{n}u^{1/3}_i\Delta x_{i} on [0,8]
\lim\limits_{{\max \Delta x}_{i} \rightarrow 0}\sum\limits_{i=1}^{n}u_{i}\ln u_{i} \Delta x_{i} on [1, e]
\int_1^e x\ln x dx
\lim\limits_{{\max \Delta x}_{i} \rightarrow 0} \sum\limits_{i=1}^{n}\ln (u_{i}+1)\Delta x_{i} on [0, e]
In Problems 23–28, find each definite integral.
\int_{-3}^{4}e\,dx
7e
\int_{0}^{3}(-\pi) \,dx
\int_{3}^{0}(-\pi)\, dt
3\pi
\int_{7}^{2}2\, ds
\int_{4}^{4}2\, \theta\ d\theta
0
\int_{-1}^{-1}8\, dr
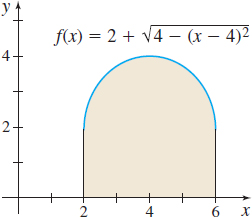
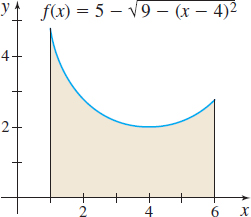
In Problems 29–32, the graph of a function is shown. Express the shaded area as a definite integral.
\int_2^6\big(2+\sqrt{4 - (x - 4)^2}\big)\, dx
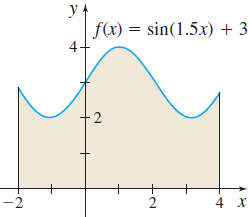
\int_{-2}^4 (3+\sin(1.5x))\, dx
In Problems 33–38, determine which of the following definite integrals can be interpreted as area. For those that can, describe the area; for those that cannot, explain why.
\int_{0}^{\pi }\sin x\,dx
Yes; answers will vary.
\int_{-\pi /4}^{\pi /4}\tan x\,dx
\int_{1}^{4}(x-2) ^{1/3}dx
No; answers will vary.
\int_{1}^{4}(x+2) ^{1/3}dx
\int_{1}^{4}(\vert x \vert \,-2 ) \,dx
No; answers will vary.
\int_{-2}^{4}\vert x \vert \,\,dx
In Problems 39–44:
- (a) For each function defined on the given interval, use a regular partition to form Riemann sums \sum\limits_{i=1}^{n} f(u_{i})\Delta x_{i}.
- (b) Express the limit as n\rightarrow \infty of the Riemann sums as a definite integral.
- (c)
 Use a computer algebra system to find the value of the definite integral in (b).
Use a computer algebra system to find the value of the definite integral in (b).
f(x) =x^{2}-1 on [0,2]
- (a) \sum\limits_{i=1}^n\left(u^2_i-1 \right)\left(\dfrac{2}{n} \right)
- (b) \int_0^2 (x^2-1) dx
- (c) \dfrac{2}{3}
f(x) =x^{3}-2 on [0,5]
f(x) =\sqrt{x+1} on [0,3]
- (a) \sum\limits_{i=1}^n (\sqrt{u_i+1}) \left(\dfrac{3}{n}\right)
- (b) \int_0^3 (\sqrt{x+1})dx
- (c) \dfrac{14}{3}
f(x) =\sin x on [0, \pi]
f(x) =e^{x} on [0, 2]
- (a) \sum\limits_{i=1}^n (e^{u_i}) \left(\dfrac{2}{n}\right)
- (b) \int_0^2 e^x\, dx
- (c) e^2-1
f(x) =e^{-x} on [0,1]
361
In Problems 45 and 46, find each definite integral using Riemann sums.
{\int_{0}^{1}(x - 4)dx}
-\dfrac{7}{2}
\int_{0}^{3}{(3x - 1)dx}
![]() In Problems 47–50, for each function defined on the interval [a,b]:
In Problems 47–50, for each function defined on the interval [a,b]:
- (a) Complete the table of Riemann sums using a regular partition of [a,b].
n 10 50 100 Using left endpoints Using right endpoints Using the midpoint - (b) Use a CAS to find the definite integral.
f(x) =2+\sqrt{x} on [1,5]
- (a)
n 10 50 100 Left 14.536 14.737 14.762 Right 15.030 14.836 14.812 Mid 14.789 14.787 14.787 - (b) {\approx} 14.787
f(x) =e^{x}+e^{-x} on [-1,3]
f(x) =\dfrac{3}{1+x^{2}} on [-1,1]
- (a)
n 10 50 100 Left 4.702 4.712 4.712 Right 4.702 4.712 4.712 Mid 4.717 4.713 4.712 - (b) \dfrac{3\pi}{2}\approx 4.712
f(x) = \dfrac{1}{\sqrt{x^2+4}} on [0,2]
Applications and Extensions
Find an approximate value of {\int_{1}^{2}}\dfrac{1}{x}\,{dx} by finding Riemann sums corresponding to a partition of [1,2] into four subintervals, each of the same length, and evaluating the integrand at the midpoint of each subinterval. Compare your answer with the true value, 0.6931\ldots .
\dfrac{4448}{6435} \approx 0.691

- (a) Find the approximate value of {\int_{0}^{2} \sqrt{4 - x^{2}}}\,dx by finding Riemann sums corresponding to a partition of [0, 2] into 16 subintervals, each of the same length, and evaluating the integrand at the left endpoint of each subinterval.
- (b) Can \int_{0}^{2}\sqrt{4-x^{2}}\, dx be interpreted as area? If it can, describe the area; if it cannot, explain why.
- (c) Find the actual value of \int_{0}^{2}\sqrt{4-x^{2}}dx by graphing y=\sqrt{4-x^{2}} and using a familiar formula from geometry.
Units of an Integral In the definite integral \int_{0}^{5}F(x)\, dx, F represents a force measured in newtons and x, 0\leq x\leq 5, is measured in meters. What are the units of \int_{0}^{5}F(x)\, dx?
newton-meters
Units of an Integral In the definite integral \int_{0}^{50}C(x)\, dx, C represents the concentration of a drug in grams per liter and x, 0\leq x\leq 50, is measured in liters of alcohol. What are the units of \int_{0}^{50}C(x)\, dx?
Units of an Integral In the definite integral \int_{a}^{b}v(t)\, dt, v represents velocity measured in meters per second and time t is measured in seconds. What are the units of \int_{a}^{b}v(t)\, dt?
meters
Units of an Integral In the definite integral \int_{a}^{b}S(t)\, dt, S represents the rate of sales of a corporation measured in millions of dollars per year and time t is measured in years. What are the units of \int_{a}^{b}S(t)\, dt?
 Area
Area- (a) Graph the function f(x) =3-\sqrt{6x-x^{2}}.
- (b) Find the area under the graph of f from 0 to 6.
- (c) Confirm the answer to (b) using geometry.
- (a)
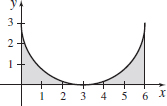
- (b) 18-\dfrac{9\pi}{2}\approx 3.863
- (c) See Student Solutions Manual.
 Area
Area- (a) Graph the function f(x) = \sqrt{4x-x^{2}}+2.
- (b) Find the area under the graph of f from 0 to 4.
- (c) Confirm the answer to (b) using geometry.
The interval [1,5] is partitioned into eight subintervals each of the same length.
- (a) What is the largest Riemann sum of f(x)=x^{2} that can be found using this partition?
- (b) What is the smallest Riemann sum?
- (c) Compute the average of these sums.
- (d) What integral has been approximated, and what is the integral's exact value?
- (a) \dfrac{95}{2}
- (b) \dfrac{71}{2}
- (c) \dfrac{83}{2}=41.5
- (d) \int_1^5 x^2~dx=41.\overline{3}
Challenge Problems
The floor function f(x) = \lfloor x\rfloor is not continuous on [0,4]. Show that {\int_{0}^{4}{f(x)\,dx}} exists.
Consider the Dirichlet function f, where f(x)=\left\{ \begin{array}{@{}l@{ }l@{ }l} {1} & \hbox{if} & {x}~\hbox{is rational} \\ {0} & \hbox{if} & {x}~\hbox{is irrational} \end{array} \right. Show that {\int_{0}^{1}{f(x)\,dx}} does not exist. (Hint: Evaluate the Riemann sums in two different ways: first by using rational numbers for { u}_{i} and then by using irrational number seak for { u}_{i}.)
See Student Solutions Manual.
It can be shown (with a certain amount of work) that if f(x) is integrable on the interval [a,b], then so is \vert f(x) \vert . Is the converse true?
If only regular partitions are allowed, then we could not always partition an interval [a,b] in a way that automatically partitions subintervals [a,c] and [c,b] for a\lt c\lt\,b. Why not?
See Student Solutions Manual.
If f is a function that is continuous on a closed interval [a,b] , except at x_{1}, x_{2}, \ldots , x_{n}, n\geq 1 an integer, where it has a jump discontinuity, show that f is integrable on [a,b] .
If f is a function that is continuous on a closed interval [a,b] , except at x_{1}, x_{2}, \ldots , x_{n}, n\geq 1 an integer, where it has a removable discontinuity, show that f is integrableon [a,b] .
See Student Solutions Manual.