6.6 Assess Your UnderstandingPrinted Page 450
Concepts and Vocabulary
True or False Work is the energy transferred to or from an object by a force acting on the object.
True
The work W done by a constant force F in moving an object a distance x along a straight line in the direction of F is _____.
W=Fx
A unit of work is called a _____, in SI units and a _____-_____, in the customary U.S. system of units.
joule; foot-pound
The work W done by a continuously varying force F=F(x) acting on an object, which moves the object along a straight line in the direction of F from x=a to x=b, is given by the definite integral _____.
W=∫baF(x) dx
451
A spring is said to be in _____when it is neither extended nor compressed.
Equilibrium
True or False The force F required to extend or compress a spring when it is either extended or compressed x units is F=−kx, where k is the spring constant.
True
True or False The mass density ρ of a fluid is defined as mass per unit volume ( kg/m3) and is a constant that depends on the type of fluid.
True
True or False Newton's Law of Universal Gravitation affirms that every body in the universe attracts every other body, and that the force F attracting two bodies is proportional to the product of the masses of both bodies and inversely proportional to the square of the distance between them.
8252 J
Skill Building
How much work is done by a variable force F(x)=(40−x) N that moves an object along a straight line in the direction of F from x=5 m to x=20 m?
True
How much work is done by a variable force F(x)=1xN that moves an object along a straight line in the direction of F from x=1 m to x=2 m?
A 40 m chain weighing 3 kg/m hangs over the side of a bridge. How much work is done by a winch that winds the entire chain in?
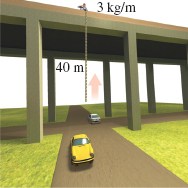
23,520 J
A 120 ft chain weighing 240 lb is dangling from the roof of an apartment building. How much work is done in pulling the entire chain up to the roof?
A force of 3 N is required to keep a spring extended 14 m beyond its equilibrium length. What is its spring constant?
k=12 N/m
A force of 6 lb is required to keep a spring compressed to 12 ft shorter than its equilibrium length. What is its spring constant?
A spring with spring constant k=5 has an equilibrium length of 0.8 m. How much work is required to stretch the spring to 1.4 m?
−0.9 J
A spring with spring constant k=0.3 has an equilibrium length of 1.2 m. How much work is required to compress the spring to1 m?
Pumping Water out of a Pool A swimming pool in the shape of a right circular cylinder, with height 4 ft and radius 12 ft, is full of water. See the figure below. How much work is required to pump all the water over the top of the pool? (The weight density of water is 62.42 lb/ft3.
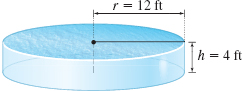
W=71907.84π ft lb
Pumping Gasoline out of a Tank A gasoline storage tank in the shape of a right circular cylinder, with height 10 m and radius 8 m, is full of gasoline. How much work is required to pump all the gasoline over the top of the tank? (The density of gasoline is ρ=720 kg/m3.)
Pumping Corn Slurry A container in the shape of an inverted pyramid with a square base of 2 m by 2 m and height of 5 m is filled to a depth of 4 m with corn slurry. How much work is required to pump all the slurry over the top of the container? (The density of corn slurry is ρ=17.9 kg/m3.)
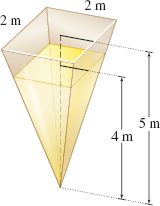
W≈1197.53 J
Pumping Olive Oil from a Vat A vat in the shape of an inverted pyramid with a rectangular base that measures 2 m by 0.5 m and height that measures 4 m is filled to a depth of 2 m with olive oil. How much work is required to pump all the olive oil over the top of the vat? (The density of olive oil is ρ=0.9/cm3.)
Applications and Extensions
Work to Lift an Elevator How much work is required if six cables, each weighing 0.36 lb/in., lift a 10,000-lb elevator 400 ft? Assume the cables work together and equally share the weight of the elevator.
6,073,600 ft lb
Work by Gravity A cable with a uniform linear mass density of 9 kg/m is being unwound from a cylindrical drum. If 15 m are already unwound, what is the work done by gravity in unwinding another 60 m of the cable?
Work to Lift a Bucket and Chain A uniform chain 10 m long and with mass 20 kg is hanging from the top of a building 10 m high. If a bucket filled with cement of mass 75 kg is attached to the end of the chain, how much work is required to pull the bucket and chain to the top of the building?
8,330 J
Work to Lift a Bucket and Chain In Problem 23, if a uniform chain 10 m long and with mass 15 kg is used instead, how much work is required to pull the bucket and chain to the top of the building?
Work of a Spring A spring, whose equilibrium length is 1m, extends to a length of 3 m when a force of 3 N is applied. Find the work needed to extend the spring to a length of 2 m from its equilibrium length.
−34 J
Work of a Spring A spring, whose equilibrium length is 2 m, is compressed to a length of 12 m when a force of 10 N is applied. Find the work required to compress the spring to a length of 1 m.
Work of a Spring A spring, whose equilibrium length is 4ft, extends to a length of 8ft when a force of 2 lb is applied. If 9 ft-lb of work is required to extend this spring from its equilibrium position, what is its total length?
10 ft
Work of a Spring If 8ft-lb of work is used on the spring in Problem 27, how far is it extended?
452
Work to Pump Water A full water tank in the shape of an inverted right circular cone is 8m across the top and 4m high. How much work is required to pump all the water over the top of the tank? (The density of water is 1000kg/m3.)
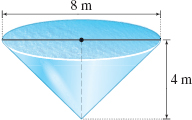
627,200π3J
Work to Pump Water If the surface of the water in the tank of Problem 29 is 2 m below the top of the tank, how much work is required to pump all the water over the top of the tank?
Work to Pump Water A water tank in the shape of a hemispherical bowl of radius 4 m is filled with water to a depth of 2 m. How much work is required to pump all the water over the top of the tank?
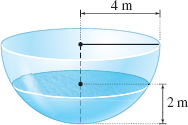
352,800π J
Work to Pump Water If the water tank in Problem 31 is completely filled with water, how much work is required to pump all the water to a height 2m above the tank?
Work to Pump Water A cylindrical tank, 4m in diameter and 6m high, is full of water. How much work is required to pump half the water over the top of the tank? (The density of water is 1000kg/m3.)
176,400π J
Work to Pump Water A swimming pool is in the shape of a rectangular parallelepiped 6ft deep, 30ft long, and 20ft wide. It is filled with water to a depth of 5ft. How much work is required to pump all the water over the top? (Hint: The weight of water is ρg=62.42lb/ft3.)
Work to Pump Water A 1 hp motor can do 550ft-lb of work per second. The motor is used to pump the water out of a swimming pool in the shape of a rectangular parallelepiped 5ft deep, 25ft long, and 15ft wide. How long does it take for the pump to empty the pool if the pool is filled to a depth of 4ft? (The weight of water is 62.42 lb/ft3.)
t≈8.5 min
Newton's Law of Universal Gravitation In Problems 36 and 37, use Newton's Law of Universal Gravitation F(x)=Gm1m2x2.
The minimum energy required to move an object of mass 30 kg a distance 30 km above the surface of Earth is equal to the work required to accomplish this. Find the work required. (Earth's radius R is approximately 6370km.)
The minimum energy required to move a rocket of mass 1000 kg a distance of 800 km above Earth's surface is equal to the work required to do this. Find the work required.
6.965×109 J
Coulomb's Law By Coulomb's Law, a positive charge m of electricity repels a unit of positive charge at a distance x with the force mx2. What is the work done when the unit charge is carried from x=2a to x=a, a>0?
Work to Lift a Leaky Load In raising a leaky bucket from the bottom of a well 25ft deep, one-fourth of the water is lost. If the bucket weighs 1.5lb, the water in the bucket at the start weighs 20lb, and the amount that has leaked out is assumed to be proportional to the distance the bucket is lifted, find the work done in raising the bucket. Ignore the weight of the rope used to lift the bucket.
475 ft-lb
Work of a Spring The spring constant on a bumping post in a freight yard is 300,000N/m. Find the work done in compressing the spring 0.1m.
Work to Pump Water A container is formed by revolving the region bounded by the graph of y=x2, and the x-axis, 0≤x≤2, about the y-axis. How much work is required to fill the container with water from a source 2 units below the x-axis by pumping through a hole in the bottom of the container?
80π3
Work of a Spring The force in newtons required to stretch a spring xmeters beyond its equilibrium length is given by F(x)=−100x.
- (a) What force will stretch the spring 0.1m? 0.2m? 0.4m?
- (b) How much work is required to stretch the spring 0.1m? 0.2m? 0.4m?
source: Adapted from F.W.Sears, M.W.Zemansky, & H.D.Young (1976), (University physics) (p. 132), Reading, MA: Addison-Wesley. Reprinted by permission.
 Work to Pump Heating Oil A cylindrical tank 6 m long and 4~m in diameter is lying on its side and is half full of heating oil. (The density of heating oil is 820kg/m3.)
Work to Pump Heating Oil A cylindrical tank 6 m long and 4~m in diameter is lying on its side and is half full of heating oil. (The density of heating oil is 820kg/m3.)- (a) Set up an integral to find the work done in pumping the oil over the top of the tank. Choose x=0 for the position of the base of the tank.
- (b) Find the work done in pumping the oil over the top of the tank to the nearest joule.
- (a) 96432∫20(4−x)√4x−x2 dx
- (b) W≈863052 J
Challenge Problems
Work to Move a Piston The force exerted by a gas in a cylinder on a piston whose area is A, is given by F=pA, where p is the force per unit area, or pressure. The work W in displacing the piston from x1 to x2 is W=∫x2x1F dx=∫x2x1pA dx=∫V2V1p dV
where dV is the accompanying infinitesimal change of volume of the gas.
- (a) During expansion of a gas at constant temperature (isothermal), the pressure depends on the volume according to the relation
p=nRTV
where n and R are constants and T is the constant temperature. Calculate the work in expanding the gas isothermally from volume V1 to volume V2.
- (b) During expansion of a gas at constant entropy (adiabatic), the pressure depends on the volume according to the relation
p=KVγ
where K and γ≠1 are constants. Calculate the work in expanding the gas adiabatically from V1 to V2.
- (a) During expansion of a gas at constant temperature (isothermal), the pressure depends on the volume according to the relation
453
Expanding Gases Problems 45 and 46 use the following discussion. The pressure p (in pounds/square inch, lb/in2) and the volume V (in cubic inches) of an adiabatic expansion of a gas are related by pVk=c, where k and c are constants that depend on the gas. If the gas expands from V=a to V=b, the work done (in inch-pounds) is W=∫baPdV.
Work of Expanding Gas The pressure of 1 lb of a gas is 100 lb\in2 and the volume is 2 ft3. Find the work done by the gas in expanding to double its volume according to the law pV1.4=c (in pounds per cubic foot).
2.092×105 in-lb
Work of Expanding Gas The pressure and volume of a certain gas obey the law pV1.2=120 (in inch-pounds). Find the work done when the gas expands from V=2.4 to V=4.6 in3.
