6.2 Assess Your UnderstandingPrinted Page 422
Concepts and Vocabulary
If a function f is continuous on a closed interval [a,b], then the volume V of the solid of revolution obtained by revolving the region bounded by the graph of f, the x-axis, and the lines x=a and x=b about the x-axis, is found using the formula V=_____.
π∫ba[f(x)]2 dx
True or False When the region bounded by the graphs of the functions f and g and the lines x=a and x=b is revolved about the x-axis, the cross section exposed by making a slice at ui perpendicular to the x-axis is two concentric circles, and the area Ai between the circles is Ai=π[f(ui)−g(ui)]2.
False
True or False If the functions f and g are continuous on the closed interval [a,b] and if f(x)≥g(x)≥0 on the interval, then the volume V of the solid of revolution obtained by revolving the region bounded by the graphs of f and g and the lines x=a and x=b about the x-axis is V=π∫ba[f(x)−g(x)]2 dx.
False
True or False If the region bounded by the graphs of y=x2 and y=2x is revolved about the line y=6, the volume V of the solid of revolution generated is found by finding the integral V=π∫76(4x2−x4)dx.
False
Skill Building
In Problems 5–10, find the volume of the solid of revolution generated by revolving the region shown below about the indicated axis.
y=2√x about the x-axis
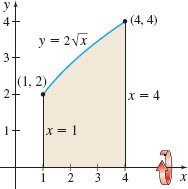
V=30π
y=x4 about the y-axis
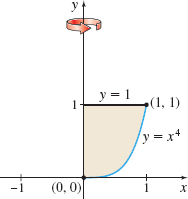
423
y=1x about the y-axis
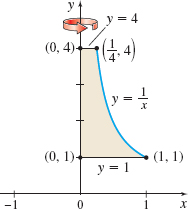
3π4
y=x2/3 about the y-axis
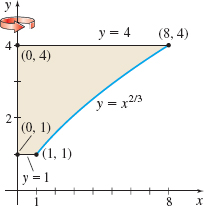
y=secx about the x-axis
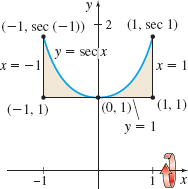
2π(tan1−1)
y=x2 about the y-axis
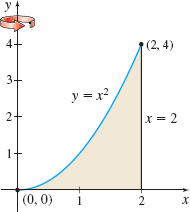
In Problems 11–16, use the disk method to find the volume of the solid of revolution generated by revolving the region bounded by the graphs of the given equations about the indicated axis.
y=2x2, the x-axis, x=1; about the x-axis
4π5
y=√x, the x-axis, x=4,x=9; about the x-axis
y=e−x, the x-axis, x=0,x=2; about the x-axis
12π(1−1e4)
y=ex, the x-axis, x=−1,x=1; about the x-axis
y=x2,x≥0,y=1,y=4; about the y-axis
15π2
y=2√x, the y-axis, y=4; about the y-axis
In Problems 17–22, use the washer method to find the volume of the solid of revolution generated by revolving the region bounded by the graphs of the given equations about the indicated axis.
y=x2,x≥0, the y-axis, y=4; about the x-axis
128π5
y=2x2,x≥0, the y-axis, y=2; about the x-axis
y=2√x, the y-axis, y=4; about the x-axis
32π
y=x2/3, the x-axis, x=8; about the y-axis
y=x3, the x-axis, x=2; about the y-axis
64π5
y=2x4, the x-axis, x=1; about the y-axis
In Problems 23–38, find the volume of the solid of revolution generated by revolving the region bounded by the graphs of the given equations about the indicated axis.
y=1x, the x-axis, x=1, x=2; about the x-axis
π2
y=1x, the x-axis, x=1, x=2; about the y-axis
y=√x, the y-axis, y=9; about the y-axis
59,049π5
y=√x, the y-axis, y=9; about the x-axis
y=(x−2)3, the x-axis, x=0,x=3; about the x-axis
129π7
y=(x−2)3, the x-axis, x=0,x=3; about the y-axis
y=(x+1)2,x≥0,y=16; about the y-axis
117π2
y=(x+1)2,x≤0,y=16; about the x-axis
x=y4−1, the y-axis; about the y-axis
64π45
y=x4−1, the x-axis; about the x-axis
y=4x, y=x3,x≥0; about the x-axis
512π21
y=2x+1,y=x,x=0,x=3; about the x-axis
y=1−x,y=ex,x=1; about the x-axis
(e22−56)π
y=cosx,y=sinx,x=0,x=π4; about the x-axis
y=cscx,y=0,x=π2,x=3π4; about the x-axis
π
y=secx,y=0,x=0,x=π3; about the x-axis
In Problems 39–46, find the volume of the solid of revolution generated by revolving the region bounded by the graphs of the given equations about the indicated line.
y=ex,y=0,x=0,x=2; about y=−1
(e42+2e2−52)π
y=1x,y=0,x=1,x=4; about y=4
y=x2, the x-axis, x=1; about x=1
π6
y=x3,x=0,y=1; about x=−1
y=√x, the x-axis, x=4; about x=−4
1024π15
y=1√x, the x-axis, x=1,x=4; about x=4
y=1x2,y=0,x=1,x=4; about y=4
363π64
y=√x,y=0,0≤x≤4; about y=−4
Applications and Extensions
Volume of a Solid of Revolution A region in the first quadrant is bounded by the x-axis and the graph of y=kx−x2, where k>0.
- (a) In terms of k, find the volume generated when the region is revolved around the x-axis.
- (b) In terms of k, find the volume generated when the region is revolved around the y-axis.
- (c) Find the number k for which the volumes found in parts (a) and (b) are equal.
- (a) k5π30
- (b) k4π6
- (c) k=5.
Volume of a Solid of Revolution
- (a) Find all numbers b for which the graphs of y=2x+b and y2=4x intersect in two distinct points.
- (b) If b=−4, find the area enclosed by the graphs of y=2x−4 and y2=4x.
424
- (c) If b=0, find the volume of the solid generated by revolving about the x-axis the region bounded by the graphs of y=2x and y2=4x.
Volume of a Solid of Revolution Find the volume of the solid of revolution generated by revolving the region bounded by the graphs of y=cosx and y=0 from x=0 to x=π2 about the line y=1. [Hint: cos2x=1+cos(2x)2.]
2π−π24
Volume of a Solid of Revolution Find the volume of the solid of revolution generated by revolving the region bounded by the graphs of y=cosx and y=0 from x=0 to x=π2 about the line y=−1. (See the hint in Problem 49.)
Challenge Problems
Volume of a Solid of Revolution The graph of the function P(x)=kx2 is symmetric with respect to the y-axis and contains the points (0,0) and (b,e−b2), where b>0.
- (a) Find k and write an equation for P=P(x).
- (b) The region bounded by P, the y-axis, and the line y=e−b2 is revolved about the y-axis to form a solid. Find its volume.
- (c) For what number b is the volume of the solid in (b) a maximum? Justify your answer.
- (a) k=e−b2b2, P(x)=e−b2b2x2
- (b) V=b2e−b2π2
- (c) b=1; answers will vary.
Volume of a Solid of Revolution Find the volume of the solid generated by revolving the region bounded by the catenary y=a cosh(xa)+b−a, the x-axis, x=0, and x=1 about the x-axis, where a>0 and b≥0. [Hint:cosh2 x=cosh(2x)+12.]
