6.3 Assess Your UnderstandingPrinted Page 431
Concepts and Vocabulary
True or False In using the shell method to find the volume of a solid revolved about the x-axis, the integration occurs with respect to x.
False
True or False The volume of a cylindrical shell of outer radius R, inner radius r, and height h is given by V=πr2h.
False
True or False The volume of a solid of revolution can be found using either washers or cylindrical shells only if the region is revolved about the y-axis.
False
True or False If y=f(x) is a function that is continuous and nonnegative on the closed interval [a,b],a≥0, then the volume V of the solid generated by revolving the region bounded by the graph of f and the x-axis from x=a to x=b about the y-axis is V=2π∫baxf(x) dx.
True
Skill Building
In Problems 5–16, use the shell method to find the volume of the solid of revolution generated by revolving the region bounded by the graphs of the given equations about the indicated axis.
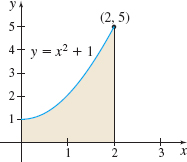
y=x2+1, the x-axis, 0≤x≤1; about the y-axis
3π2
y=x3,y=x2; about the y-axis
y=√x,y=x2; about the y-axis
3π10
y=1x, the x-axis, x=1,x=4; about the y-axis
y=x3, the y-axis, y=8; about the x-axis
768π7
y=√x, the y-axis, y=2; about the x-axis
x=√y, the y-axis, y=1; about the x-axis
4π5
x=4√y, the y-axis, y=4; about the x-axis
y=x,y=x2; about the x-axis
2π15
y=x,y=x3; in the first quadrant; about the x-axis
y=e−x2, and the x-axis, from x=0 to x=2; about the y-axis
(e4−1e4)π
y=x3+x and the x-axis, 0≤x≤1; about the y-axis
In Problems 17–22, use the shell method to find the volume of the solid of revolution generated by revolving the region bounded by the graphs of the given equations about the indicated line.
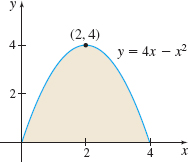
y=x2,y=4x−x2; about x=4
16π
y=x2,y=4x−x2; about x=3
y=x2,y=0,x=1,x=2; about x=1
17π6
y=x2,y=0,x=1,x=2; about x=−2
x=y−y2, the y-axis; about y=−1
π2
x=y−y2, the y-axis; about y=1
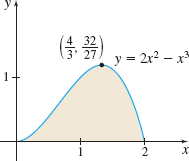
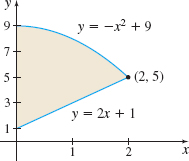
In Problems 23–26, use either the shell method or the disk/washer method to find the volume of the solid of revolution generated by revolving the shaded region in each graph:
- (a) about the x-axis.
- (b) about the y-axis.
- (c) Explain why you chose the method you used.
- (a) 128π105
- (b) 16π5
- (c) Answers will vary.
432
- (a) 206π15
- (b) 12π
- (c) Answers will vary.
In Problems 27–38, use either the shell method or the disk/washer method to find the volume of the solid of revolution generated by revolving the region bounded by the graphs of the given equations about the indicated axis.
y=3√x, the y-axis, y=2; about the x-axis
64π5
y=√x+x, the x-axis, x=1,x=4; about the y-axis
y=x3 and y=x to the right of x=0; about the y-axis
4π15
y=x3,y=x2; about the x-axis
y=3x2 and y=30−x to the right of x=1; about the y-axis
308π3
y=3x2 and y=30−x to the right of x=1; about the x-axis
y=x2 and y=8−x2 to the right of x=1; about the x-axis
80π3
y=x2 and y=8−x2 to the right of x=1; about the y-axis
y=x2,y=x; about the y-axis
π6
y=x2/3+x1/3, the x-axis, x=1,x=8; about the y-axis
y=√x and y=18−x2 to the right of x=1; about the y-axis
1177π10
y=√x and y=18−x2 to the right of x=1; about the x-axis
Applications and Extensions
Volume of a Solid of Revolution Find the volume of the solid of revolution generated by revolving the region bounded by the graph of y=1(x2+1)2 and the x-axis from x=0 to x=1 about the y-axis as shown in the figure.
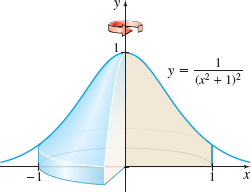
π2
Volume of a Solid of Revolution Find the volume of the solid of revolution generated by revolving the region bounded by the graph of y=√x+1+x and the x-axis from x=0 to x=3 about the y-axis.
Volume of a Solid of Revolution Find the volume of the solid of revolution generated by revolving the region in the first quadrant bounded by the x-axis and the graph of y=2x−x2:
- (a) about the x-axis.
- (b) about the y-axis.
- (c) about the line x=3.
- (d) about the line y=1.
- (a) 1615π
- (b) 8π3
- (c) 16π3
- (d) 85π
 Volume of a Solid of Revolution Find the volume of the solid of revolution generated by revolving the region in the first quadrant bounded by the x-axis and the graph of y=x√9−x.
Volume of a Solid of Revolution Find the volume of the solid of revolution generated by revolving the region in the first quadrant bounded by the x-axis and the graph of y=x√9−x.- (a) about the x-axis.
- (b) about the y-axis.
- (c) about the line y=−3.
- (d) about the line x=−2.
Volume of a Solid of Revolution Suppose f(x)≥0 for x≥0, and the region bounded by the graph of f and the x-axis from x=0 to x=k,k>0, is revolved about the x-axis. If the volume of the resulting solid is 15k5+k4+43k3, find f.
f(x)=x2+2x√π
Volume of a Solid of Revolution Find the volume of the solid generated by revolving about the y-axis the region bounded by the graph of y=e−x2 and the y-axis from x=0 to the positive x-coordinate of the point of inflection of y=e−x2.
 Volume of a Solid of Revolution Show that the volume V of the solid generated by revolving the region bounded by the graph of y=sin3x and the x-axis from x=0 to x=π about the line x=−π is given by V=4π2.
Volume of a Solid of Revolution Show that the volume V of the solid generated by revolving the region bounded by the graph of y=sin3x and the x-axis from x=0 to x=π about the line x=−π is given by V=4π2.
See Student Solutions Manual.
Challenge Problems
Comparing Volume Formulas In the figure below, the region bounded by the graphs of y=g(x),y=f(x), and the y-axis is to be revolved about the y-axis. Show that the resulting volume V is given by:
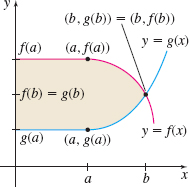
- (a) V=πa2[f(a)−g(a)]+2π∫bax[f(x)−g(x)]dx if the shell method is used.
- (b) V=π∫g(b)g(a)[g−1(y)]2dy+π∫f(a)g(b)[f−1(y)]2dy if the disk method is used
Suppose a plane region of area A that lies to the right of the y-axis is revolved about the y-axis, generating a solid of volume V. If this same region is revolved about the line x=−k,k>0, show that the solid generated has volume V+2πkA.
See Student Solutions Manual.
Find the volume generated by revolving the region bounded by the parabola y2=8x and its latus rectum about the latus rectum:
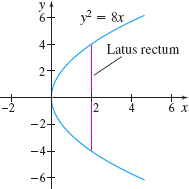
- (a) by using the disk method.
- (b) by using the shell method.
(The latus rectum is the chord through the focus perpendicular to the axis of the parabola.) See the figure.