7.6 Assess Your UnderstandingPrinted Page 518
518
Concepts and Vocabulary
True or False The Trapezoidal Rule approximates an integral ∫baf(x)dx by replacing the graph of f with line segments.
True
True or False Simpson’s Rule approximates an integral by using parabolic arcs.
True
Skill Building
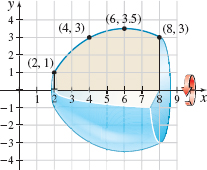
For Problems 3 and 5, use the graph below to approximate the area A. Round answers to three decimal places.
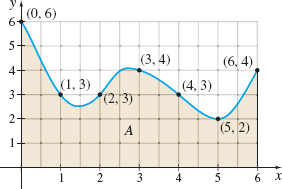
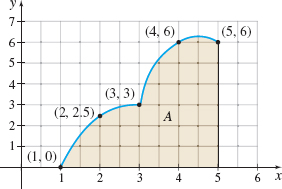
For Problems 4 and 6, use the graph below to approximate the area A. Round answers to three decimal places.
Use the Trapezoidal Rule with n = 3 and n = 6 to approximate the area under the graph.
22 and 20
Use the Trapezoidal Rule with n = 2 and n = 4 to approximate the area under the graph.
Use Simpson’s Rule with n = 2 and n = 6 to approximate the area under the graph.
26 and 583
Use Simpson’s Rule with n = 2 and n = 4 to approximate the area under the graph.
In Problems 7—12:
- (a) Use the Trapezoidal Rule to approximate each integral.
- (b)
 Estimate the error in using the approximation. Express the answer rounded to three decimal places.
Estimate the error in using the approximation. Express the answer rounded to three decimal places. - (c) Find the number n of subintervals needed to guarantee that an approximation is correct to within 0.0001.
∫ππ/2sinxxdx; n = 3
- (a) 0.483
- (b) 0.007
- (c) 26
∫2π3π/2cosxxdx; n = 3
∫10e−x2dx; n = 4
- (a) 0.743
- (b) 196
- (c) 41
∫10ex2dx; n = 4
∫0−1dx√1−x3; n = 4
- (a) 0.907
- (b) 1192
- (c) 29
∫10dx√1+x3; n = 3
In Problems 13—18:
- (a) Use Simpson’s Rule to approximate each integral.
- (b)
 Estimate the error in using the approximation. Express the answer rounded to three decimal places.
Estimate the error in using the approximation. Express the answer rounded to three decimal places. - (c) Find the number n of subintervals needed to guarantee that an approximation is correct to within 0.0001.
∫21exxdx; n = 4
- (a) 3.059
- (b) 5.309×10−4
- (c) 8
∫2π3π/2cosxxdx; n = 4
∫10e−x2dx; n = 4
- (a) 0.747
- (b) 2.604×10−4
- (c) 6
∫10ex2dx; n = 4
∫0−1dx√1−x3; n = 4
- (a) 0.910
- (b) 3.255×10−4
- (c) 6
∫10dx√1+x2; n = 4
Applications and Extensions
- (a) Show that ∫21dxx=ln2.
- (b) Use the Trapezoidal Rule with n=5 to approximate ∫21dxx.
- (c) Use Simpson’s Rule with n=6 to approximate ∫21dxx.
- (a) See the Student Solutions Manual.
- (b) 0.6956
- (c) 0.6932
Area Selected measurements of a function f are given in the table below. Use Simpson’s Rule to approximate the area enclosed by the graph of f, the x-axis, and the lines x=2 and x=4.4.
x 2.0 2.4 2.8 3.2 3.6 4.0 4.4 y 3.03 4.61 5.80 6.59 7.76 8.46 9.19
Arc Length Approximate the arc length of the graph of y=sinx from x=0 to x=π2
- (a) using Simpson’s Rule with n=4.
- (b) using the Trapezoidal Rule with n=3.
- (a) 1.910
- (b) 1.910
Arc Length Approximate the arc length of the graph of y=ex from x=0 to x=4
- (a) using Simpson’s Rule with n=4.
- (b) using the Trapezoidal Rule with n=8.
Work A gas expands from a volume of 1 cubic inch (in.3) to 2.5 in.3; values of the volume V and pressure p (in pounds per square inch) during the expansion are given in the table below. Find the total work W done in the expansion using Simpson’s Rule. (Hint: W=∫bapdV).
V 1 1.25 1.5 1.75 2 2.25 2.5 p 68.7 55.0 45.8 39.3 34.4 30.5 27.5
≈62.983 in lb
Work In the table below, F is the force in pounds acting on an object in its direction of motion and x is the displacement of the object in feet. Use the Trapezoidal Rule to approximate the work done by the force in moving the object from x=0 to x=50.
x 0 5 10 15 20 25 30 35 40 45 50 F 100 80 66 56 50 45 40 36 33 30 28
519
Volume In the table below, S is the area in square meters of the cross section of a railroad track cutting through a mountain, and x meters is the corresponding distance along the line. Use the Trapezoidal Rule to find the number of cubic meters of earth removed to make the cutting from x=0 to x=150. See the figure below.
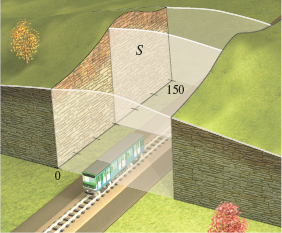
x 0 25 50 75 100 125 150 S 105 118 142 120 110 90 78
16,787.5 m3
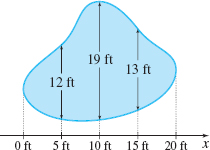
Area Use Simpson’s Rule to approximate the surface area of the pond pictured in the figure.
Volume The area of the horizontal section of a reservoir is A square meters at a height x meters from the bottom. Corresponding values of A and x are given in the table below. Approximate the volume of water in the reservoir using the Trapezoidal Rule and also using Simpson’s Rule. See the figure.
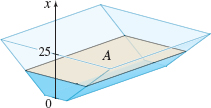
x 0 2.5 5 7.5 10 12.5 15 17.5 20 22.5 25 A 0 2510 3860 4870 5160 5590 5810 6210 6890 7680 8270
T: 131,787.5 m3, S: 132,625 m3
Area A series of soundings taken across a river channel is given in the table below, where x meters is the distance from one shore and y meters is the corresponding depth of the water. Find its area by the Trapezoidal Rule.
x 0 10 20 30 40 50 60 70 80 y 5 10 13.2 15 15.6 12 6 4 0
Volume of a Solid of Revolution Use the Trapezoidal Rule with n=3 to approximate the volume of the solid of revolution formed by revolving the region shown in the figure below about the x-axis.
1052π≈164.934
Distance Traveled The speed v, in meters per second, of an object at time t is given in the table below.
t 0 0.5 1 1.5 2 2.5 3 v 5.1 5.3 5.6 6.1 6.8 6.7 6.5 - (a) Use the Trapezoidal Rule to approximate the distance s traveled by the object from t=0 to t=3.
- (b) Use Simpson’s Rule to approximate the distance s traveled by the object from t=0 to t=3.
Volume of a Solid of Revolution Approximate the volume of the solid of revolution in the figure below generated by revolving the region bounded by the graph of y=sinx and the y-axis from x=0 to x=π2 about the y-axis
- (a) using Simpson’s Rule with n=4.
- (b) using the Trapezoidal Rule with n=3.
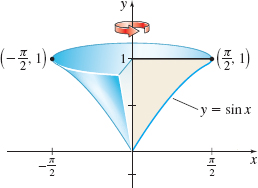
- (a) Using the disk method: V≈1.6095, Using the shell method: V≈1.4709
- (b) Using the disk method: V≈1.9705, Using the shell method: V≈1.3228
Arc Length Use the Trapezoidal Rule to find the arc length of the ellipse 9x2+100y2=900 in the first quadrant from x=0 to x=8. Partition the interval into four equal subintervals, and round the answer to three decimal places.
Approximate ∫π0f(x)dx if f(x)={sinxxifx≠01ifx=0
- (a) Use the Trapezoidal Rule with n=6.
- (b) Use Simpson’s Rule with n=6.
- (a) 1.845
- (b) 1.852
 Approximate ∫1−15e−x2dx.
Approximate ∫1−15e−x2dx.- (a) Use the Trapezoidal Rule with n=20 subintervals.
- (b) Use Simpson’s Rule with n=20 subintervals.
- (c) Use a CAS to find the integral.
520
Challenge Problems
Let Tn be the approximation to ∫baf(x)dx given by the Trapezoidal Rule with n subintervals. Without using the error formula given in the text, show that lim.
See the Student Solutions Manual.
Show that if f(x)=Ax^{3}+Bx^{2}+Cx+D, then Simpson’s Rule gives the exact value of \int_{a}^{b}f(x)\,dx.
