exercises
In Exercises 1 to 8, sketch the given vector field or a small multiple of it.
Question 4.53
\({\bf F}(x,y)=(2,2)\)
Question 4.54
\({\bf F}(x,y)=(4,0)\)
Question 4.55
\({\bf F}(x,y)=(x,y)\)
Question 4.56
\({\bf F}(x,y)=(-x,y)\)
Question 4.57
\({\bf F}(x,y)=(2y,x)\)
Question 4.58
\({\bf F}(x,y)=(y,-2x)\)
Question 4.59
\({\bf F}(x,y)=\bigg(\displaystyle\frac{x}{\sqrt{x^2+y^2}},\displaystyle\frac{y}{\sqrt{x^2+y^2}}\bigg)\)
Question 4.60
\({\bf F}(x,y)=\bigg(\displaystyle\frac{y}{\sqrt{x^2+y^2}},\displaystyle\frac{x}{\sqrt{x^2+y^2}}\bigg)\)
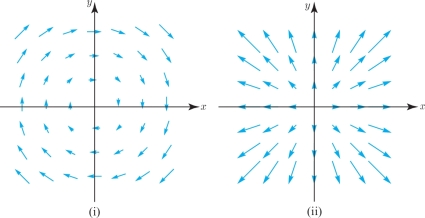
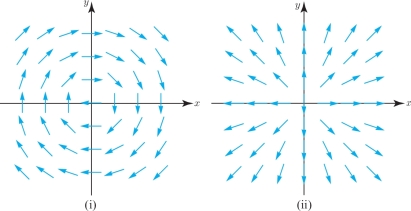
In the following two exercises, match the given vector field with its pictorial description (see Figure 4.29 and Figure 4.30).
Question 4.61
- (a) \(\textbf{V}(x,y) = x \textbf{i} + y \textbf{j}\)
- (b) \(\textbf{V}(x,y) = y \textbf{i} - x \textbf{j}\)
Question 4.62
- (a) \(\textbf{V}(x,y) = \displaystyle\frac{y}{\sqrt{x^2 + y^2}} \textbf{i} - \displaystyle\frac{x}{\sqrt{x^2 + y^2}} \textbf{j}\)
- (b) \(\textbf{V}(x,y) = \displaystyle\frac{x}{\sqrt{x^2 + y^2}} \textbf{i} + \displaystyle\frac{y}{\sqrt{x^2 + y^2}} \textbf{j}\)
Where are these vector fields not defined? How are these vector fields related to those in Problem 9?
In Exercises 11 to 14, sketch a few flow lines of the given vector field.
Question 4.63
\({\bf F}(x,y)=(y,-x)\)
Question 4.64
\({\bf F}(x,y)=(x,-y)\)
Question 4.65
\({\bf F}(x,y)=(x,x^2)\)
Question 4.66
\({\bf F}(x,y,z)=(y,-x,0)\)
244
In Exercises 15 to 18, show that the given curve \({\bf c}(t)\) is a flow line of the given velocity vector field \({\bf F}(x,y,z)\).
Question 4.67
\({\bf c}(t)=(e^{2t},\log |t|,1/t),t\neq 0; {\bf F}(x,y,z)=(2x,z,-z^2)\)
Question 4.68
\({\bf c}(t)=(t^2,2t-1,\sqrt{t}),t > 0 ;{\bf F}(x,y,z)=(y+1,2,1/2z)\)
Question 4.69
\({\bf c}(t)=(\sin t,\,\cos t,e^t);{\bf F}(x,y,z)=(y,-x,z)\)
Question 4.70
\({\bf c}(t)=(\displaystyle\frac{1}{t^3},e^t,\displaystyle\frac{1}{t});{\bf F}(x,y,z)=(-3z^4,y,-z^2)\)
Question 4.71
Let \(\textbf{F}(x,y,z)=(x^2,yx^2,z+zx)\) and \(\textbf{c}(t)= (\displaystyle\frac{1}{1-t},0,\displaystyle\frac{e^t}{1-t})\). Show \(\textbf{c}(t)\) is a flow line for \(\textbf{F}\).
Question 4.72
Show that \(\textbf{c}(t)=(a\cos t-b \sin t, a \sin t +b\cos t)\) is a flow line for \(\textbf{F}(x, y)=(-y, x)\) for all real values of \(a\) and \(b\).
Question 4.73
- (a) Let \(\textbf{F}(x, y, z)= (yz, xz, xy)\). Find a function \(f \colon \mathbb R^3 \to \mathbb R\) such that \(\textbf{F}=\nabla f\).
- (b) Let \(\textbf{F}(x, y, z)= (x, y, z)\). Find a function \(f \colon \mathbb R^3 \to \mathbb R\) such that \(\textbf{F}=\nabla f\).
Question 4.74
Let \(f(x, y)=x^2+y^2\). Sketch the gradient vector field \(\nabla f\) together with some level sets of \(f\). How are they related?
Question 4.75
Show that it takes half as much energy to launch a satellite into an orbit just above the earth as it does to escape the earth. (Ignore the rotation of the earth.)
Question 4.76
Let \({\bf c}(t)\) be a flow line of a gradient field \({\bf F}=-{\nabla} V\). Prove that \(V({\bf c}(t))\) is a decreasing function of \(t\).
Question 4.77
Suppose that the isotherms in a region are all concentric spheres centered at the origin. Prove that the energy flux vector field points either toward or away from the origin.
Question 4.78
Sketch the gradient field \(-{ \nabla} V\) for \(V(x,y)=(x+y)/(x^2+y^2)\) and the equipotential surface \(V=1\).
Question 4.79
Let \(\textbf{F}(x, y, z)= (xe^y, y^2z^2, xyz)\) and suppose \(\textbf{c}(t)=\big(x(t), y(t), z(t)\big)\) is a flow line for \(\textbf{F}\). Find the system of differential equations that the functions \(x(t), y(t),\) and \(z(t)\) must satisfy.