5.6 The Triple Integral
Triple integrals are needed for many physical problems. For example, if the temperature inside an oven is not uniform, determining the average temperature involves “summing” the values of the temperature function at all points in the solid region enclosed by the oven walls and then dividing the answer by the total volume of the oven. Such a sum is expressed mathematically as a triple integral.
Definition of the Triple Integral
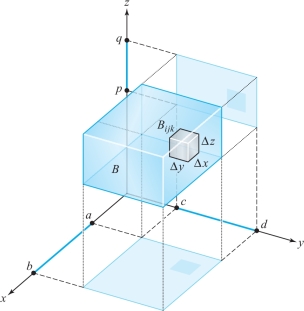
Our objective now is to define the triple integral of a function \(f(x, y, z)\) over a box (rectangular parallelepiped) \(B = [a, b]\) \(\times\) \([c, d]\) \(\times\) \([p, q]\). Proceeding as in double integrals, we partition the three sides of \(B\) into \(n\) equal parts and form the sum \[ S_n = \sum_{i = 0}^{n-1}\sum_{j=0}^{n-1}\sum_{k=0}^{n-1} f({\bf c}_{\it ijk})\, \Delta V, \] where \({\bf c}_{\it ijk}\) is a point in \(B_{\it ijk}\), the \(ijk\)th rectangular parallelepiped (or box) in the partition of \(B\), and \(\Delta V\) is the volume of \(B_{\it ijk}\) (see Figure 5.30).
295
Definition: Triple Integrals
Let \(f\) be a bounded function of three variables defined on \(B\). If \({\mathop{\rm limit}_{n\to \infty}}\, S_n = S\) exists and is independent of any choice of \({\bf c}_{\it ijk}\), we call \(f\) integrable and call \(S\) the triple integral (or simply the integral) of \(f\) over \(B\) and denote it by \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{B}\, f\ {\it dV}, \intop\!\!\!\intop\!\!\!\intop\nolimits_{B}\, f(x, y,z)\, {\it {\,d} V} \qquad\hbox{or} \intop\!\!\!\intop\!\!\!\intop\nolimits_{B}\, f(x, y, z)\,{\it dx}\, {\it dy}\, {\it dz}. \]
Properties of Triple Integrals
As before, we can prove that continuous functions defined on \(B\) are integrable. Moreover, bounded functions whose discontinuities are confined to graphs of continuous functions [such as \(x = \alpha(y,z), y = \beta(x, z)\), or \(z = \gamma(x, y)\)] are integrable. The other basic properties (such as the fact that the integral of a sum is the sum of the integrals) for double integrals also hold for triple integrals. Especially important is the reduction to iterated integrals:
Reduction to Iterated Integrals
Let \(f(x, y, z)\) be integrable on the box \(B = [a, b] \times [c, d] \times [p, q]\). Then any iterated integral that exists is equal to the triple integral; that is, \begin{eqnarray*} \intop\!\!\!\intop\!\!\!\intop\nolimits_{B} f(x, y, z)\,{\it dx}\,{\it dy}\,{\it dz} &=& \int_p^q\int_c^d\int_a^b f(x,y, z)\,{\it dx}\,{\it dy}\,{\it dz}\\[2pt] &=& \int_p^q\int_a^b\int_c^d f(x,y, z)\,{\it dy}\,{\it dx}\,{\it dz}\\[2pt] &=& \int_a^b\int_p^q\int_c^d f(x,y, z)\,{\it dy}\,{\it dz}\,{\it dx}, \end{eqnarray*} and so on. (There are six possible orders altogether.)
296
example 1
- (a) Let \(B\) be the box \([0, 1] \times \big[{-}\frac{1}{2}, 0\big] \times \big[0, \frac{1}{3}\big]\). Evaluate \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{B}(x+2y+3z)^2{\it dx}\,{\it dy}\,{\it dz}. \]
- (b) Verify that we get the same answer if the integration is done in the order \(y\) first, then \(z\), and then \(x\).
solution
- (a) According to the principle of reduction to iterated integrals, this integral may be evaluated as \begin{eqnarray*} &&\int_0^{1/3}\int_{-1/2}^0\int_0^1 (x+2y+3z)^2 {\it dx}\,{\it dy}\,{\it dz}\\[8pt] &&\quad = \int_0^{1/3}\int_{-1/2}^0\Bigg[\frac{(x+2y+3z)^3}{3} \bigg|_{x=0}^1\Bigg]{\it dy}\,{\it dz}\\[8pt] &&\quad = \int_0^{1/3}\int_{-1/2}^0\frac{1}{3}\Big[(1+2y+3z)^3 - (2y+3z)^3\Big]{\it dy}\,{\it dz}\\ [8pt] &&\quad = \int_0^{1/3}\frac{1}{24}\Big[(1+2y +3z)^4 - (2y + 3z)^4\Big]\Big|_{y=-1/2}^0\, dz\\[8pt] &&\quad = \int_0^{1/3}\frac{1}{24}\Big[(3z+1)^4 - 2(3z)^4 + (3z-1)^4\Big] {\it dz}\\[8pt] &&\quad = \frac{1}{24\,{\cdot}\, 15}\Big[(3z+1)^5 - 2(3z)^5 + (3z-1)^5\Big]\Big|_{z=0}^{1/3}\\ [8pt] &&\quad = \frac{1}{24\,{\cdot}\, 15}(2^5-2) = \frac{1}{12}.\\[-11pt] \end{eqnarray*}
- (b) \begin{eqnarray*} &&\intop\!\!\!\intop\!\!\!\intop\nolimits_{B} (x+2y+3z)^2 {\it dy}\,{\it dz}\,{\it dx}\\[8pt] &&\quad = \int_0^1 \int_0^{1/3}\int_{-1/2}^0(x+2y+3z)^2{\it dy}\,{\it dz}\,{\it dx}\\[8pt] &&\quad = \int_0^1 \int_0^{1/3} \Bigg[\frac{(x+2y+3z)^3}{6} \bigg|_{y = -1/2}^0\Bigg]{\it dz}\,{\it dx}\\[8pt] &&\quad = \int_0^1 \int_0^{1/3}\frac{1}{6}\Big[(x+3z)^3 - (x+3z-1)^3\Big]{\it dz}\,{\it dx}\\[8pt] &&\quad = \int_0^1\frac{1}{6}\Bigg\{\Bigg[\frac{(x+3z)^4}{12} - \frac{(x+3z-1)^4}{12} \Bigg]\bigg|_{z=0}^{1/3}\Bigg\}{\it dx}\\[8pt] &&\quad = \int_0^1\frac{1}{72}\Big[(x+1)^4 + (x-1)^4 - 2x^4\Big]{\it dx}\\[8pt] &&\quad = \frac{1}{72} \frac{1}{5}\Big[(x+1)^5 + (x-1)^5 - 2x^5\Big]_{x=0}^1 = \frac{1}{12}.\\[-30pt] \end{eqnarray*}
297
example 2
Integrate \(e^{x+y+z}\) over the box \([0, 1]\times [0, 1]\times[0, 1]\).
solution We perform the integrations in the standard order: \begin{eqnarray*} &&\int_0^1\int_0^1\int_0^1 e^{x+y+z}\,{\it dx}\,{\it dy}\,dz = \int_0^1\int_0^1 (e^{x+y+z}|_{x=0}^1)\,{\it dy}\,{\it dz}\\[6pt] &&\quad = \int_0^1\int_0^1(e^{1+y+z} - e^{y+z})\,{\it dy}\,dz = \int_0^1 \Big[e^{1+y+z} - e^{y+z}\Big]_{y=0}^1{\it dz}\\[6pt] &&\quad = \int_0^1\Big[e^{2+z}-2e^{1+z} + e^z\Big]\,dz = \Big[e^{2+z} - 2e^{1+z} + e^z\Big]_0^1\\[6pt] &&\quad = e^3 - 3e^2 + 3e -1 = (e-1)^3.\\[-36pt] \end{eqnarray*}
As in the two-variable case, we define the integral of a function \(f\) over a bounded region \(W\) by defining a new function \(f^*\), equal to \(f\) on \(W\) and zero outside \(W\), and then setting \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{W} f(x, y, z)\,{\it dx}\,{\it dy}\,{\it dz} = \intop\!\!\!\intop\!\!\!\intop\nolimits_{B} f^*(x, y, z)\,{\it dx}\,{\it dy}\,{\it dz}, \] where \(B\) is any box containing the region \(W\).
Elementary Regions
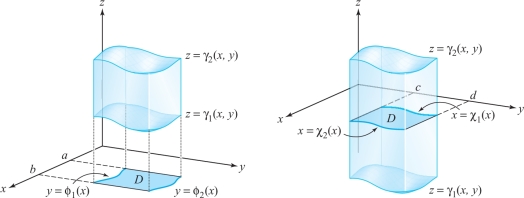
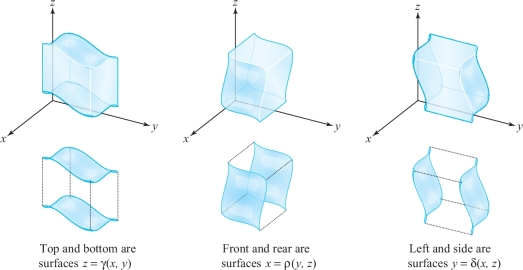
As before, we restrict our attention to particularly simple regions. An elementary region in three-dimensional space is one defined by restricting one of the variables to be between two functions of the remaining variables, the domains of these functions being an elementary (i.e., an \(x\)-simple or a \(y\)-simple) region in the plane. For example, if \(D\) is an elementary region in the \(xy\) plane and if \(\gamma_1(x, y)\) and \(\gamma_2(x, y)\) are two functions with \(\gamma_2(x,y)\ge\gamma_1(x, y)\), an elementary region consists of all \((x, y, z)\) such that \((x, y)\) lies in \(D\) and \(\gamma_1(x, y)\le z\leq \gamma_2(x, y)\). Figure 5.31 shows two elementary regions.
example 3
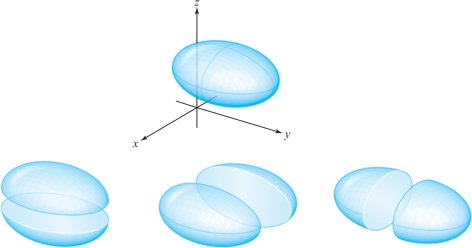
Describe the unit ball \(x^2 + y^2 +z^2 \le 1\) as an elementary region.
solution This can be done in several ways. One, in which \(D\) is \(y\)-simple, is: \begin{eqnarray*} &-1\le x \le 1, &\\[3pt] &-\sqrt{1-x^2} \le y \le \sqrt{1 - x^2}, &\\[3pt] & -\sqrt{1 - x^2-y^2} \le z \le \sqrt{1 - x^2-y^2}.&\\[-30pt] \end{eqnarray*}
298
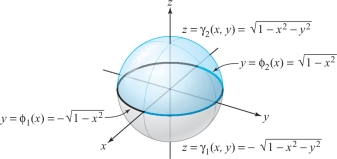
In doing this, we first write the top and bottom hemispheres as \(z = \sqrt{1 - x^2 - y^2}\) and \(z = -\sqrt{1 - x^2 -y^2}\), respectively, where \(x\) and \(y\) vary over the unit disk (that is, \(- \sqrt{1 - x^2}\le y\le \sqrt{1 - x^2}\) and \(x\) varies between \(-1\) and 1). (See Figure 5.32.) We can describe the region in other ways by interchanging the roles of \(x, y\), and \(z\) in the defining inequalities.
Integrals over Elementary Regions
As with integrals in the plane, any function of three variables that is continuous over an elementary region is integrable on that region. An argument like that for double integrals shows that a triple integral over an elementary region can be rewritten as an iterated integral in which the limits of integration are functions. The formulas for such iterated integrals are given in the following box.
Triple Integrals by Iterated Integration
Suppose that \(W\) is an elementary region described by bounding \(z\) between two functions of \(x\) and \(y\). Then either \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{W} f(x, y, z)\, {\it dx}\,{\it dy}\,{\it dz} = \int_a^b \int_{\phi_1(x)}^{\phi_2(x)} \int_{\gamma_1(x, y)}^{\gamma_2(x, y)}f(x,y,z)\, {\it dz}\,{\it dy}\,{\it dx} \] [see Figure 5.31] or \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{W} f(x, y, z)\, {\it dx}\,{\it dy}\,{\it dz} = \int_c^d \int_{\psi_1(y)}^{\psi_2(y)} \int_{\gamma_1(x, y)}^{\gamma_2(x, y)}f(x,y,z)\, {\it dz}\,{\it dx}\,{\it dy} \] [see Figure 5.31].
If \(f=1\), we get the integral \({\intop\!\!\!\intop\!\!\!\intop}_W {\it dx}\,{\it dy}\,{\it dz}\), which is the volume of the region \(W\).
example 4
Verify that the volume formula for the ball of radius 1: \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{W} {\it dx}\,{\it dy}\,{\it dz} = \frac{4}{3}\pi, \] where \(W\) is the set of \((x, y, z)\) with \(x^2 + y^2 + z^2 \le 1\).
299
solution We use the description of the unit ball from Example 3. From the first formula in the preceding box, the integral is \[ \int_{-1}^1 \int_{-\sqrt{1-x^2}}^{\sqrt{1-x^2}} \int^{\sqrt{1-x^2-y^2}}_{-\sqrt{1-x^2-y^2}} {\it dz}\,{\it dy}\,{\it dx}. \]
Holding \(y\) and \(x\) fixed and integrating with respect to \(z\) yields \[ \int_{-1}^1 \int_{-\sqrt{1-x^2}}^{\sqrt{1-x^2}} \bigg[z \Big|^{\sqrt{1-x^2-y^2}}_{-\sqrt{1-x^2-y^2}}\bigg]\, {\it dy}\, {\it dx} = 2 \int_{-1}^1\Bigg[\int_{-\sqrt{1-x^2}}^{\sqrt{1-x^2}}(1-x^2-y^2)^{1/2}\, {\it dy} \Bigg]{\it dx}. \]
Because \(x\) is fixed in the \(y\)-integral, it can be expressed as \(\int_{-a}^a(a^2 - y^2)^{1/2}\,{\it dy}\), where \(a = (1- x^2)^{1/2}\). This integral is the area of a semicircular region of radius \(a\), so that \[ \int_{-a}^a(a^2 - y^2)^{1/2}\,{\it dy} = \frac{a^2}{2}\pi. \] (We could also have used a trigonometric substitution or a table of integrals.) Thus, \[ \int_{-\sqrt{1-x^2}}^{\sqrt{1-x^2}} (1-x^2 - y^2)^{1/2}{\it dy} = \frac{1-x^2}{2}\pi, \] and so \begin{eqnarray*} && 2\int_{-1}^1\int_{-\sqrt{1-x^2}}^{\sqrt{1-x^2}}(1 - x^2 - y^2)^{1/2} {\it dy}\,{\it dx} = 2\int_{-1}^1\pi\frac{1-x^2}{2}\,{\it dx}\\[2pt] &&\quad = \pi \int_{-1}^1(1-x^2)\,{\it dx} = \pi\bigg(x - \frac{x^3}{3}\bigg) \bigg|_{x = -1}^1 = \frac{4}{3}\pi.\\[-30pt] \end{eqnarray*}
Other types of elementary regions are shown in Figure 5.33. For instance, in the second region, \((y,z)\) lies in an elementary region in the \(yz\) plane and \(x\) lies between two graphs: \[ \rho_1(y, z) \le x \le \rho_2(y, z). \]
300
As shown in Figure 5.34, some elementary regions can be simultaneously described in all three ways. We shall call these regions symmetric elementary regions.
Corresponding to each description of a region as an elementary region is an integration formula. For instance, if \(W\) is expressed as the set of all \((x, y, z)\) such that \[ c \le y \le d, \psi_1(y)\le z\le \psi_2(y), \rho_1(y, z)\le x\le \rho_2(y, z), \] then \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{W} f(x, y, z)\, {\it dx}\,{\it dy}\,dz = \int_c^d\int_{\psi_1(y)}^{\psi_2(y)} \int_{\rho_1(y,z)}^{\rho_2(y, z)}f(x, y, z)\,{\it dx}\,{\it dz}\,{\it dy}. \]
example 5
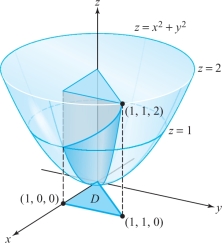
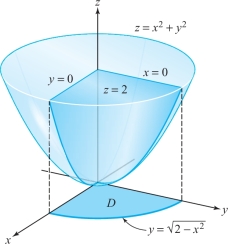
Let \(W\) be the region bounded by the planes \(x=0, y=0\), and \(z=2\), and the surface \(z = x^2 + y^2\) and lying in the quadrant \(x \ge 0, y \ge 0\). Compute \(\displaystyle\intop\!\!\!\intop\!\!\!\intop\nolimits_Wx\,{\it dx}\,{\it dy}\,{\it dz}\) and sketch the region.
solution Method 1. The region \(W\) is sketched in Figure 5.35. As indicated in the figure, we can describe this region by the inequalities \[ 0 \le x\le \sqrt{2}, 0 \le y \le \sqrt{2 - x^2}, x^2+y^2 \le z\le 2. \]
301
Therefore, \begin{eqnarray*} \intop\!\!\!\intop\!\!\!\intop\nolimits_{W} x\, {\it dx}\,{\it dy}\,{\it dz} &=& \int_0^{\sqrt{2}}\Bigg[\int_0^{\sqrt{2-x^2}} \bigg(\int_{x^2+y^2}^2 x\, dz\bigg)\, {\it dy}\Bigg]\, {\it dx}\\[4pt] &=& \int_0^{\sqrt{2}}\int_0^{\sqrt{2 - x^2}}x(2-x^2-y^2)\,{\it dy}\,{\it dx}\\[4pt] &=& \int_0^{\sqrt{2}} x\bigg[(2-x^2)^{3/2} - \frac{(2-x^2)^{3/2}}{3}\bigg]{\it dx}\\ [4pt] &=& \int_0^{\sqrt{2}}\frac{2x}{3}(2-x^2)^{3/2}\,{\it dx} = \frac{-2(2 - x^2)^{5/2}}{15}\bigg|_0^{\sqrt{2}}\\[4pt] &=& 2\,{\cdot}\, \frac{2^{5/2}}{15} = \frac{8\sqrt{2}}{15}. \end{eqnarray*}
Method 2. We can also place limits on \(x\) first and describe \(W\) by \(0\leq x\leq (z-y^2)^{1/2}\) and \((y, z)\) in \(D\), where \(D\) is the subset of the \(yz\) plane with \(0\le z\le 2\) and \(0\le y\le z^{1/2}\) (see Figure 5.36).
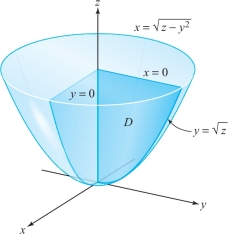
Therefore, \begin{eqnarray*} \intop\!\!\!\intop\!\!\!\intop\nolimits_{W}x\, {\it dx}\,{\it dy}\,{\it dz} &=& \intop\!\!\!\intop\nolimits_{D}\bigg(\int^{(z-y^2)^{1/2}}_{0}x\, {\it dx} \bigg)\,{\it dy}\, {\it dz}\\[6pt] &=&\int^2_0\bigg[\int^{z^{1/2}}_{0}\bigg(\int^{(z-y^2)^{1/2}}_{0}x {\it dx} \bigg)\,{\it dy}\bigg]\, {\it dz}\\[6pt] &=&\int^2_0\int^{z^{1/2}}_{0}\bigg(\frac{z-y^2}{2}\bigg)\, {\it dy}\,{\it dz}\\[6pt] &=&\frac{1}{2}\int^2_0\bigg( z^{3/2}-\frac{z^{3/2}}{3}\bigg)\, {\it dz} = \frac{1}{2}\int^2_0\frac{2}{3} z^{3/2}\,{\it dz}\\[6pt] &=& \bigg[\frac{2}{15}z^{5/2}\bigg]^2_0=\frac{2}{15}2^{5/2}=\frac{8\sqrt{2}}{15}, \end{eqnarray*} which agrees with our previous answer.
302
example 6
Evaluate \[ \int_0^1\int_0^x\int_{x^2+y^2}^2{\it dz}\,{\it dy}\,{\it dx}. \]
Sketch the region \(W\) of integration and interpret.
solution
\begin{eqnarray*} \int_0^1\int_0^x\int_{x^2+y^2}^2 {\it dz}\,{\it dy}\,{\it dx} &=& \int_0^1\int_0^x(2-x^2-y^2)\,{\it dy}\,{\it dx}\\[6pt] &=& \int_0^1\bigg(2x-x^3 - \frac{x^3}{3}\bigg)\,{\it dx} = 1 -\frac{1}{4} -\frac{1}{12} = \frac{2}{3}. \end{eqnarray*}
This integral is the volume of the region sketched in Figure 5.37.