Question 6.81

\({\displaystyle\intop\!\!\!\intop\nolimits_{\! D}} \frac{\textstyle 1}{{\textstyle \sqrt{xy}}}\ {\it dA}\), where \(D=[0,1]\times [0,1]\)

Question 6.82

\({\displaystyle\intop\!\!\!\intop\nolimits_{\! D}} \frac{\textstyle1}{{\textstyle\sqrt{|x-y|}}}\,{\it dx}\,{\it dy}\), where \(D=\{(x,y)\mid 0\leq x\leq 1\), \(0\leq y\leq 1\), \(y\leq x\}\)

Question 6.83

\( {\displaystyle\intop\!\!\!\intop\nolimits_{\! D}}(y/x)\,{\it dx}\,{\it dy}\), where \(D\) is bounded by \(x=1,x=y\), and \(x=2y\)

Question 6.84

\({\displaystyle\int\nolimits^1_0}{\displaystyle\int\nolimits^{e^v}_0}\log x\,{\it dx}\,{\it dy}\)

Question 6.85

Let \(D = [0,1] \times [0,1]\). Let \(0 < \alpha < 1\) and \(0 < \beta < 1\). Evaluate: \[ \intop\!\!\!\intop\nolimits_{D} \frac{dx\, dy}{x^{\alpha}y^{\beta}}. \]

Question 6.86

Let \(D = [1, \infty) \times [1,\infty]\). Let \(1 < \gamma\) and \(1 < \rho\). Evaluate: \[ \intop\!\!\!\intop\nolimits_{D} \frac{dx\, dy}{x^{\gamma}y^{\rho}}. \]

346

Question 6.87

• (a) Evaluate \[ \intop\!\!\!\intop\nolimits_{D}\frac{\it dA}{(x^2+y^2)^{2/3}}, \] where \(D\) is the unit disk in \({\mathbb R}^2\).
• (b) Determine the real numbers \(\lambda\) for which the integral \[ \intop\!\!\!\intop\nolimits_{D}\frac{\it dA}{(x^2+y^2)^\lambda} \] is convergent, where again \(D\) is the unit disk.

Question 6.88

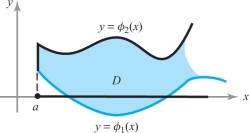
• (a) Discuss how you would define \({\intop\!\!\intop}_D f \ {\it dA}\) if \(D\) is an unbounded region—for example, the set of \((x,y)\) such that \(a\leq x< \infty\) and \(\phi_1(x)\leq y \leq \phi_2(x)\), where \(\phi_1 \leq \phi_2\) are given (Figure 6.24).
• (b) Evaluate \({\intop\!\!\intop}_D xy e^{-(x^2+y^2)}{\it dx}\,{\it dy}\) if \(x\geq 0,\) \(0\leq y\leq 1\).

Question 6.89

Using Exercise 8, integrate \(e^{-xy}\) for \(x\geq 0,1\leq y\leq 2\) in two ways. Assuming Fubini’s theorem can be used, show that \[ \int_{0}^{\infty}\frac{e^{-x}-e^{-2x}}{x}{\it dx}=\log 2. \]

Question 6.90

Show that the integral \[ \int^1_0\int^a_0(x/\sqrt{a^2-y^2})\,{\it dy}\,{\it dx} \] exists, and compute its value.

Question 6.91

Discuss whether the integral \[ \intop\!\!\!\intop\nolimits_{D}\frac{x+y}{x^2+2xy+y^2}{\it dx}\,{\it dy} \] exists where \(D=[0,1]\times [0,1]\). If it exists, compute its value.

Question 6.92

We can also consider improper integrals of functions that fail to be continuous on entire curves lying in some region \(D\). For example, by breaking \(D=[0,1]\times [0,1]\) into two regions, define and then discuss the convergence of the integral \[ \intop\!\!\!\intop\nolimits_{D}\frac{1}{\sqrt{|x-y|}}{\it dx}\,{\it dy}. \]

Question 6.93

Let \(W\) be the first octant of the ball \(x^2+y^2+z^2\leq a^2\), where \(x\geq 0,y\geq 0,z\geq 0\). Evaluate the improper integral \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{W}\frac{(x^2+y^2+z^2)^{1/4}}{\sqrt{z+(x^2+y^2+z^2)^2}}{\it dx}\,{\it dy}\,{\it dz} \] by changing variables.

Question 6.94

Let \(f\) be a nonnegative function that may be unbounded and discontinuous on the boundary of an elementary region \(D\). Let \(g\) be a similar function such that \(f(x,y)\leq g(x,y)\) whenever both are defined. Suppose \({\intop\!\!\intop}_Dg(x,y)\,{\it dA}\) exists. Argue informally that this implies the existence of \({\intop\!\!\intop}_Df(x,y)\,{\it dA}\).

Question 6.95

Use Exercise 14 to show that \[ \intop\!\!\!\intop\nolimits_{D} \frac{\sin^2(x-y)}{\sqrt{1-x^2-y^2}}{\it dy}\,{\it dx} \] exists where \(D\) is the unit disk \(x^2+y^2\leq 1\).

Question 6.96

Let \(f\) be as in Exercise 14 and let \(g\) be a function such that \(0\leq g(x,y)\leq f(x,y)\) whenever both are defined. Suppose that \({\intop\!\!\intop}_Dg(x,y)\, {\it dA}\) does not exist. Argue informally that \({\intop\!\!\intop}_Df(x,y) \, {\it dA}\) cannot exist.

Question 6.97

Use Exercise 16 to show that \[ \intop\!\!\!\intop\nolimits_{D}\frac{e^{x^2 + y^2}}{x-y}{\it dy}\,{\it dx} \] does not exist, where \(D\) is the set of \((x,y)\) with \(0\leq x\leq 1\) and \(0\leq y\leq x\).

Question 6.98

Let \(D\) be the unbounded region defined as the set of \((x,y,z)\) with \(x^2+y^2+z^2\geq 1\). By making a change of variables, evaluate the improper integral \[ \intop\!\!\!\intop\!\!\!\intop\nolimits_{D}\frac{{\it dx}\,{\it dy}\,{\it dz}}{(x^2+y^2+z^2)^2}. \]

Question 6.99

Evaluate \[ \int^1_0 \int^y_0 \frac{x}{y} {\it dx}\, {\it dy} \qquad\hbox{and}\qquad \int^1_0 \int^1_x \frac{x}{y} {\it dy}\, {\it dx}. \] Does Fubini’s theorem apply?

347

Question 6.100

In Exercise 17 of Section 5.2 we showed that \[ \int^1_0\!\! \int^1_0 \frac{x^2-y^2}{(x^2 +y^2)^2} {\it dy}\, {\it dx}\,\ne \int^1_0\!\!\int^1_0 \frac{x^2-y^2}{(x^2 +y^2)^2} {\it dx}\, {\it dy}. \] Thus, Fubini’s theorem does not hold here, even though the iterated improper integrals both exist. What went wrong?

Question 6.101

If \(0 \leq f(x,y) \leq g(x,y)\) for all \((x,y) \in D\), and the improper integral of \(g\) \[ {\intop\!\!\!\intop}_{D} g(x,y) \, {\it dx\, dy} \] exists, then \({\intop\!\!\intop}_{D} f(x,y) \, {\it dx\, dy}\) also exists. Use this fact and exercises 5 and 6 to argue that if \(0 < \alpha,\beta < 1\) and \(1 < \gamma,\rho\), then \[ {\intop\!\!\!\intop}_{D} \frac{{\it dx\, dy}}{x^{\alpha}y^{\beta} + x^{\gamma}y^{\rho}} \] exists, where \(D = [0, \infty) \times [0, \infty)\).

[HINT: Write \(D = D_1 \cup D_2\) and apply Exercise 14 to each \(D_{i}\) separately.]