exercises
411
Question 7.131
Consider the closed surface \(S\) consisting of the graph \(z=1 - x^{2} - y^{2}\) with \(z \geq 0\), and also the unit disc in the \({\it xy}\) plane. Give this surface an outer normal. Compute: \[ {\int\!\!\!\int}_{S} \textbf{F} \cdot \,d\textbf{S} \] where \(\textbf{F}(x,y,z) = (2x,2y,z)\).
Question 7.132
Evaluate the surface integral \[ {\int\!\!\!\int}_{S} \textbf{F} \cdot \,d\textbf{S} \] where \(\textbf{F}(x,y,z) = x\textbf{i} + y\textbf{j} + z^2 \textbf{k}\) and \(S\) is the surface parameterized by \(\Phi(u,v) = (2 \sin u, 3 \cos u, v)\), with \(0 \leq u \leq 2 \pi\) and \(0 \leq v \leq 1\).
412
Question 7.133
Let \(\textbf{F}(x,y,z) = ( x,y,z )\). Evaluate \[ {\int\!\!\!\int}_{S} \textbf{F} \cdot \,d\textbf{S}, \] where \(S\) is:
- (a) the upper hemisphere of radius 3, centered at the origin.
- (b) the entire sphere of radius 3, centered at the origin.
Question 7.134
Let \(\textbf{F}(x,y,z) = 2x\textbf{i} - 2y\textbf{j} + z^2 \textbf{k}\). Evaluate \[ {\int\!\!\!\int}_{S} \textbf{F} \cdot \,d\textbf{S}, \] where \(S\) is the cylinder \(x^{2}+y^{2}=4\) with \(z \in [0,1]\).
Question 7.135
Let the temperature of a point in \({\mathbb R}^3\) be given by \(T(x, y, z) = 3x^2 + 3z^2\). Compute the heat flux across the surface \(x^2 + z^2 = 2, 0\leq y\leq 2\), if \(k = 1\).
Question 7.136
Compute the heat flux across the unit sphere \(S\) if \(T(x, y, z) = x\). Can you interpret your answer physically?
Question 7.137
Let \(S\) be the closed surface that consists of the hemisphere \(x^2+y^2+z^2=1, z\geq 0\), and its base \(x^2+y^2\leq 1,z=0\). Let \({\bf E}\) be the electric field defined by \({\bf E}(x, y, z) = 2x{\bf i}\,+ 2y{\bf j} + 2z{\bf k}\). Find the electric flux across \(S\). (HINT: Break \(S\) into two pieces \(S_1\) and \(S_2\) and evaluate \({\int\!\!\!\int}_{S_1}{\bf E}\, {\cdot} \,d{\bf S}\) and \({\int\!\!\!\int}_{S_2}{\bf E} \, {\cdot} \,d{\bf S}\) separately.)
Question 7.138
Let the velocity field of a fluid be described by \({\bf F}=\sqrt{y}{\bf i}\) (measured in meters per second). Compute how many cubic meters of fluid per second are crossing the surface \(x^2 + z^2 = 1\), \(0\leq y\leq 1 , 0\le x \le 1\).
Question 7.139
Evaluate \({\int\!\!\!\int}_S\,(\nabla \times {\bf F}) \, {\cdot} \,d{\bf S}\), where \(S\) is the surface \(x^2 + y^2 + 3z^2 = 1, z\leq 0\) and \({\bf F}\) is the vector field \({\bf F}=y{\bf i} - x{\bf j} + zx^3y^2{\bf k}\). (Let \({\bf n}\), the unit normal, be upward pointing.)
Question 7.140
Evaluate \({\int\!\!\!\int}_S(\nabla \times {\bf F}) \, {\cdot}\, \,d{\bf S}\), where \({\bf F}=(x^2+y-4){\bf i}+ 3{\it xy} {\bf j}+(2xz+z^2){\bf k}\) and \(S\) is the surface \(x^2 + y^2 + z^2 = 16,z\geq 0\). (Let \({\bf n}\), the unit normal, be upward pointing.)
Question 7.141
Calculate the integral \({\int\!\!\!\int}_S {\bf F}\, {\cdot}\, \,d{\bf S}\), where \(S\) is the entire surface of the solid half ball \(x^2+y^2+z^2\leq 1,z\geq 0\), and \({\bf F}=(x+3y^5){\bf i}+(y+10xz){\bf j}+(z-{\it xy}){\bf k}\). (Let \(S\) be oriented by the outward-pointing normal.)
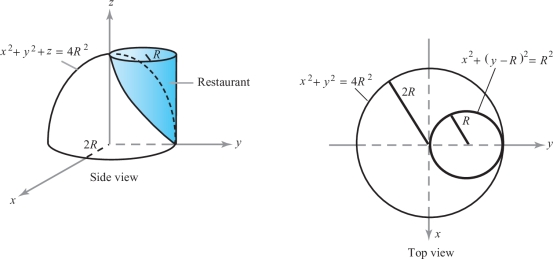
Question 7.142
footnote #A restaurant is being built on the side of a mountain. The architect’s plans are shown in Figure 7.51.
- (a) The vertical curved wall of the restaurant is to be built of glass. What will be the surface area of this wall?
- (b) To be large enough to be profitable, the consulting engineer informs the developer that the volume of the interior must exceed \(\pi\! R^4/2\). For what \(R\) does the proposed structure satisfy this requirement?
(c) During a typical summer day, the environs of the restaurant are subject to a temperature field given by \[ T(x,y,z)=3x^2+(y-R)^2+16z^2. \]
A heat flux density \({\bf V}=-k\,\nabla T\) (\(k\) is a constant depending on the grade of insulation to be used) through all sides of the restaurant (including the top and the contact with the hill) produces a heat flux.
What is this total heat flux? (Your answer will depend on \(R\) and \(k\).)
413
Question 7.143
Find the flux of the vector field \({\bf V}(x,y,z)=3{\it xy}^2{\bf i}+ 3x^2y{\bf j}+z^3{\bf k}\) out of the unit sphere.
Question 7.144
Evaluate the surface integral \({\int\!\!\!\int}_S{\bf F}\, {\cdot}\, {\bf n} dA\), where \({\bf F}(x,y,z)={\bf i}+{\bf j}+z(x^2+y^2)^2{\bf k}\) and \(S\) is the surface of the cylinder \(x^2+y^2\leq 1,0\leq z\leq 1\).
Question 7.145
Let \(S\) be the surface of the unit sphere. Let \({\bf F}\) be a vector field and \(F_r\) its radial component. Prove that \[ \int\!\!\!\int\nolimits_{S}{\bf F}\, {\cdot} \,\,d{\bf S} =\int^{2\pi}_{\theta = 0}\int^{\pi}_{\phi = 0}F_r\sin\phi \,d\phi \,d\theta. \]
What is the corresponding formula for real-valued functions \(f\)?
Question 7.146
Prove the following mean-value theorem for surface integrals: If \({\bf F}\) is a continuous vector field, then \[ \int\!\!\!\int\nolimits_{S}{\bf F}\, {\cdot}\, {\bf n}\,dS =[{\bf F}({\rm Q})\, {\cdot}\, {\bf n}({\rm Q})]A(S) \] for some point \({\rm Q}\in S\), where \(A(S)\) is the area of \(S\). [HINT: Prove it for real functions first, by reducing the problem to one of a double integral: Show that if \(g\geq 0\), then \[ \int\!\!\!\int\nolimits_{D} f\! g \,\,d\! A=f({\rm Q})\int\!\!\!\int\nolimits_{D} g \,d\! A \] for some \({\rm Q}\in D\) (do it by considering \(({\int\!\!\!\int}_Df\! g\,d\! A)/({\int\!\!\!\int}_Dg\,d\! A)\) and using the intermediate- value theorem).]
Question 7.147
Work out a formula like that in Exercise 15 for integration over the surface of a cylinder.
Question 7.148
Let \(S\) be a surface in \({\mathbb R}^3\) that is actually a subset \(D\) of the \({\it xy}\) plane. Show that the integral of a scalar function \(f(x, y, z)\) over \(S\) reduces to the double integral of \(f(x, y, z)\) over \(D\). What does the surface integral of a vector field over \(S\) become? (Make sure your answer is compatible with Example 6.)
Question 7.149
Let the velocity field of a fluid be described by \({\bf F} = {\bf i} + x{\bf j} + z{\bf k}\) (measured in meters per second). Compute how many cubic meters of fluid per second are crossing the surface described by \(x^2 + y^2 + z^2 = 1, z \geq 0\).
Question 7.150
- (a) A uniform fluid that flows vertically downward (heavy rain) is described by the vector field \({\bf F}(x, y, z) = (0, 0, -1)\). Find the total flux through the cone \(z = (x^2 + y^2)^{1/2}, x^2 + y^2 \leq 1\).
- (b) The rain is driven sideways by a strong wind so that it falls at a \(45^\circ\) angle, and it is described by \({\bf F}(x,y,z)=-(\sqrt{2}/2,0,\sqrt{2}/2)\). Now what is the flux through the cone?
Question 7.151
For \(a>0,b>0,c>0\), let \(S\) be the upper half ellipsoid \[ S=\bigg\{(x,y,z) \,\bigg| \,\frac{x^2}{a^2}+\frac{y^2}{b^2}+\frac{z^2}{c^2} =1,z\geq 0\bigg\}, \] with orientation determined by the upward normal. Compute \({\int\!\!\!\int}_S{\bf F}\, {\cdot}\, \,d{\bf S}\) where \({\bf F}(x,y,z)= (x^3,0,0)\).
Question 7.152
If \(S\) is the upper hemisphere \(\{(x,y,z) \mid x^2+y^2+ z^2=1,z\geq 0\}\) oriented by the normal pointing out of the sphere, compute \({\int\!\!\!\int}_S{\bf F}\, {\cdot} \,d{\bf S}\) for parts (a) and (b).
- (a) \({\bf F}(x,y,z)=x{\bf i}+y{\bf j}\)
- (b) \({\bf F}(x,y,z)=y{\bf i}+x{\bf j}\)
- (c) For each of these vector fields, compute \({\int\!\!\!\int}_S\,( \nabla \times {\bf F})\, {\cdot}\, \,d{\bf S}\) and \(\int_C{\bf F}\, {\cdot}\, \,d{\bf s}\), where \(C\) is the unit circle in the \({\it xy}\) plane traversed in the counterclockwise direction (as viewed from the positive \(z\) axis). (Notice that \(C\) is the boundary of \(S\). The phenomenon illustrated here will be studied more thoroughly in the next chapter, using Stokes’ theorem.)