Question 15.40

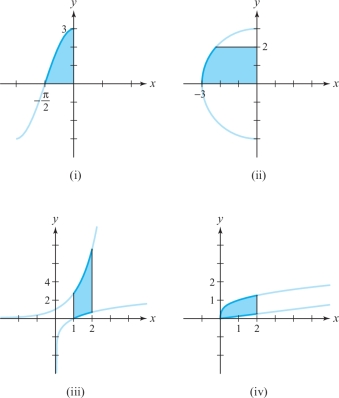
In parts (a) through (d) below, each iterated integral is an integral over a region \(D\). Match the integral with the correct region of integration.

• (a) \(\displaystyle\int_{1}^{2} \int_{\ln x}^{e^x} \, {\it dy}\, {\it dx}\)
• (b) \(\displaystyle\int_{0}^{2} \int_{(1/8) x}^{x^{1/3}} \, {\it dy}\, {\it dx}\)
• (c) \(\displaystyle\int_{0}^{2} \int_{-\sqrt{9 - y^2}}^{0} \, {\it dx}\, {\it dy}\)
• (d) \(\displaystyle\int_{0}^{3} \int_{\arccos y/3}^{0} \, {\it dx}\, {\it dy}\)

Question 15.41

Sketch the region \(D\) in \(\mathbb R^2\) that represents the region of integration:

• (a) \(\displaystyle\int_{-2}^{2} \int_{0}^{4 - y^2} (4 - x) \, {\it dx}\, {\it dy}\)
• (b) \(\displaystyle\int_{0}^{3} \int_{-x}^{x} (6 + y - 2x) \, {\it dy}\, {\it dx}\)

Question 15.42

Evaluate the following iterated integrals and draw the regions \(D\) determined by the limits. State whether the regions are \(x\)-simple, \(y\)-simple, or simple.

• (a) \(\displaystyle\int_0^1\int_0^{x^{2}} {\it dy}\, {\it dx} \)
• (b) \(\displaystyle\int_1^2\int_{2x}^{3x+1} {\it dy}\,{\it dx} \)
• (c) \(\displaystyle\int_0^1\int_1^{e^x} (x+y)\, {\it dy}\, {\it dx}\)
• (d) \(\displaystyle\int_0^1\int_{x^3}^{x^{2}} y\, {\it dy}\,{\it dx}\)

Question 15.43

Evaluate the following integrals and sketch the corresponding regions.

• (a) \(\displaystyle\int_{-3}^2\int_0^{y^2}(x^2+y)\, {\it dx}\, {\it dy} \)
• (b) \(\displaystyle\int_{-1}^1\int_{-2|x|}^{|x|}e^{x+y} {\it dy}\,{\it dx} \)
• (c) \(\displaystyle\int_0^1 \int_{0}^{(1-x^{2})^{1/2}} {\it dy}\,{\it dx} \)
• (d) \(\displaystyle\int_{0}^{\pi/2}\int_0^{\cos x} y \sin x {\it dy}\,{\it dx} \)
• (e) \(\displaystyle\int_{0}^1\!\int_{y^2}^y\, (x^n\,{+}\,y^m)\, {\it dx}\, {\it dy} , m,n\,{>}\,0\)
• (f) \(\displaystyle\int_{-1}^0\int_{0}^{2(1-x^{2})^{1/2}} x {\it dy}\, {\it dx}\)

289

Question 15.44

Use double integrals to compute the area of a circle of radius \(r\).

Question 15.45

Using double integrals, determine the area of an ellipse with semiaxes of length \(a\) and \(b\).

Question 15.46

What is the volume of a barn that has a rectangular base 20 ft by 40 ft, vertical walls 30 ft high at the front (which we assume is on the 20-ft side of the barn), and 40 ft high at the rear? The barn has a flat roof. Use double integrals to compute the volume.

Question 15.47

Let \(D\) be the region bounded by the positive \(x\) and \(y\) axes and the line \(3x + 4y = 10\). Compute \[ \intop\!\!\!\intop\nolimits_{D}\, (x^2+y^2) \,{\it dA}. \]

Question 15.48

Let \(D\) be the region bounded by the \(y\) axis and the parabola \(x=-4y^2+3\). Compute \[ \intop\!\!\!\intop\nolimits_{D}\, x^3 y\, {\it dx}\, {\it dy} . \]

Question 15.49

Evaluate \(\displaystyle\int_0^1\int_0^{x^2}(x^2+xy-y^2)\, {\it dy}\, {\it dx}\). Describe this iterated integral as an integral over a certain region \(D\) in the \(xy\) plane.

Question 15.50

Let \(D\) be the region given as the set of \((x,y)\), where \(1\leq x^2+y^2\leq 2\) and \(y\geq 0\). Is \(D\) an elementary region? Evaluate \({\intop\!\!\!\intop}_Df(x,y)\ {\it dA}\), where \(f(x,y)=1+xy\).

Question 15.51

Evaluate the following double integral: \[ \intop\!\!\!\intop\nolimits_{D} \cos y \, {\it dx}\, {\it dy}, \] where the region \(D\) is bounded by \(y=2x\), \(y=x\), \(x=\pi\), and \(x=2 \pi\).

Question 15.52

Evaluate the following double integral: \[ \intop\!\!\!\intop\nolimits_{D} xy \, {\it dA}, \] where the region \(D\) is the triangular region whose vertices are \((0,0), (0,2), (2,0)\).

Question 15.53

Use the formula \(A(D)= {\intop\!\!\!\intop}_D \, {\it dx}\, {\it dy}\) to find the area enclosed by one period of the sine function \(\sin x\), for \(0\leq x\leq 2\pi\), and the \(x\) axis.

Question 15.54

Find the volume of the region inside the surface \(z=x^2+y^2\) and between \(z=0\) and \(z=10\).

Question 15.55

Set up the integral required to calculate the volume of a cone of base radius \(r\) and height \(h\).

Question 15.56

Evaluate \({\intop\!\!\!\intop}_D y\ {\it dA}\), where \(D\) is the set of points \((x,y)\) such that \(0\leq 2x/\pi\leq y, y\leq \sin x\).

Question 15.57

From the ninth exercise, Section 15.2, \(\displaystyle\int_a^b\int_c^d f(x)g(y)\, {\it dy}\, {\it dx} = \left(\int_a^b f(x)\, {\it dx}\right)\!\) \(\displaystyle\left(\int_c^d g(y)\, {\it dy} \right)\). Is it true that \(\intop\!\!\!\intop\nolimits_D f(x) g(y)\ {\it dx} \ {\it dy} =\displaystyle\left(\int^b_a f(x)\ {\it dx} \right) \!\left(\int^{\phi_2(b)}_{\phi_1(a)}g(y)\ {\it dy}\right)\) for \(y\)-simple regions?

Question 15.58

Let \(D\) be a region given as the set of \((x, y)\) with \(-\phi(x)\leq y\leq \phi(x)\) and \(a\leq x\leq b\), where \(\phi\) is a nonnegative continuous function on the interval \([a,b]\). Let \(f(x,y)\) be a function on \(D\) such that \(f(x,y)=-f(x,-y)\) for all \((x,y)\in D\). Argue that \({\intop\!\!\!\intop}_D f(x,y)\, {\it dA}=0\).

Question 15.59

Use the methods of this section to show that the area of the parallelogram \(D\) determined by two planar vectors \({\bf a}\) and \({\bf b}\) is \(|a_1b_2-a_2b_1|\), where \({\bf a}=a_1 {\bf i}+a_2 {\bf j}\) and \({\bf b} =b_1{\bf i}+b_2 {\bf j}\).

Question 15.60

Describe the area \(A(D)\) of a region as a limit of areas of inscribed rectangles, as in Example 3.