Chapter 1. Successful Dieting: Everything Up to the Independent-Samples t Test

Introduction

Which Test Is Best?

true

You must read each slide, and complete any questions on the slide, in sequence.

Successful Dieting: Everything Up to the Independent-Samples t Test

By Warren Fass, University of Pittsburgh Bradford, and Susan A. Nolan, Seton Hall University

Pinola, M. (2016, February 12). The foods successful dieters eat more of, according to MyFitnessPal. https://lifehacker.com/the-foods-successful-dieters-eat-mUnsu10.ore-of-according-to-1758743956?utm_source=recirculation&utm_medium=recirculation&utm_campaign=fridayPM

Introduction

Successful Dieting: Everything Up to the Independent-Samples t Test

Tofu with boiled vegetables on plate and tomato closeup

mama_mia/Shutterstock

In this activity, we will consider various findings from the lifehacker.com article, "The Foods Successful Dieters Eat More Of, According to MyFitnessPal" (Pinola, 2016). Then you will identify statistical analyses that could be used to support the various conclusions.

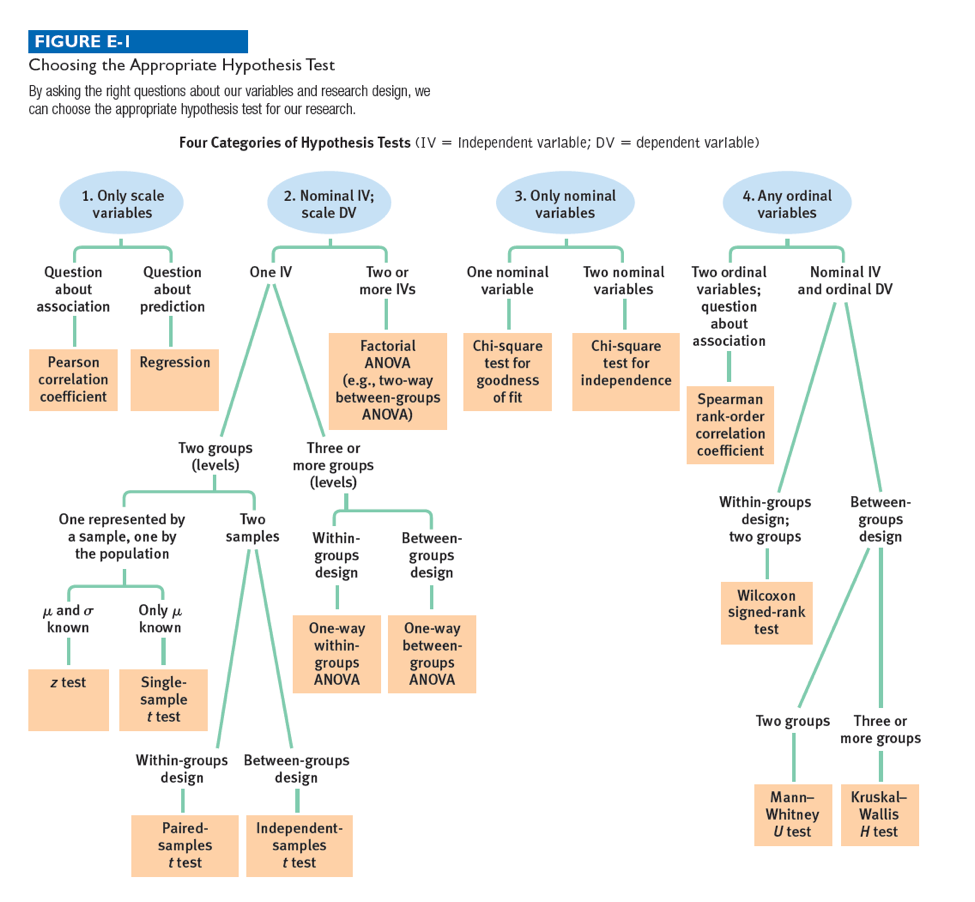
Guidelines for choosing the appropriate hypothesis test

Flashon Studio/Shutterstock

Choosing the Appropriate Hypothesis Test, Image Long Description

By asking the right questions about our variables and research design, we can choose the appropriate hypothesis test for our research.

Four Categories of Hypothesis Tests (IV = Independent variable; DV = dependent variable)

1. Only scale variables

1.1. Question about association

1.1.1. Pearson correlation coefficient

1.2. Question about prediction

1.2.1. Regression

2. Nominal IV; Scale DV

2.1. One IV

2.1.1. Two groups (levels)

2.1.1.1. One represented by a sample, one by the population

2.1.1.1.1. Mu and sigma known

2.1.1.1.1.1. z test

2.1.1.1.2. Only mu known

2.1.1.1.2.1. Single-sample t-test

2.1.1.2. Two samples

2.1.1.2.1. Within-groups design

2.1.1.2.1.1. Paired-samples t test

2.1.1.2.2. Between-groups design

2.1.1.2.2.1. Independent-samples t test

2.1.2. Three or more groups (levels)

2.1.2.1. Within-groups design

2.1.2.1.1. One-way within-groups ANOVA

2.1.2.2. Between-groups design

2.1.2.2.1. One-way between groups ANOVA

2.2. One-way between groups ANOVA

2.2.1. Factorial ANOVA (e.g., two-way between-groups ANOVA)

3. Only nominal variables

3.1. One nominal variable

3.1.1. Chi-square test for goodness of fit

3.2. Two nominal variables

3.2.1. Chi-square test for independence

4. Any ordinal variables

4.1. Two ordinal variables; question about association

4.1.1. Spearman rank-order correlation coefficient

4.2. Nominal IV and ordinal DV

4.2.1. Within-groups design; two groups

4.2.1.1. Wilcoxon signed-rank test

4.2.2. Between-groups design

4.2.2.1. Two groups

4.2.2.1.1. Mann-Whitney U test

4.2.2.2. Three or more groups

4.2.2.2.1. Kruskal-Wallis H test

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Plus Size Female Exercise Outdoor Happy Smile Under Sunny Blue Sky

Flashon Studio/Shutterstock

Have you ever tried to lose weight? If you are like us, and thousands of other people, you may have tried monitoring what you eat, following different diet plans, and/or exercising. With so many ads for weight loss methods, it can be confusing to figure out which option is best; however, there are a variety of Web sites and smart phone apps that might help.

For example, users of MyFitnessPal enter data (either on the Web site or on a smart phone) related to their food intake and the type of exercise performed (Pinola, 2016). MyFitnessPal has compiled these data from approximately 4.2 million users to distinguish the diets of successful dieters from those of unsuccessful dieters. MyFitnessPal operationally defined success in dieting as attaining a weight within 5% of the desired weight-loss goal. So, if you wanted to lose 20 pounds (9.1 kilograms), success would mean getting within 1 pound (0.5 kilogram) of that goal – that is, losing at least 19 pounds (8.6 kilograms). A total of 427,000 out of 4.2 million users met that criterion for successful dieters. The remaining 3.8 million users were considered the unsuccessful dieters. What can we learn from these data?

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

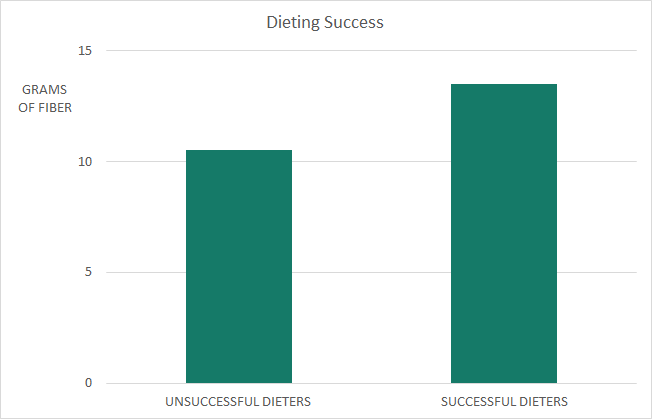
What do successful dieters eat compared to unsuccessful dieters? The answer may be surprising. Let’s look at one of the findings obtained by MyFitnessPal (Pinola, 2016):

Source: https://blog.myfitnesspal.com/eat-like-successful-myfitnesspal-user (p. 14)

Mean daily fiber intake of unsuccessful and successful dieters, Image Long Description

The graph shows that successful dieters had a mean daily fiber intake of 13.5 grams and the unsuccessful dieters had a mean daily fiber intake of 10.5 grams. Consequently, successful dieters eat 29% more fiber.

Mean daily fiber intake of unsuccessful and successful dieters
	Grams of fiber
Unsuccessful dieters	10.5
Successful dieters	13.5

As indicated in the graph, successful dieters had a mean daily fiber intake of 13.5 grams and the unsuccessful dieters had a mean daily fiber intake of 10.5 grams.

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Which statistical test could be used to determine if the successful dieters consumed significantly more daily fiber than the unsuccessful dieters?

Question

IBSseAoOkP1KAdM4IebO6ThsDXmNfo+ng8+Z38jJqzRF8xHhUCobEC7xH6ZOqc0/v3XtBSN0mDvKqDA8G+tUij/jO9xSFJUxUW7zRICQL52JUqI6N4yWl/fMsnEQKTPHfk8Mag8tA9wYSk//QgRLbd/fSQEVpTOWYge9X5of1GgwJRCb81k5fAUKHWq4AyjRrvGWZ6CYqwjAnpQJ

Correct! The researchers could have used an independent-samples t test because there is one nominal independent variable, success in dieting, with two levels or groups: successful and unsuccessful. There is one scale dependent variable, fiber intake in grams. And participants are in only one of the two groups, so it is a between-groups design.

Now you can skip ahead to the next example by clicking here. Or, if you would like more practice walking through the flowchart questions, then simply click the Next button in the bottom right corner of the screen.

Actually, that’s not the correct statistical analysis. Let’s walk through the questions on the flow chart in Appendix E to help you determine what analysis could be used in this case.

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

In which of the following four categories does this situation fall? Click if you’d like to see the data again. And click on the flowchart button if you’d like to see the overview for choosing the best test.

What do successful dieters eat compared to unsuccessful dieters? The answer may be surprising. Let’s look at one of the findings obtained by MyFitnessPal (Pinola, 2016):

The mean number of known victims of serial killers in North America compared to that of all countries combined.

Data from: https://en.wikipedia.org/wiki/List_of_serial_killers_by_number_of_victims (2015)

Mean daily fiber intake of unsuccessful and successful dieters, Image Long Description

Mean daily fiber intake of unsuccessful and successful dieters
	Grams of fiber
Unsuccessful dieters	10.5
Successful dieters	13.5

Question

sk97pNhgnDdS8cMuwHIcNGkOQu8zhZMruu2u88fM0qGdW97cS4aHwZRZbj0MaoIISfOaTTPjzGWg1FzVajDZage/lPkk+YotrDyrM4QRuYSjUH7OQ2HACIQv/DswADhdI5A+huzRe13qreW2Z1Br7AMENdjNksgE7me7mwahmFhtkzVYuVwBFlOiJ9OZW5vUlsPBQYcLL3jLqBfNxdCDJ0EbNFFxH5eS1bf2GZ8sMwV6K6EIQ4ef/XW9nyNdS1iE2U6c0LtvlZs=

Correct! There is at least one nominal independent variable and a scale dependent variable.

Actually, there is at least one nominal independent variable and a scale dependent variable.

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many nominal independent variables are there?

Question

Q/Akfrz/Qz13c1iia8RuB7BeUIbXiznSo1NNai0VR8qmpJfvVlqVP27jevCpjgCDm8TkDdykb5M1S/VixN6aB3xHZ1nWsgEVBEMob+G6Aok=

Correct! There is one nominal independent variable – dieter’s outcome. (The dependent variable, fiber intake in grams, is scale.)

Actually, there is only one nominal independent variable – dieter’s outcome. (The dependent variable, fiber intake in grams, is scale.)

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many levels does this independent variable have?

Question

Mo2sQ1oCUhznV+GnK8Wf81khqMWDU6hRlouB3iPgqhGwNqp8hV5Xo2x5F8H4dQW44S93hes84sOVhvoga+5CnZv5lto=

Correct! One level of the independent variable would be successful diet outcome, and the other level would be unsuccessful diet outcome.

Actually, there are two levels of the independent variable. One level of the independent variable would be successful diet outcome, and the other level would be unsuccessful diet outcome.

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many samples are there?

Question

erSrES0ZAKOTuDVIOkPYxo68vABJGDaUDXG6TmSIRkLosxO6LJX5akrQUQ0rEiotwDB3vhjEbWAnDNb8Ym+vlk3W3Cgp99rCvSXF1j7D9Odq2PTFPriRGfn1CyHSGVpFnDibDyZQo6o8DkbWaY85rZeLSiabcqypOzYupdwx3rUVpNJt

Correct! There are two samples, one consisting of the successful dieters and one consisting of the unsuccessful dieters.

Actually, there are two samples, one consisting of the successful dieters and one consisting of the unsuccessful dieters.

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

What type of design is this?

Question

k5gfwlifhUJcajKPDYPy7aXBa2aHyzwx/UO795ojOcNz+53Tt0mf3xcM0Nm5g8cOaYpjbioWobo5Lcuf5tNpzQ==

Correct! This is a between-groups design. Each participant appears in only one of the groups.

Actually, the design is a between-groups design. Each participant appears in only one of the groups.

Example 1 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Based on the answers to these questions, what statistical test could be used to determine if the successful dieters consumed significantly more daily fiber than the unsuccessful dieters?

Question

T5xdlkKPu/chfl0E/1uKeuQF20mmdz2wKS3nw0NczKlR4mfAW3gUFE8Z8CZapsslM/N42cLiybv5oSLOENaODXAzj5Cd6e5Ix92G4LBJ8YF2jzOOn6dcws8mkmAJtd9wphWeJn51miSnqNI0iWJ8qE5G+z+YP3xzSZNreVgHw9tIk8U6nJto10hqiu+PLiaf

Correct! The researchers could have used an independent-samples t test because there is one nominal independent variable, success in dieting, with two levels, or groups: successful and unsuccessful. There is one scale dependent variable, fiber intake in grams. And participants are in only one of the two groups, so it is a between-groups design.

Actually, that’s not the correct statistical analysis. The researchers could have used an independent-samples t test because there is one nominal independent variable, success in dieting, with two levels, or groups: successful and unsuccessful. There is one scale dependent variable, fiber intake in grams. And participants are in only one of the two groups, so it is a between-groups design.

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Bodrov Kirill/Shutterstock

Now let’s look at another finding from MyFitnessPal (Pinola, 2016). As previously indicated, successful dieters attained a weight loss within 5% of their goals. What about the unsuccessful dieters? What percentage within their weight-loss goals did they attain? How different was that percentage from the successful dieter’s percentage? Unfortunately, the unsuccessful dieter’s percentage was not provided in the article. However, we can set-up the situation as if those data were provided. For the purposes of this example, let’s assume that the successful dieters attained a mean of 3.7% from their weight-loss goal. Imagine that you randomly selected 20 unsuccessful dieters, obtained their percentages within their weight-loss goal and found their mean for this sample was 9.95%, with a standard deviation of 2.866%.

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

What statistical analysis could be used to determine whether the unsuccessful dieters attained a significantly different percentage from their weight-loss goal, on average, than did the successful dieters?

Question

Z4M0eLlwsZ70WJPajowvWIW1QIOa5giXRXIw7sgknIgobnrIt5KGhUo7TTAhNa4b6BKnv0LNU7TwfIn19AnNCiRtLEWSS+Tei/s5RTEVkMdjLHLJLFGbpM7EzqeuALGNw3ZteG4ROFQ/rzKveq5iy9H+tLlVmJ/cuBBKtY8zkXnZDqJUHe80vjcp+7RoXkCxgCj48bc9mQf+DhJi

Correct! The researchers could have used a single-sample t test because there is one nominal independent variable –dieter’s outcome. There are two levels or groups: unsuccessful and successful. The former is represented by a sample and the latter by a population. There is a scale dependent variable, percentage within weight-loss goal, and we know the population mean, but not the population standard deviation.

Now you can skip ahead to the next example by clicking here. Or, if you would like more practice walking through the flowchart questions, then simply click the Next button in the bottom right corner of the screen.

That’s not the correct statistical analysis. Let’s walk through the questions on the flow chart in Appendix E to help you determine what analysis could be used in this case.

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Now let’s look at another finding from MyFitnessPal (Pinola, 2016). As previously indicated, successful dieters attained a weight-loss within 5% of their goals. What about the unsuccessful dieters? What percentage within their weight-loss goals did they attain? How different was that percentage from the successful dieter’s percentage? Unfortunately, the unsuccessful dieter’s percentage was not provided in the article. However, we can set-up the situation as if those data were provided. For the purposes of this example, let’s assume that the successful dieters attained a mean of 3.7% from their weight-loss goal. Imagine that you randomly selected 20 unsuccessful dieters, obtained their percentages within their weight-loss goal and found their mean for this sample was 9.95%, with a standard deviation of 2.866%.

Question

Correct! There is at least one nominal independent variable and a scale dependent variable.

Actually, there is at least one nominal independent variable and a scale dependent variable.

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many nominal independent variables are there?

Question

Q/Akfrz/Qz13c1iia8RuB7BeUIbXiznSo1NNai0VR8qmpJfvVlqVP27jevCpjgCDm8TkDdykb5M1S/VixN6aB3xHZ1nWsgEVBEMob+G6Aok=

Correct! There is one nominal independent variable – dieter’s outcome. (The dependent variable, percentage from weight-loss goal, is scale.)

Actually, there is only one nominal independent variable – dieter’s outcome. (The dependent variable, percentage from weight-loss goal, is scale.)

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many levels does this independent variable have?

Question

Mo2sQ1oCUhznV+GnK8Wf81khqMWDU6hRlouB3iPgqhGwNqp8hV5Xo2x5F8H4dQW44S93hes84sOVhvoga+5CnZv5lto=

Correct! There are two levels or groups: unsuccessful and successful.

Actually, there are two levels or groups: unsuccessful and successful.

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many samples are there?

Question

F+6tP8Au4CvEs3lOf9LMVirQuTgSrCPBtsRZ6RM5Tj2W+NkAFedJqhj/DWdmlpIVzg1W27TEBgaFHxMHQBEkYpDXiHLca2YHqRv9WFfZBoNxS4IEOEpGWdg86Z5JyYAzL372HLwnwXTo4aYtWrYGZ1po2GYLoIIIrxLGY4oRDiSbWzO8

Correct! There are two levels or groups: unsuccessful and successful dieters. The former is represented by the sample and the latter by a population.

Actually, there are two levels or groups: unsuccessful and successful. The former is represented by the sample and the latter by a population.

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

For the level represented by a population, what parameters do you know with respect to the scale dependent variable?

Question

f+xoaMsVRA8/kHGTamnYqLXVqtMn9oexz80p5bW94H0EpT/L4ID+m9uRFtS04DNC72DlEK5HkvdKHBoyXbtIyR46QO4wIUXsH5opPVNhFAJrPTQ4zJO3QnAsNZK2ROz/Pn6mOMUz1qhgjrGWB05VEw==

Correct! For the scale dependent variable, percentage within weight-loss goal, we know only the population mean of 3.7% – not the population standard deviation.

Actually, for the scale dependent variable, percentage within weight-loss goal, we just know the population mean of 3.7% – not the population standard deviation.

Example 2 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Based on the answers to these questions, what test could be used?

Question

jmUQj6ZR7AulOuiihyA7eKCN9JQXzWSQBuODR1YfmJBoklQvUBlYojUWPuTQklYIEy/eGeM3gv+rk4yjhcYpNTMOdI5IIpB1wN2678WJ0V+8vuuVaOBWE/nOYrxKdnPXgE2zDcD2eEn3rDJm7894nuLEg/993X7j2dvG1Y5H9nz/ftnJGfFff7eynhVa7miN

Correct! The researchers could have used a single-sample t test. There is one nominal independent variable – dieter’s outcome. There are two levels or groups: unsuccessful and successful. The former is represented by a sample and the latter by a population. There is a scale dependent variable, percentage within weight-loss goal, and we know the population mean, but not the population standard deviation, for this measure.

Actually, we could have used a single-sample t test. There is one nominal independent variable – dieter’s outcome. There are two levels or groups: unsuccessful and successful. The former is represented by a sample and the latter by a population. There is a scale dependent variable, percentage within weight-loss goal, and we know the population mean, but not the population standard deviation, for this measure.

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Let’s look at a final set of data from MyFitnessPal (Pinola, 2016). MyFitnessPal researchers found that successful and unsuccessful dieters consumed similar percentages of calories from carbohydrates, fats, and proteins each day. However, as we can see in this bar graph, among all dieters, there seemed to be different percentages of calories consumed per day attributed to carbohydrates, fats, and proteins.

Source: https://1y2u3hx8yml32svgcf0087imj-wpengine.netdna-ssl.com/wp-content/uploads/2016/01/Successful_Users_Infographic_sec2.png (p. 14)

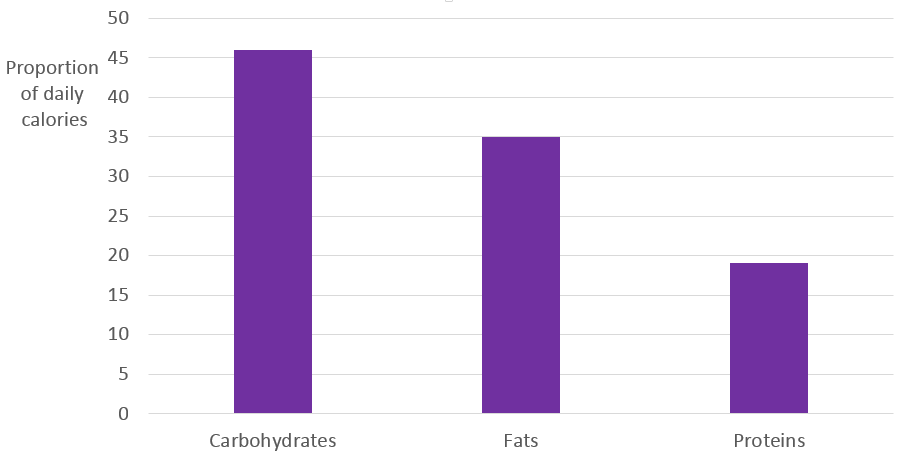
Percentages of calories from carbohydrates, fats, and proteins consumed by successful and unsuccessful dieters each day, Image Long Description

Both groups consumed 46% of carbs, 35% of fats and 19% of proteins.

Percentages of calories from carbohydrates, fats, and proteins consumed by successful and unsuccessful dieters each day.
	Proportion of daily calories
Carbohydrates	46
Fats	35
Proteins	19

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Imagine that you randomly selected 100 dieters and recorded the percentage of carbohydrate calories and the percentage of fat calories that each of them consumed in a day. What statistical test could be used to determine if the dieters consumed a significantly greater percentage of carbohydrate calories than fat calories per day?

Question

/SQmF9yOYHiJixJgLZ1YJgXiokm8EvlF7f0Yl1qS/xoSPMtFa2tAD0dwnb+ZOk7o9A6royXZRKpMElvswTY4jvQRkRl6RpW6sFZmLl2KHFDrrXRgtsGmpBlW/kBNBFZ+AhBHAhVd/qBKXSl7a1ULVifZePAJkjQShi6Ef1YZwqpno/ubIJk2pn/ljzdjFHKIlbYUwpMG4Tav3HUd

Correct! The researchers could have used a paired-samples t test. There is one nominal independent variable, type of food category, with two levels or groups: carbohydrates and fats. There is one scale dependent variable, percentage of calories. All participants have consumed both types of food.

Now you can skip ahead to the end of the activity by clicking here. Or, if you would like more practice walking through the flowchart questions, then simply click the Next button in the bottom right corner of the screen.

Actually, that’s not the correct statistical analysis. Let’s walk through the questions on the flow chart in Appendix E to help you determine what analysis could be used in this case.

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Let’s look at a final set of data from MyFitnessPal (Pinola, 2016). The bar graph shows that successful and unsuccessful dieters consumed similar percentages of calories from carbohydrates, fats, and proteins each day. However, among all dieters, there seemed to be different percentages of calories consumed per day attributed to carbohydrates, fats, and proteins.

Data from: https://1y2u3hx8yml32svgcf0087imj-wpengine.netdna-ssl.com/wp-content/uploads/2016/01/Successful_Users_Infographic_sec2.png

Percentages of calories from carbohydrates, fats, and proteins consumed by successful and unsuccessful dieters each day, Image Long Description

Both groups consumed 46% of carbs, 35% of fats and 19% of proteins.

Percentages of calories from carbohydrates, fats, and proteins consumed by successful and unsuccessful dieters each day.
	Proportion of daily calories
Carbohydrates	46
Fats	35
Proteins	19

Question

Correct! There is at least one nominal independent variable and a scale dependent variable.

Actually, there is at least one nominal independent variable and a scale dependent variable.

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many nominal independent variables are there?

Question

Q/Akfrz/Qz13c1iia8RuB7BeUIbXiznSo1NNai0VR8qmpJfvVlqVP27jevCpjgCDm8TkDdykb5M1S/VixN6aB3xHZ1nWsgEVBEMob+G6Aok=

Correct! There is one nominal independent variable – type of food category. (The dependent variable, percentage of calories, is scale.)

Actually, there is only one nominal independent variable – type of food category. (The dependent variable, percentage of calories, is scale.)

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many levels does this independent variable have?

Question

Mo2sQ1oCUhznV+GnK8Wf81khqMWDU6hRlouB3iPgqhGwNqp8hV5Xo2x5F8H4dQW44S93hes84sOVhvoga+5CnZv5lto=

Correct! The independent variable has two levels or groups: carbohydrates and fats.

Actually, the independent variable has two levels or groups: carbohydrates and fats.

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

How many samples are there?

Question

erSrES0ZAKOTuDVIOkPYxo68vABJGDaUDXG6TmSIRkLosxO6LJX5akrQUQ0rEiotwDB3vhjEbWAnDNb8Ym+vlk3W3Cgp99rCvSXF1j7D9Odq2PTFPriRGfn1CyHSGVpFnDibDyZQo6o8DkbWaY85rZeLSiabcqypOzYupdwx3rUVpNJt

Correct! There are two samples, one consisting of the carbohydrates and one consisting of fats.

Actually, there are two samples, one consisting of the carbohydrates and one consisting of fats.

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

What type of design is this?

Question

mE/BzzBhIzYNHpmwBzVj8ykwikrj4Fnq2o5IoPYXFHrvU024YN3He5knq55rB3PIO7MtPaayb1wkko+EmmvgnQ==

Correct! This is a within-groups design. Each participant consumed both types of food.

Actually, the design is a within-groups design. Each participant consumed both types of food.

Example 3 of 3

Successful Dieting: Everything Up to the Independent-Samples t Test

Based on the answers to these questions, what test could be used?

Question

V0DvHoCPFJGIKzaSQygSd788VQ67NyRgPeRw55rSGceab4iEegQcs9UDeREes8hPjcLNoMX8VUdSA8+dzV6cvvqx4Dq/TgaqPP9xy8NwSl77tZvidmP891ZNB/ROqORPLviIpwhmV+wPOBrAzyeD15+RBCXJoPDAAqm2uzVN+kxe5qqQF6uNw/lJAW9aVani898KLvZeZRtWH+iz

Correct! The researchers could have used a paired-samples t test because there is one nominal independent variable, type of food category, with two levels, or groups: carbohydrates and fats. There is one scale dependent variable, percentage of calories. And participants have consumed both types of food, so it is a within-groups design.

Actually, that’s not the correct statistical analysis. The researchers could have used a paired-samples t test because there is one nominal independent variable, type of food category, with two levels, or groups: carbohydrates and fats. There is one scale dependent variable, percentage of calories. And participants have consumed both types of food, so it is a within-groups design.

1.1 Activity Completed!

Congratulations! You have completed the activity and gained some good experience in choosing the best hypothesis test.