Chapter 9.
Overview
Generality
We’ve seen that the Energy-Interaction Model can inform us about the final state of a system if we know the initial state and have information about the interactions that occur in between initial and final. What that model doesn’t tell us, and can’t tell us, is whether a process can be reversed, whether the system can start in the final state and end up in the initial state. Energy is conserved just as well if time is reversed and our final state comes first with the initial state later and reversed interactions. On the other hand, there are very many processes that proceed only in one direction, a direction that we call “toward equilibrium”. These processes are never seen to happen in reverse even though energy conservation would allow it. A list of macroscopic processes that proceed in only one direction (toward equilibrium) includes i) most chemical reactions, ii) heat transfer, and iii) diffusion, although we could list many others. The Statistical Model of Thermodynamics uses a microscopic picture of these macroscopic systems to “explain” why these processes happen only in one direction and how the “initial” state differs from the “final” state so that it is easy to identify which is which. The idea we will use can be put simply: Processes proceed toward equilibrium because that is the most likely thing to happen and for macroscopic systems the probability of the system moving toward equilibrium is so much larger than the probability of moving in the other direction that we never see the reverse process.
Focus
In the statistical model of thermodynamics, our focus is on using statistics involving microscopic variables to understand the macroscopic properties associated with entropy and equilibrium.
Model Summary
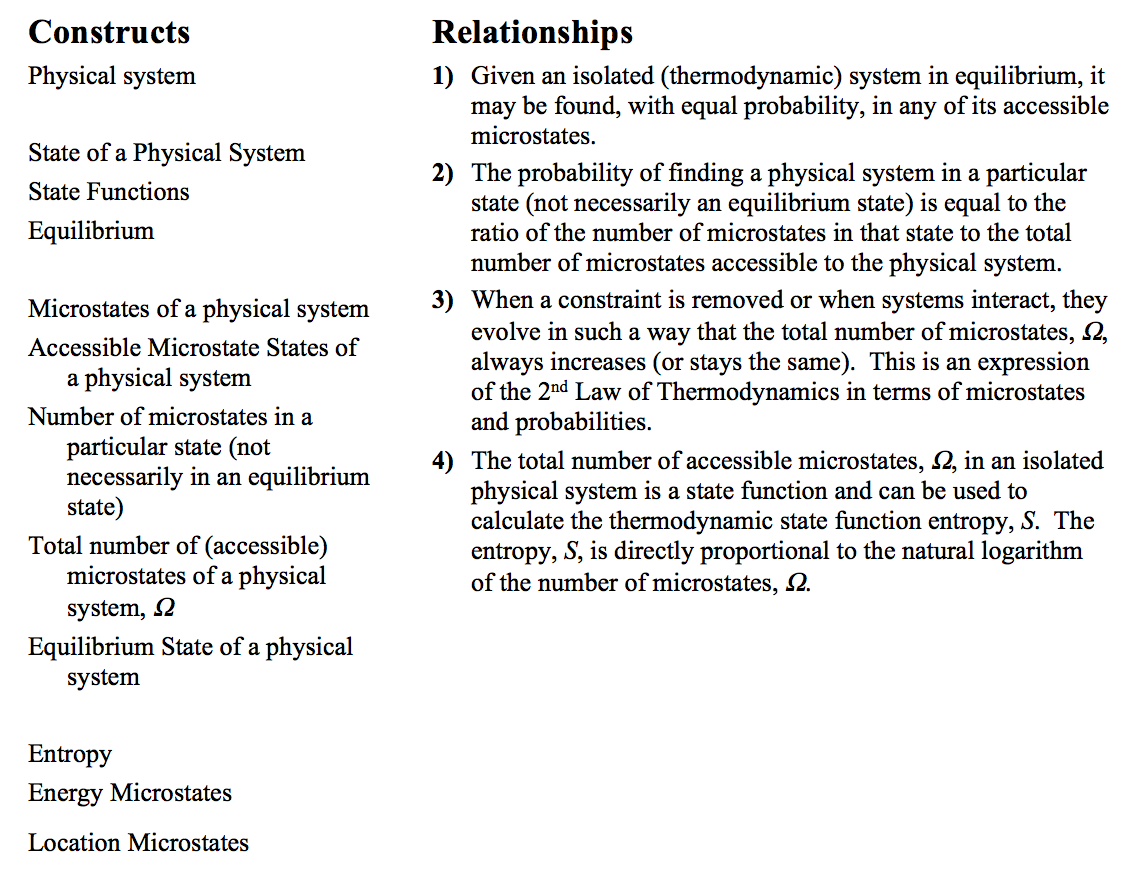
Representational Tools
Algebraic Representations
P(state i) = (# of microstates possible within state i) / Ω
where P is the probability of finding the system in state i (P is not the pressure here).
S = kB lnΩ, where kB is known as Boltzmann’s constant
kB = 1.381 x 10-23 J/K
Elaboration
Expanded Definitions of Model Constructs
Physical System
Thermodynamics will always deal with macroscopic physical systems. We use the word “macroscopic” to include any system made up of a very large number of individual components where “large number” need only be large enough for statistical methods to be valid. A system made up of one million atoms might, for our purposes, be considered “macroscopic” even though it may have a diameter of 10’s of nanometers so that it couldn’t be seen with the naked eye.
State of a Physical System
In thermodynamics, the “state” of a macroscopic physical system will be defined by a set of macroscopic variables including P, T, V, U, etc. which are either measureable or calculable. These variables are macroscopic in nature but may be different for different macroscopic pieces of our system. For instance, the temperature of one macroscopic part of our physical system might be different than the temperature of another macroscopic piece of our physical system.
State Functions
The state variables (such as P, T, V, U, etc.) are related to each other by equations of state. So, since each of these state variables can be written as a function of other state variables, each state variable can be also referred to as a state function.
Equilibrium
Experiment shows us that when we wait long enough the state variables associated with a physical system become constant in time. We call this state the equilibrium state. Once a physical system is in an equilibrium state it can be moved to a new equilibrium state only by way of an interaction with one or more other physical systems.
Microstates of a physical system and Number of microstates in a particular state (not necessarily in an equilibrium state)
A macroscopic physical system whose state is defined by a specific set of state variables is still made up of very many components (the microscopic components are usually atoms or molecules) and any one of these components can have a wide range of possible energies and, usually, can be found anywhere in the volume of the physical system. Specifying the energy of each atom/molecule (we really need to specify all independent terms in the kinetic energy of each particle) and the location of each atom/molecule will completely specify one “microstate” of the physical system. In other words, if there are Avogadro’s number (NA) of atoms we would need to give at least 6 NA pieces of information to specify a microstate of this physical system. As the atoms change location and transfer energy between themselves the physical system is moving through different microstates.
Accessible Microstate States of a physical system
Some microstates may be unavailable to a physical system. For instance, if our physical system consists of two objects at different temperatures and these two objects are not thermally connected in any way (so that they cannot exchange energy) then the only accessible microstates will have total energy of object 1 equal to a constant U1 and total energy of object 2 equal to a constant U2. On the other hand, if these two objects are thermally connected then microstates with energy of object 1 less then or greater than U1 become accessible as long as the total energy, U1 + U2, is still the same. So releasing the constraints on a system increases the number of accessible microstates. Only microstates that are accessible to the system within the time scale of an experiment are included in our model.
For almost any isolated physical system, experiment suggests that all microstates that are accessible to that system have equal probability of occurring. So our model is that each accessible microstate of an isolated system has the same probability of occurring. This is similar to assuming that each of the two microstates of a coin, heads or tails, has equal probability of occurring (experiments also almost always show this to be a good model for coin tossing).
Total number of (accessible) microstates of a physical system, Ω
We use the Greek letter Omega, Ω, as the number of accessible microstates. Once we know the state variables (say N, V, and U) so that we know the state of our physical system then we can calculate the number of accessible microstates and because Ω is completely determined by these state variables, Ω is a state variable.
Given that all accessible microstates of an isolated system are equally probable, the probability of the physical system finding itself in state i (with pressure Pi, temperature Ti, etc.) is:
P(state i) = (# of microstates possible within state i) / Ω
where Ω is the total # of accessible microstates (which includes the microstates within state i as well as all other accessible microstates). Note that P can be probability or pressure so be careful.
Equilibrium State of a physical system
The equilibrium state is the state that the system always ends up in when we wait long enough because that is the most probable state. If a physical system is not in an equilibrium state then it will spontaneously change in a way that brings it closer to the equilibrium state and the reason that it will spontaneously change is because that is the most likely thing (highest probability, Pi) to happen.
Even though all Ω microstates are available to a system its equilibrium state always includes just a subset of the accessible microstates. For instance, even though two objects in thermal contact with total energy U can share that energy in many many ways they always end up sharing it so that each available energy mode has the same energy on average and the reason this happens is that this state (state i, say) of equal sharing of energy is the most likely state (has the largest number of microstates).
Entropy
We define the entropy to be S = kB lnΩ where kB is known as Boltzmann’s constant. Note that S is a state variable because Ω is a state variable AND that a system that is not in equilibrium will change its distribution of particles within the volume and/or energy among the particles so that Ωi is maximized. In other words, an isolated system will change in such a way that S is maximized.
Stotal = S1 + S2 which means that entropy is an additive state variable like energy, U, or number of particles, N.
Energy Microstates and Location Microstates
Notice that the number of microstates is the number of ways of distributing i) N particles within the volume, V AND ii) distributing the total internal energy, U, among the N particles. Because both V and U are important here they both need to be considered in discussing equilibrium for actual isolated systems.
For instance, in the adiabatic (Q = 0) expansion of a gas the volume increases so, thinking of volume only, one might conclude that Ω increased. However, work energy is taken out during the expansion so Eth decreased so, thinking of energy only, one might conclude that Ω decreased. Did Ω decrease or increase? Our thermodynamic model lets us use dS = dQ/T to conclude that ΔS = 0 because Q = 0 so we can conclude that Ω didn’t change at all because S didn’t change at all in this adiabatic process. The volume effect exactly cancels the energy effect in this process but that is not a general rule. In some processes giving the atoms more volume (or increasing their mobility in some other way) will be more important that taking away energy (usually Eth) and in some processes the reverse will be true.