Chapter 1. Experiment 15

Determination of Specific Heat and Various Heats of Reactions

Purpose of the Experiment

Determine the specific heat of aluminum and to determine the enthalpy change for an acid/base neutralization reaction and the dissolution reaction of ammonium nitrate.

Background Required

You should be familiar with basic laboratory techniques for volume, mass, and temperature measurements. You should also be familiar with the concepts of heat and enthalpy, and with stoichiometric calculations.

Background Information

A calorimeter is an apparatus used to measure the quantity of heat involved in a chemical or physical change. If the measurements are made at constant pressure, ex., atmospheric pressure, then heat (q) equals the enthalpy change (ΔH).

Specific heat (J/g°C) is defined as the amount of heat (q in Joules) required to change the temperature of one gram of a substance by 1°C as shown in Equation 1. This can be rearranged into Equation 2. (ΔT = T_final – T_initial)

The First Law of Thermodynamics states Energy is conserved. So the heat released by the system plus the heat gained by the surroundings must equal zero. Thus the heats of the system and surrounding are equal in magnitude, but opposite in sign as shown in Equation 3. Since polystyrene coffee cups are good insulators, it is assumed that all of the heat lost or gained by the system will be transferred to the water/solution (surroundings).

Example

Problem

A 24.3 g Fe sample is heated to 100.0°C in a hot water bath. The metal is quickly transferred to 30.0 mL of water at 22.0°C in a coffee cup calorimeter. The maximum temperature reached by the water was 28.3°C. Determine q_Fe and the specific heat of Fe. (Specific heat_H2O = 4.18 J/g°C and density_H2O = 1.00 g/ mL)

Solution

(1) The Fe is the system and the water is the surroundings. Calculate the q_H2O, using Equation 5. You have to first determine the mass of the water using density, as in Equation 4.

mass_H2O = mL_H2O × density_H2O (Eq. 4)

mass_H2O = 30.0 mL × 1.00 g/mL = 30.0 g

q_H2O = {specific heat_H2O × mass_H2O × ΔT_H2O} (Eq. 5)

q_H2O = {4.18 J/g°C × 30.0 g × (28.3 – 22.0°C)}

q_H2O = 790.0 J

(2) Determine q_Fe, using Equation 3.

q_Fe (J) = –q_H2O (J)

q_Fe = –790.0 J

(3) Determine specific heat of Fe, using Equation 6.

Example

Problem

When 2.71 g of KOH(s) dissolves in 50.0 mL of water in a coffee cup calorimeter, the temperature rises from 23.8°C to 37.1°C. Determine ΔH for this dissolution of KOH in (kJ)/mol KOH dissolved. (Assume that the specific heat of the solution is the same as the specific heat of water.)

Solution

(1) The KOH is the system and the aqueous solution is the surroundings. Calculate the q_solution using Equation 7. You have to first determine the mass of the solution, which is the mass of the water AND the mass of the KOH dissolved.

mass_solution = (50.0 mL × 1.00 g/mL) = 2.71 g KOH = 52.7 g

q_solution = {specific heat_solution × mass_solution × ΔT_solution} (Eq. 7)

q_solution = {4.18 J/g°C × 52.7 g × (37.1 – 23.8°C)}

q_solution = 2930 J

(2) Determine q_KOH, using Equation 3.

q_KOH (J) = –q_solution (J)

q_KOH = –2930 J = –2.93 kJ

(3) Determine moles of KOH. Then determine ΔH_rxn in kJ/mol KOH, using Equation 8.

In This Experiment

In Part I of this experiment, the system is the Aluminum rods and the surroundings are the water. The specific heat of Aluminum will be determined. The percent error will also be determined using the literature specific heat of Al.

In Part II, the system is the reaction and the aqueous solution is the surroundings.The mass of the solution equals the combined total mass of HCl and NaOH solutions. Assume the density of the HCl and NaOH solutions are equal to that of water (1 g/mL). Use the density to determine the mass. The ΔH_rxn for the reaction between HCl and NaOH will be determined per mole of HCl neutralized in the reaction. (HCl is the limiting reagent and NaOH is present in excess.)

HCl(aq) + NaOH(aq) → NaCl(aq) + H₂O(ℓ)

In Part III, the system is the NH₄NO₃ and the aqueous solution is the surroundings.The mass of the solution equals the combined total mass of NH₄NO₃ and water. The ΔH_rxn for dissolving NH₄NO₃ in water will be determined per mole of NH₄NO₃ dissolved.

NH₄NO₃(s) →NH₄NO₃(aq)

Procedure

Always Wear Safety Goggles and Use Good Lab Practices

Chemical Alert:

Part I

Determination of the Specific Heat of Aluminum

1. Start a boiling water bath using about 150 mL in a 250 mL beaker on a hot plate. After the water is boiling, turn the heat setting to ~7 to maintain a constant boil.

2. Weigh and record the mass of two Aluminum rods together.

3. Connect a temperature probe to the LabQuest. On the METER SCREEN, tap the MODE box. On the MODE SCREEN, keep the mode as Time Based and adjust the time length to 3 minutes. Click OK and place the temperature probe in the boiling water bath.

Once the water is boiling, gently add the rods to the boiling water (but do not start data collection yet).

Record the temperature of the water bath and remove the temperature probe.

This is T_initial for the Aluminum.

After five minutes, the rods and the boiling water will reach a thermal equilibrium, i.e., temperature of the rods will equal the temperature of the water.

4. Build a simple calorimeter by placing two polystyrene coffee cups inside a 400 mL beaker. Add 30 mL of water to the coffee cups and wait one minute.

Insert the temperature probe into the water. Record T_initial of the water.

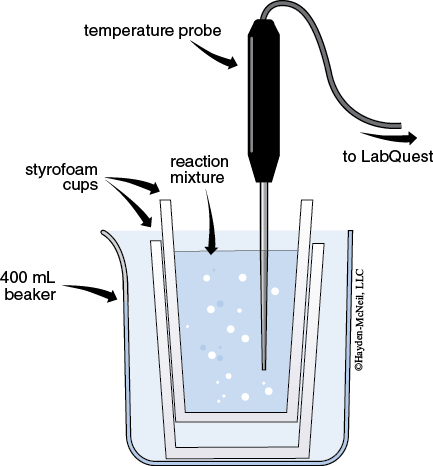
As quickly as possible add the rods to the calorimeter using crucible tongs. Gently swirl the calorimeter for three minutes.

When the data collection is complete, tap Analyze at the top of the screen and then select Statistics. Tap the check box for Temperature. Drag the stylus from the initial to highest temperature. (It highlights the selected data and gives you the statistics.)

5. Record the max temperature as T_final for the water and T_final for the Aluminum. Tap the meter icon to return to the METER SCREEN.

6. Pour out the water. Dry off the metal rods and return them to the dispensing table.

Dry out the calorimeter and turn off the hot plate.

Part II

Determination of ∆H_rxn for the Reaction of HCl and NaOH

7. Measure 50.0 mL of 1.0 M NaOH in a clean, dry graduated cylinder and pour into the DRY calorimeter. Place the temperature probe in the solution. After one minute, record T_initial of the solution.

8. Measure 40.0 mL of 1.0 M HCl in a second, clean, dry graduated cylinder.

9. Tap PLAY to begin data collection. Tap the DISCARD button to discard the last run.

Pour the HCl solution as quickly as possible into the NaOH solution in the calorimeter.

10. At the end of 3 minutes, Analyze using Statistics on the Temperature data set. Highlight the region of initial to the highest temperature with the stylus. Record the max temperature of the solution as T_final.

11. Pour the solution down the drain with running water. Rinse the calorimeter, graduated cylinders, and thermometer with water.

Dry the coffee cups and repeat the procedure for a second trial.

Part III

Determination of ΔH_rxn for NH₄NO₃ Dissolving in Water

12. Measure 30.0 mL of water in a graduated cylinder and pour into the DRY calorimeter. Place the temperature probe in the solution. After one minute, record T_initial of the solution.

13. Using the weighing by difference technique, obtain about 4 g of NH₄NO₃. Record all masses.

14. Tap PLAY to begin data collection and discard the previous run.

Add the NH₄NO₃as quickly as possible to the water in the calorimeter. Gently swirl the calorimeter (to dissolve the solid) for three minutes.

At the end of 3 minutes, Analyze using Statistics on the Temperature data set. Highlight the region of initial to the lowest temperature with the stylus. Record the minimum temperature of the solution as T_final.

15. Pour the reaction solution down the drain with running water. Rinse the calorimeter and temperature probe with water.

Return the LabQuest and temperature probe.

Return the coffee cups to dispensing table.

Calculations

Part I: Determination of the Specific Heat of Aluminum

a. Calculate the mass of the water for each trial.

b. Calculate q_H₂_O and q_{Al metal} in Joules for each trial.

c. Calculate the specific heat of Aluminum.

d. Calculate the % error for the specific heat of Aluminum using the specific heat of Al and the accepted specific heat of Al as 0.90 J/g°C using Equation 9.

EQUATION

Part II: Determination of ΔH_rxn for the Neutralization Reaction of HCl and NaOH

e. Calculate the combined mass of the solution for each trial.

f. Calculate q_solution and q_rxn in Joules for each trial.

g. Calculate the moles of HCl neutralized.

h. Calculate the ∆H_rxn in kJ/mol of HCl neutralized for each trial. Average the two values of ∆H_rxn.

Part III: Determination of ΔH_rxn for NH₄NO₃ Dissolving in Water

i. Calculate the combined mass of the solution (solid 1 water).

j. Calculate q_solution and q_rxn.

k. Calculate the moles of NH₄NO₃ dissolved for each trial.

l. Calculate the ∆H_rxn in kJ/mol of NH₄NO₃ dissolved.

Discussion

1. Summarize the results of this experiment.

2. What is the quality of the results? Do the results seem reasonable?

3. What were the errors or possible errors in the experiment?

4. How would the errors affect the results of the experiment?

Study Questions

1. Define the various terms used: calorimeter, specific heat, q, and the First Law of Thermodynamics.

2. A 20.94 g sample of an unknown metal is heated to 99.4°C in a hot water bath. The metal is quickly transferred to 100 mL of water at 22.0°C in a coffee cup calorimeter. The maximum temperature reached by the water was 24.6°C. What is the q_water in J and what is q_metal in J?

What is the specific heat of the metal in J/g°C?

3. In a neutralization experiment, 35 mL of 1.0 M HNO₃ was mixed with 50 mL of 1.0 M NaOH. How many moles of nitric acid were neutralized in this reaction?

4. When 35 mL of 1.0 M HNO₃ was mixed with 50 mL of 1.0 M NaOH in a coffee cup calorimeter, the temperature of the solution rose from 24.8°C to 31.7°C. What is the q_solution in J and what is q_rxn in J?

What is the ΔH_rxn in kJ/mol of HNO₃ neutralized?

5. When 5.0 g of CaCl₂ was dissolved in 50 mL of water in a coffee cup calorimeter, the temperature of the solution rose from 22.3°C to 30.5°C. What is the q_solution in J and what is q_rxn in J?

What is the ΔH_rxn in kJ/mol of CaCl₂ dissolved?

6. It was assumed that the water (or solution) absorbed all of the heat lost by the system. Do you feel this assumption was valid? If some of the heat were lost to the coffee cup or air, how would this affect your calculation of q_system?

7. One way to judge the accuracy of your calorimeter is to compare the results for a known system. Examine your % error of specific heat of Aluminum. What does this indicate about the accuracy of your calorimeter?

8. For the following errors, determine if the error will affect the calculation of q_system. If it does affect the calculation, then state if it will increase or decrease the value of q_system.

a. Placing the hot aluminum rod on the lab bench for 30 seconds before adding it to the calorimeter.

b. Adding 50 mL of water, but only recording the volume of water as 30 mL.

c. The solid NH₄NO₃ did not all dissolve after adding it to the water.

d. Some of the HCl splashed out while pouring it into the calorimeter.

9. Examine the signs of ΔH_rxn for the dissolution of NH₄NO₃ and for the neutralization reaction of HCl and NaOH. Classify both reactions as either endothermic or exothermic. Does this agree with your observations, e.g., did the coffee cup seem to get warmer or colder?