Chapter 22. Observations of Black Holes

22.1 Introduction

Astronomers detect black holes based on their gravitational influence on objects near them, as in this binary system.

The goals of this module: After completing this exercise, you should be able to:

1. Explain why black holes are difficult to observe directly.
2. Describe how astronomers determine the existence of black holes.
3. Discuss the various types of black holes.

In this module you will explore:

1. How X-rays are given off around black holes, even though energy can't escape from black holes themselves.
2. How astronomers discovered that black holes are found in a variety of masses, depending on their origin and location.
3. How astronomers believe there are types of black holes which exist, but haven't been detected yet.

Why you are doing it: Black holes are fascinating objects which spark the imagination of science and science fiction lovers everywhere, but can be difficult to detect because they do not give off any energy of their own. While difficult, it is not impossible to detect black holes, and in this activity you will discover how astronomers are able to do so.

22.2 Background

Today the existence of black holes is widely accepted among members of the scientific community, despite the fact that it is impossible to directly observe one (since black holes give off no radiation of their own). The theoretical existence of black holes was first suggested over 200 hundred years ago.

In 1783, geologist and amateur astronomer John Mitchell suggested that if it was possible for an object to be massive enough, then close to its surface the escape speed (the speed needed to escape from the gravitational pull of the object) would be greater than the speed of light. Even light would be unable to escape from this extremely massive object, assuming light was affected by the force of gravity. In 1796, mathematician Pierre-Simon Laplace discussed similar ideas in early publications of his book. Astronomers today now refer to these Newtonian concepts of black holes as "dark stars."

Summary

The suggestion that objects from which not even light could escape could exist resurfaced in 1915, when Albert Einstein put forth his theory of general relativity. Around the same time, Karl Schwarzschild theorized the existence of a point mass gravitationally strong enough to behave like a black hole, leading to the understanding of the event horizon which surrounds a black hole.

Illustration of how a hypothetical nearby 10-solar-mass black hole would distort the view of stars behind it.

If black holes are considered "black" because not even light can escape from their gravitational pull, then how is it possible to detect them?

22.3 The Existence of Black Holes

So how do we know that black holes exist? While we cannot observe them directly, we can observe their effects on other objects. As material falls into a black hole, tidal stresses pull on the object, eventually breaking it into pieces. At the same time, the material is heated to high temperatures due to tidal friction. Material this hot radiates energy mostly in the X-ray portion of the electromagnetic spectrum. If enough material falls in towards the black hole, or if there is a steady flow of material, a flattened region of gas called an accretion disk will form around the black hole and will radiate X-rays. Therefore, we can detect X-ray emission not from the black hole itself, but from the accretion disk around it.

Summary

An artist's illustration of a black hole with a binary companion.

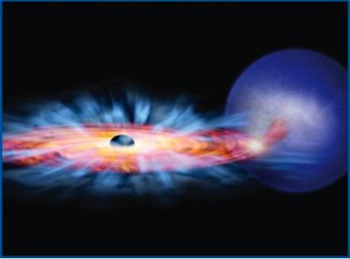
In some cases the black hole may actually be a part of a binary system, with material from the outer envelope of its binary companion spiraling down towards it, such as in the case of Cygnus X-1, illustrated above. Cygnus X-1 is a strong source of X-ray emission (hence the "X") in the constellation of Cygnus, the swan. When astronomers looked at Cygnus X-1 visually, they found a star, HDE 226868, shown as the white object in the figure above. While astronomers could not observe a companion to HDE 226868, they found out through spectroscopic analysis that HDE 226868 is indeed orbiting around another object. The spectrum of HDE 226868 contains absorption lines which are shifting toward the red side of the spectrum and then to the blue, and then back again, due to the Doppler shifting of its light as it first moves away from us, then towards us as it orbits.

Based on the period of time over which it takes this shifting of spectral lines to repeat itself, astronomers can determine the amount of time it takes HDE 226868 to orbit its unseen companion once. Based on the maximum Doppler shift of the spectral lines, astronomers can determine the speed of HDE 226868 as it orbits. Using this information, astronomers were then able to use Newton's modified version of Kepler's third law to determine a lower limit to the mass of the unseen object, and determined that it has a mass of at least 7 solar masses. Based on this evidence, the fact that we cannot observe it otherwise, and yet it is a powerful source of X-rays, astronomers strongly believe that the unseen companion is, in fact, a black hole.

22.4 Supermassive Black Holes

A supermassive black hole in the center of the galaxy.

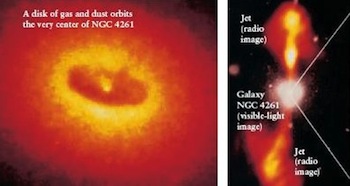
Systems like Cygnus X-1 provide observational evidence suggesting the existence of black holes on the order of a few solar masses which formed as the result of the deaths of massive stars, but these are not the only type of black holes that are known to exist. Astronomers also have evidence for the existence of supermassive black holes, with masses on the order of a million solar masses or more. These black holes are found in the centers of galaxies, but how did they form? Astronomers believe that as a galaxy forms, its center may have accumulated large amounts of material which, if dense enough, could have collapsed into a black hole. Galaxies such as our own have masses greater than 100 billion solar masses, so a million-solar-mass black hole represents only a tiny fraction of the total mass of a galaxy.

How do we know these supermassive black holes exist? The same way we know smaller black holes exist, by their gravitational effect on nearby objects. The picture above shows a suspected black hole at the center of the galaxy NGC 4261. The picture on the left taken with the Hubble Space Telescope clearly shows a disk of gas and dust orbiting around a central object. Doppler measurements of the light coming from the edges of the disk indicate that the material in it is moving at a speed of hundreds of kilometers per second. Based on this speed, and the distance that this material is from the central object, we use Kepler's third law to calculate a mass of the central object of 1.2 billion solar masses, but it is no larger than the size of our solar system! Only a black hole can fit those criteria.

22.5 The Supermassive Black Hole in our Galaxy

Similar measurements can be made for the supermassive black hole in the center of our Galaxy. The animation below shows the positions and trajectories for 6 stars which orbit around the center of our Galaxy. On the top of the animation is a scroll bar that allows you to change the mass of the central object about which the stars are orbiting. Move the scroll bar left (lower mass) and right (greater mass) to see how the mass of the central object affects the stars' orbits.

Summary

Based on the average distance of the orbiting stars from the center of our Galaxy, as well as their periods of orbit, astronomers have used Kepler's third law to calculate a mass of 3.7 million solar masses for the central object in the Milky Way Galaxy, which is known as Sagittarius A*.

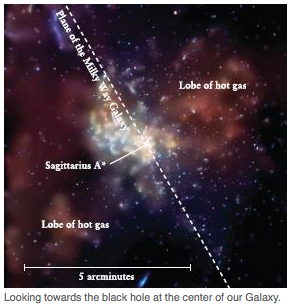
The figure above displays a composite X-ray image of Sagittarius A*, the suspected supermassive black hole in the center of our Galaxy. In this figure, you can see two lobes of hot gas with temperatures around 2 million Kelvins. These must have been heated by X-rays from the central region. As we saw with Cygnus X-1, X-ray emission is expected from accretion disks around black holes. Astronomers believe that supermassive black holes lie at the centers of all galaxies.

22.6 Black Holes of Other Masses

Possible evidence of intermediate-mass black holes.

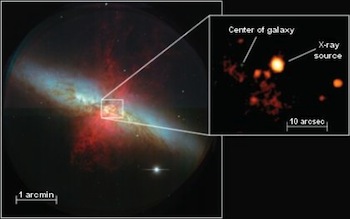
Astronomers have observational evidence of solar-mass black holes resulting from the collapse of massive stars, as well as supermassive black holes near the centers of galaxies, but what about black holes with masses somewhere in between? Until recently, no observational evidence for black holes in this range existed. However, in 2000, astronomers discovered an X-ray source in galaxy M82 (shown in the figure to the right), which, based on how luminous the source appears at X-ray wavelengths, suggests how much material must be falling onto the source to sustain this luminosity. It turns out that in order to maintain its high level of X-ray luminosity, the central object must have a mass of at least 500 solar masses, yet rapid fluctuations in the luminosity suggest that the source is rather small. Only an intermediate black hole fits this description.

There has also been speculation by Stephen Hawking of the existence of primordial black holes, which would have formed during the formation of the Universe, 13.7 billion years ago. Hawking hypothesizes that regions of the early Universe could have been dense enough to collapse into extremely low mass black holes, with masses as large as Earth's, or as small as 50 billionths of a kilogram. At the moment, though, the existence of these primordial black holes is still speculation; no primordial black holes have been detected yet.

22.7 Quick Check

Indepth Activity: Observations of Black Holes