Chapter 26. The Finite Age of the Universe

26.1 Introduction

The COBE satellite first measure the spectrum of cosmic microwave background radiation, an indicator that we live in a universe of finite age.

The goals of this module: After completing this exercise, you should be able to:

1. Explain Olbers' paradox.
2. Explain the significance of the Cosmic Microwave Background Radiation.
3. List the observational evidence that astronomers have that supports a finite age for the universe.

In this module you will explore:

1. Why the night time sky is dark.
2. How we know that the age of the universe is finite.
3. What astronomers mean by the lookback time of an object.

Why you are doing it: Today almost all astronomers believe that the universe had a definite beginning with the Big Bang, but that wasn't always the case. At one point some astronomers believed that the universe was infinite in size and age; that it has always existed. In this activity we will explore the evidence which supports a finite age for the universe.

26.2 Background

Astronomers have measured the ages of a number of celestial objects and found an upper limit to their ages, suggesting a finite age for the universe.

Astronomers have determined a number of methods for measuring the ages of objects within our universe. Within our Solar System, we can use radioactive dating to determine the ages of rocks from various bodies, such as the Earth, the Moon, Mars and meteorites. Along with numerical modeling of our Sun, we have determined an age for our Solar System of approximately 4.6 billion years.

As we move outward into our Galaxy, we can determine the ages of stars within young open clusters, such as those shown in the figure above. By studying the HR diagram of a cluster of stars, we can determine when the stars within the cluster formed. When we do this, we find that these systems tend to be younger than 7 - 9 billion years old. We don't find young open clusters older than this. When looking at globular clusters in the halo of our Galaxy, we find that these stars are older, around 11 billion years old or so.

If we use Hubble's law to approximate the age of the universe, tracing back history to a time when all objects were together in one point, we get an age of 13.7 billions years. No matter what celestial objects we determine ages for, we find that there seems to be a limit to how old objects in our universe are. We never find objects older than 13.7 billion years. The fact that there are no objects older than this age suggests that the universe has not been around forever, but has a finite age. There are other ways that astronomers know that the universe has a finite age.

26.3 Why is the Night Sky Dark?

26.4 Olbers' Paradox

When we look at the sky at night it is dark.

Obviously when we look at the sky at night it is dark, yet according to the scenario on the previous page, it shouldn't be. How do we explain this apparent paradox? The solution is simple and was first put forth by the astronomer Heinrich Olbers. We assumed that galaxies were distributed uniformly throughout an infinite universe. This assumption is incorrect. The universe, as we can observe it, is not infinite, but rather is finite in size. If that is the case, then the total amount of light we receive from all of the galaxies we can observe is finite as well, and not enough to illuminate the night time sky.

Why is the universe as we can observe it finite? It is because the speed of light is finite (incredibly fast, but finite). Traveling at a speed of 300,000 kilometers per second, light seems to travel instantaneously from a light bulb to your eyes when you turn on a switch, but over very long distances, it takes light time to travel. Light from the Sun, at a distance of 150,000,000 km away from the Earth, takes a little over 8 minutes to reach us. Light from objects even farther away takes even longer. When we look at objects in space, we are seeing the light which has left them some time ago in the past. We are actually observing the objects as they appeared in the past. How far back in time we are looking when observing an object is known as the lookback time. The farther away an object lies, the larger its lookback time.

26.5 Observational Evidence of the Beginning of the Universe

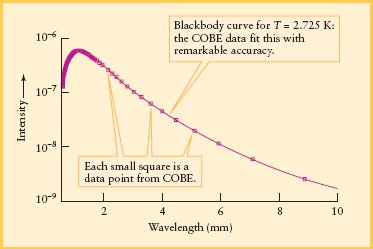
The spectrum of the cosmic microwave background.

Other than Olbers' paradox, what evidence do we have of this finite observable universe?

In the early 1960's, two scientists, Arno Penzias and Robert Wilson, were working on a radio antenna designed for communication purposes. As they were testing it, they were confused by the presence of a background signal no matter where they pointed their antenna. After carefully removing any possible source that could be causing the background noise, Penzias and Wilson still detected this background signal. Fortunately, at around the same time, physicists Robert Dicke and P. J. E. Peebles predicted the existence of such a background signal, and Penzias and Wilson discovered that what they were detecting was not just random noise but actually the remnant radiation from the beginning of the universe itself.

What Penzias and Wilson discovered has become known as the Cosmic Microwave Background (CMB) Radiation. Launched in 1989, astronomers used the COBE satellite to measure the CMB over a range of wavelengths, and determined that this radiation obeys blackbody radiation laws, peaking at a wavelength of 1.06 mm, as shown in the figure.

This near constant temperature detected in all directions is considered the remains from the tremendous release of energy from the Big Bang itself.

26.6 So What is the Cosmic Microwave Background Radiation?

To understand what we are observing when we look at the Cosmic Microwave Background Radiation, we need to go back to the very formation of the universe itself. When the universe began, 13.7 billion years ago, a tremendous amount of energy was created and formed what came to be our universe. As this energy expanded in all directions, matter existed in the form of elementary particles (electrons, protons, etc.). At this point, any photons traveling through the universe were quickly absorbed and scattered by free electrons, making it difficult for photons to freely travel. We say that the universe at this point was opaque. As time went on, the universe continued to expand, and as it did so it cooled off.

To see how the photons moved in the early universe, click on the start button.

Roughly 380,000 years after the Big Bang, the universe cooled to a temperature of 3,000 Kelvin. At this point, temperatures were low enough that elementary particles could combine to form atoms. When this occurred, photons were then free to move through the universe, and the universe became transparent. Photons moving around at this time had a distribution of wavelengths as determined by blackbody laws, and peaked at a wavelength of around 1 μm. As the photons continued to move through the universe, the cosmological redshift shifted their wavelengths to longer and longer wavelengths, causing their corresponding blackbody temperature to drop. As we observe the photons today, we are measuring a blackbody temperature of only 2.7 K because of this.

To see how the photons moved after the first atoms formed, click on the Continue button. What we are observing, therefore, when we detect the Cosmic Microwave Background Radiation, are the photons that became free to move at this period of transition between the opaque universe and the transparent universe, as depicted in the animation above. Because of the lookback time of objects at far distances, if light was not free to move through the universe before a certain time in the history of our universe, then we cannot see to a time before this either. This defines the extent of the finite observable universe in which we live. We will never be able to observe a time earlier than this.

26.7 Quick Check Quiz

Indepth Activity: The Finite Age of the Universe