Chapter 6. Change in mechanical energy when nonconservative forces act (6-26)

Question

lXazFIJws+JtMHteBxxx5Xow3gI4o5cn6eivkXcb2q9SPFakyREuBf+Na4zpXbgy0UzY2F4fJG2NknbocBlUP/3GCeNbFV8qik/ElBkvvfclOECdVJTlNfLz81jNwl+G+fArbr2PUzxKzV27

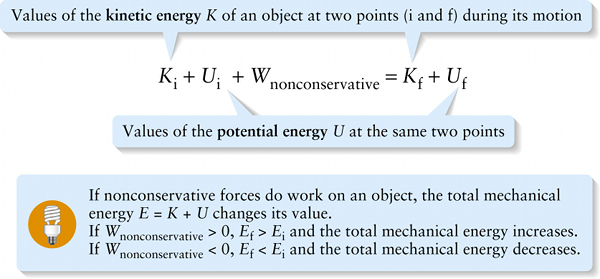
{"title":"Values of the kinetic energy K of an object at two points (i and f) during its motion","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"1,1,18,26\"},{\"shape\":\"rect\",\"coords\":\"234,1,255,22\"}]"} {"title":"Values of the potential energy U at the same two points","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"118,11,119,13\"},{\"shape\":\"rect\",\"coords\":\"39,0,59,24\"},{\"shape\":\"rect\",\"coords\":\"275,1,296,21\"}]"}

Question

/oKIfyKEbVq11INyzCT5Bj03mdpvPWpBryDxUdrLL+ge1ZJujMGEtya2+VzGeOIYLaGAIwlVJHh0G+qmkODyaGSK0IxQDOne0RwW/w==

{"title":"Values of the kinetic energy K of an object at two points (i and f) during its motion","description":"Incorrect","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"1,1,18,26\"},{\"shape\":\"rect\",\"coords\":\"234,1,255,22\"}]"} {"title":"Values of the potential energy U at the same two points","description":"Correct!","type":"correct","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"118,11,119,13\"},{\"shape\":\"rect\",\"coords\":\"39,0,59,24\"},{\"shape\":\"rect\",\"coords\":\"275,1,296,21\"}]"}

Review

Equation 6-26 gives us more insight into the difference between conservative and nonconservative forces. While the pencil in Figure 6-28 is in flight—that is, during the time from when it leaves your hand to when it returns to your hand—the value of \(E = K + U\) remains the same. Kinetic energy decreases and changes into potential energy as the pencil ascends, but the potential energy is turned back into kinetic energy as the pencil falls.