Chapter 7. External force and momentum change for an object (7-23)

Question

TZhMbmbo8T+wYvHwn2Jmw+onDR4rlGwhex+u29+UpBeT1vURTwQwIbqHfh5WADjzt9je/SEiVYI=

{"title":"The sum of all external forces acting on an object","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"40,22,148,45\"}]"} {"title":"Duration of a time interval over which the external forces act","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"118,11,119,13\"},{\"shape\":\"rect\",\"coords\":\"163,14,181,36\"}]"} {"title":"Change in the momentum vector p of the object during that time interval","description":"Incorrect","type":"incorrect","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"219,17,237,34\"},{\"shape\":\"rect\",\"coords\":\"274,12,301,34\"}]"}

Question

P+t0T4fB+PcLcQrVKumMVxqGQQIkjqS3fKHK4aY+Y/+eGlp2LovU6zmf7NY/VTdK8OiVAIgKwJDSPgCbpum8vford+Q=

{"title":"The sum of all external forces acting on an object","description":"Incorrect","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"40,22,148,45\"}]"} {"title":"Duration of a time interval over which the external forces act","description":"Correct!","type":"correct","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"118,11,119,13\"},{\"shape\":\"rect\",\"coords\":\"163,14,181,36\"}]"} {"title":"Change in the momentum vector p of the object during that time interval","description":"Incorrect","type":"incorrect","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"219,17,237,34\"},{\"shape\":\"rect\",\"coords\":\"274,12,301,34\"}]"}

Question

R4QTozr0IuCRAAa4qKdw36mUCwBN+83L6MDlZPNOIc+Mau0EyQg8Fw9zhKkGhnEu6PrzF5qHlpm8f9rzrWe+GiIXAfvvjKd2aaRG3Q==

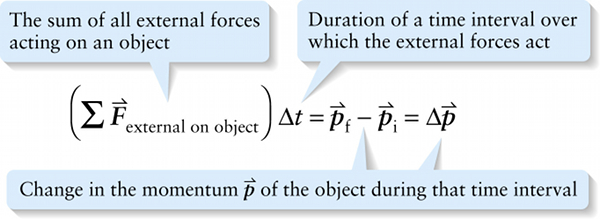
{"title":"The sum of all external forces acting on an object","description":"Incorrect","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"40,22,148,45\"}]"} {"title":"Duration of a time interval over which the external forces act","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"118,11,119,13\"},{\"shape\":\"rect\",\"coords\":\"163,14,181,36\"}]"} {"title":"Change in the momentum vector p of the object during that time interval","description":"Correct!","type":"correct","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"219,17,237,34\"},{\"shape\":\"rect\",\"coords\":\"274,12,301,34\"}]"}

Review

We learned in Section 7-3 that if external forces act on a system of objects for a time \(\Delta{t}\), the result is a change in the momentum of the system from an initial value \(\vec{P}_i\) to a final value \(\vec{P}_f\). This change is given by Equation 7-13: \(\left(\sum{\vec{F}_\mathrm{external\ on\ object}}\right) \Delta{t} = \vec{P}_f - \vec{P}_i\)

Now suppose the system is made up of only a single object. Then we can replace the symbol \(\vec{P}\) (for the momentum of a system) by \(\vec{p}\) (for the momentum of a single object), and Equation 7-13 becomes: