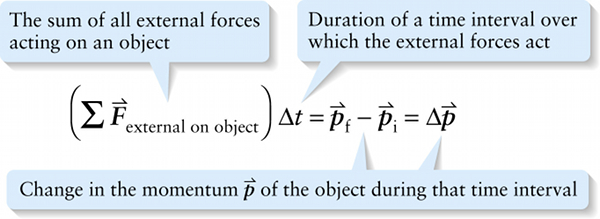
External force and momentum change for an object (7-23)

Balloon Art Concept Check: Click on the part of the equation that is described by the bubble text shown

Question 1 of 3

Question

Duration of a time interval over which the external forces act
Feedback
{"title":"The sum of all external forces acting on an object","description":"Wrong","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"4,6,150,44\"}]"} {"title":"Duration of a time interval over which the external forces act","description":"Correct!","type":"correct","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"171,17,183,34\"}]"} {"title":"Change in the momentum p of the object during that time interval","description":"Incorrect","type":"incorrect","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"218,20,238,34\"},{\"shape\":\"rect\",\"coords\":\"287,19,300,38\"}]"}

Review

Since the speed of light in a vacuum \(c\) is the maximum speed an object can acquire,this expression says that the maximum kinetic energy that an object can acquire is \((1/2)mc^2\). This contradicts the idea that there should be no limit on an object’s kinetic energy. Clearly, we need an improved equation for kinetic energy.

There is a similar problem with the Newtonian expression for momentum. We saw in Section 7-6 that the change in momentum of an object is determined by the external forces on the object and the duration of the time interval over which the forces act: