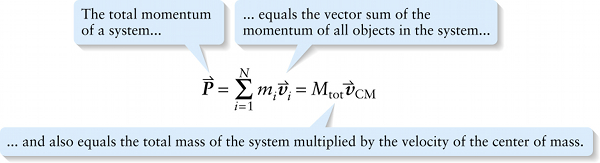
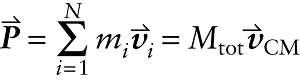Total momentum and the velocity of the center of mass (7-33)

Review

The quantity \(m_i\vec{v}_i\) on the right-hand side of Equation 7-32 should be familiar: This is just the momentum of the \(i\)th object in the system. So \(\sum_{i=1}^N m_i\vec{v}_i\) is the vector sum of the momentum of all objects that make up the system. This is just the \(\textit{total}\) momentum of the system, which we denote as \(\vec{P}\). If we multiply Equation 7-32 by the total mass of the system \(M_\mathrm{tot}\), we get