Chapter 13. Pressure variation for a sinusoidal sound wave propagating in the +x direction (13-9)

Review

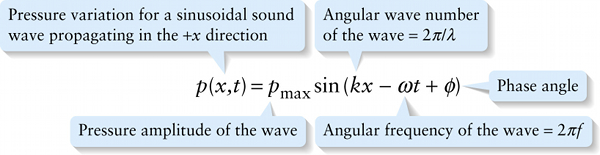
Since a sound wave is a longitudinal wave, a positive value of \(y(x,t)\) means that the medium is displaced in the positive \(x\) direction, while a negative value of \(y(x,t)\) means that the medium is displaced in the negative \(x\) direction (Figure 13-9). As a result, as a sound wave propagates it squeezes together air molecules at some places along the wave, resulting in regions of air that have higher density and higher pressure. Adjacent regions of air are slightly depleted of air molecules and therefore have lower density and lower pressure. As Figure 13-9 shows, the pressure is greatest or least at points where the displacement is \(\textit{zero}\). So while the pressure is also described by a sinusoidal function, it is shifted by one quarter-cycle from the wave function for displacement \(y(x,t)\). That’s why we’ve drawn the displacement graph in Figure 13-9 as a \(\textit{cosine}\) curve but the pressure variation graph as a sine curve. In particular, if the displacement is described by Equation 13-6,

\(y(x,t) = A \cos\ (kx - \omega{t} + \phi)\)

the pressure variation is given by