Chapter 14. Ideal gas law in terms of number of molecules (14-6)
Question
Hc6ZasWYRRYf/rShcG9V8BY1U9rX2ke8O/gPWm5eq8kZguO8O0PuwThuRBU=
Question
i5XrrbaL0sRiC8vSbSdY8jYXAB62QOt/GleHKCwE46gY7iC1ryxzfFVy1mWTNhxQBp6aPN2aeMo=
Question
A1DNMDK9RQNrFNSE8Xl81QraJRihc3su/kb9bS2+9b6RpFW0So/hlM5DXrZfc4EgDeKiP+U8vfkQr+Pi
Question
/9IODz7PTiIPwtNrsDRjeLOlsZnOKcIzkZAAnM9HT0bYowYc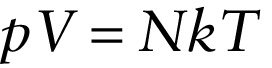
Question
oS7kZmm9qo1JqVqqr9YdDuZg2pAqzIrwrbhmxHrriE0UaB+X6u0PoSM9X2g=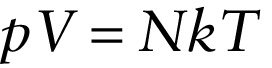
Review
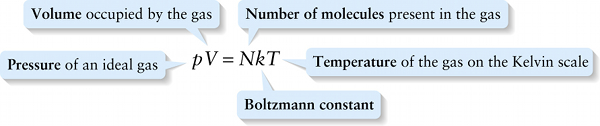
The constant k in Equation 14-6, called the \(\textbf{Boltzmann constant}\), turns out to have the same value for all gases. To four significant figures,
\(k = 1.381 \times 10^{-23}\mathrm{J}/\mathrm{K}\)
Equation 14-6 is called the \(\textbf{ideal gas law}\), and a gas that would obey this equation exactly is called an \(\textbf{ideal gas}\). Real gases are \(\textit{no}\)t ideal: They do not obey this equation exactly, especially at high pressures or at temperatures close to the point at which the gases become liquids. At relatively low gas densities, however, the ideal gas law does a good job of representing the relationship between pressure, volume, and temperature for real gases. That’s why an ideal gas is a useful idealization.