Chapter 15. Molar specific heat at constant volume for an ideal gas (15-18)

Question

HIjfMGlAXaXvTHRHz25yWfisPxRe8mAtE0G294LK4yHtdCr02L0MNxWfyNdASVZwN6NfX9zcYyHzf+R9

{"title":"Molar specific heat of an ideal gas at constant pressure","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"rect\",\"coords\":\"1,7,42,51\"},{\"shape\":\"poly\",\"coords\":\"144,22\"}]"} {"title":"Ideal gas constant","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"259,6,297,52\"}]"} {"title":"Molar specific heat of an ideal gas at constant volume","description":"Wrong","type":"incorrect","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"110,6,151,47\"}]"}

Review

We'll user the symbol \(D\) to represent the number of degrees of freedom. Then the average energy per gas molecule is \((D/2)kT\). One mole of the gas contains Avogadro's number of molecules, so the energy per mole is \(N_A(D/2)kT = (D/2)(N_Ak)T = (D/2)RT\) (Again, recall that \(k = R/N_A\), so \(R = N_Ak\).) Then the internal energy of \(n\) moles of ideal gas is

(15-16) \(U = n\left(\frac{D}{2}R\right)T\) (ideal gas, \(D\) degrees of freedom)

If the temperature of the gas changes by \(\Delta{T}\), it follows from Equation 16-16 that the internal energy change is

(15-17) \(\Delta{U} = n\left(\frac{D}{2}R\right)\Delta{T}\) (ideal gas, \(D\) degrees of freedom)

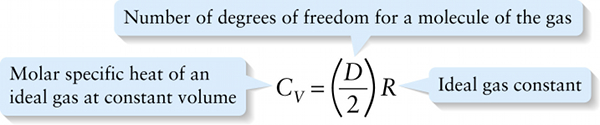
But we saw above that for an ideal gas, \(\Delta{U} = nC_V \Delta{T}\). Comparing this to Equation 15-17, we see that