Electric flux (16-6)
Question 1 of 5
Question
Magnitude of the electric field due to a point charge Q
{"title":"Electric flux through a surface","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"8,2,33,27\"}]"} {"title":"Area of the surface","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"69,1,88,26\"},{\"shape\":\"rect\",\"coords\":\"163,2,184,32\"}]"} {"title":"Magnitude of the electric field","description":"Incorrect","type":"incorrect","color":"#333300","code":"[{\"shape\":\"rect\",\"coords\":\"185,1,209,31\"}]"} {"title":"Angle between the electric field and the perpendicular to the surface","description":"Incorrect","type":"incorrect","color":"#000080","code":"[{\"shape\":\"rect\",\"coords\":\"272,2,290,30\"}]"} {"title":"The component of electric field perpendicular to the surface","description":"Incorrect","type":"incorrect","color":"#008000","code":"[{\"shape\":\"rect\",\"coords\":\"92,1,110,29\"}]"}
Review
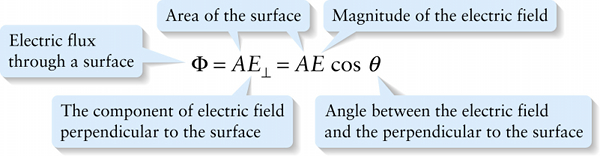
Figure 16-17 shows how we extend the idea of flux to the electric field. Instead of a pipe carrying a fluid, let’s look at a region of space where there is an electric field →E. We saw in Section 16-5 that the value of →E can vary from point to point, so we consider a small enough region that we can treat →E as having essentially the same value over that region. We then imagine a small rectangular area A that we can orient however we like. By analogy to the flux of water in Figure 16-16, we define the electric flux \boldsymbol{\Phi} (the upper-case Greek letter phi) through the area A as follows:
