Chapter 17. Equivalent capacitance of capacitors in parallel (17-26)

Question

WTvClGg/dPpC3+qgoDVDgeopymQfpYE22n6E5L8DTgXjiBX/64SBSIYRfLaU5C5OPJf9jg==

{"title":"Equivalent capacitance of capacitors in parallel","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"3,5,24,31\"}]"} {"title":"Capacitances of the individual capacitors","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"119,5,148,30\"},{\"shape\":\"rect\",\"coords\":\"189,5,218,31\"},{\"shape\":\"rect\",\"coords\":\"263,4,289,31\"}]"}

Question

h/getXxJJhxsCNm+Q+kKjeGvS5C5T/6G3hln2X+REMwaxTyi+BLDbn+ldmdxZ7b5

{"title":"Equivalent capacitance of capacitors in parallel","description":"Incorect","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"3,5,24,31\"}]"} {"title":"Capacitances of the individual capacitors","description":"Correct!","type":"correct","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"119,5,148,30\"},{\"shape\":\"rect\",\"coords\":\"189,5,218,31\"},{\"shape\":\"rect\",\"coords\":\"263,4,289,31\"}]"}

Review

For the equivalent capacitor of capacitance \(C_{\mathrm{equiv}}\) that corresponds to the three capacitors in parallel, Equation 17-14 says that \(q = C_{\mathrm{equiv}}V\). Comparing this to Equation 17-25 shows that

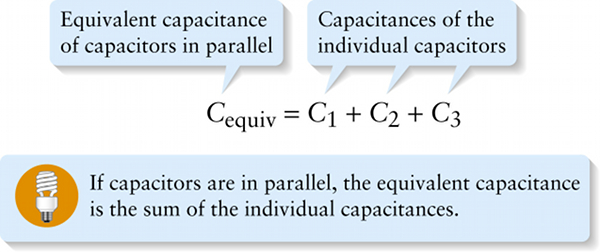
If there are more than three capacitors in parallel, the same rule given in Equation 17-26 applies. The equivalent capacitance of a parallel combination is always greater than the smallest capacitance of any of the individual capacitors.