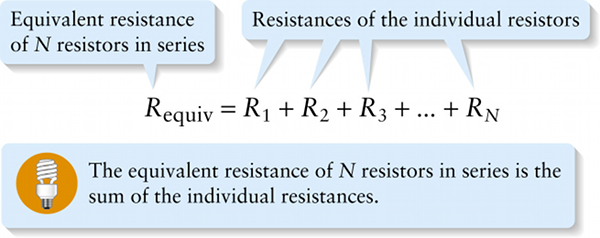
Equivalent resistance of resistors in series (18-11)

Question 1 of 2

Question

Resistances of the individual resistors

{"title":"Equivalent resistance of N resistors in series","description":"Wrong","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"1,2,16,24\"}]"} {"title":"Resistances of the individual resistors","description":"Correct!","type":"correct","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"83,1,100,25\"},{\"shape\":\"rect\",\"coords\":\"132,1,150,24\"},{\"shape\":\"rect\",\"coords\":\"180,2,199,24\"},{\"shape\":\"rect\",\"coords\":\"270,1,287,26\"}]"}

Question

Equivalent resistance of $N$ resistors in series

{"title":"Equivalent resistance of N resistors in series","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"1,2,16,24\"}]"} {"title":"Resistances of the individual resistors","description":"Wrong","type":"incorrect","color":"#ffcc00","code":"[{\"shape\":\"rect\",\"coords\":\"83,1,100,25\"},{\"shape\":\"rect\",\"coords\":\"132,1,150,24\"},{\"shape\":\"rect\",\"coords\":\"180,2,199,24\"},{\"shape\":\"rect\",\"coords\":\"270,1,287,26\"}]"}

Review

Equation 18-11 tells us that by combining resistors in series, we create a circuit with a higher equivalent resistance than that of any of the individual resistors. A special case is when we put two identical resistors $R$ in series. The equivalent resistance is

$R_{\mathrm{equiv}} = R + R = 2R$

The equivalent resistance of two identical resistors in series is twice that of each indi vidual resistor.