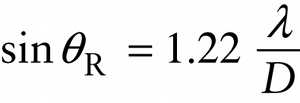Rayleigh’s criterion for resolvability (23-26)

Balloon Art Concept Check: Click on the part of the equation that is described by the bubble text shown

Question 1 of 3

Question

Angle between two pointlike objects that can barely be resolved through an optical device
Feedback
{"title":"Angle between two pointlike objects that can barely be resolved through an optical device","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"59,29,89,65\"}]"} {"title":"Wavelength of the light","description":"Incorrect","type":"incorrect","color":"#008000","code":"[{\"shape\":\"rect\",\"coords\":\"265,0,297,43\"}]"} {"title":"Diameter of the circular aperture of the device","description":"Incorrect","type":"incorrect","color":"#000080","code":"[{\"shape\":\"rect\",\"coords\":\"257,60,298,96\"}]"}

Review

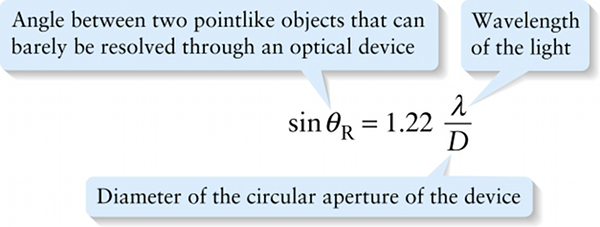
The nineteenth-century English physicist John William Strutt, 3rd Baron Rayleigh, proposed the following criterion for resolvability: Two distant, pointlike objects observed through a circular aperture can be resolved when the central maximum of one coincides with the center of the first dark fringe of the other. The angle \(\theta_{\mathrm{R}}\)that separates two point objects that are just barely resolved through a circular aperture, known as the angular resolution of the aperture, is then just the angle given by Equation 23-25: