Chapter 25. Galilean velocity transformation (25-5)
Question
diIdpQmtAM0w2uF8EY2VMI3CWYSYa44uFALLw0ARj8O9anG5SpELfH3GYxL1hIR6APuuHEIFmSxM9uTgPmnhgo9RSLIUeswZ9/4TUY1jNVny8WaG/yNb63YT8V3vKaWYUiP9Go0VbVx3FQRfzoj9ueooXncbpECBiePjVORWrq3va5b07LblK95iSWgn8/REVMyhN686QFVa8/WVY6XTCp2VGE7vFsyUjLKwWw==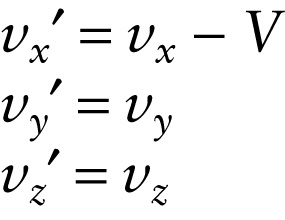
Question
BA8DCpSX37frJbqvz5LXANwhk1V2qynjjmgUO7Soivcid11rldpPU6B17nEhdb5PoszQbYjoD3KsOPqhJhPdqVenZvlIVm7J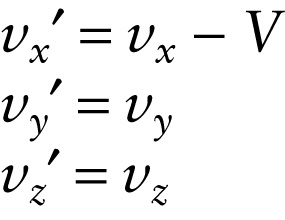
Question
D+WOhs0zZ/8KaB78taACBJjkVlOhKblz9m8z5ibEM/k473feOiMOteG/aeILoA4caSD9G+gORjBdxg6kl81w3/W1s6Y=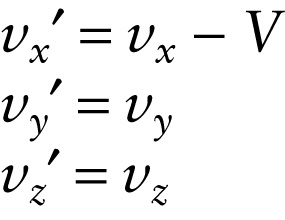
Review
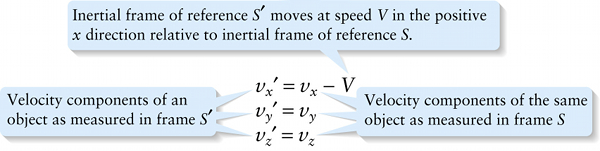
Equations 25-5, which relate the velocity of an object in frame \(S'\) to the velocity of the same object in frame \(S\), are called the Galilean velocity transformation.
As an example, think again of the ball shown in Figure 25-5. If the ball moves straight up and down as measured in frame \(S'\), then in that frame the ball is in free fall with only a \(y\)component of velocity. The other two components The other two components are zero: \(v_x' = v_z' = 0\). As measured in frame \(S\), the velocity of the ball has component
\(\begin{eqnarray*} v_x &=& v_x' + V = V \\ v_y &=& v_y'\\ v_z &=& v_z' = 0 \end{eqnarray*}\)
As measured in frame \(S\), the ball moves up and down along the \(y\) direction with the same velocity as measured in frame \(S': v_y = v_y'\). At the same time, as measured in frame \(S\) the ball maintains a constant velocity \(V\) in the \(x\) direction. That’s just the behavior we expect for a projectile: Its motion is a combination of up-and-down free fall and constant-velocity horizontal motion (see Section 3-6).