Chapter 25. Relativistic gamma (25-19)

Question

AP/4VBL1oTcQkM9xjXU2rfhd11FldcYf7akrg2wBkKlhw8tkjjMjElzHbRUSnb0g1FM/NGyX1rr7gmdkzXJgf+RNrlxf+kyrk3v+fg==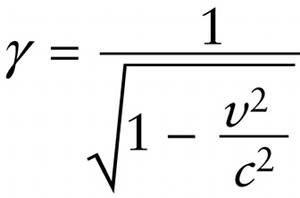

{"title":"Relativistic gamma for a particle moving at speed v","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"4,36,36,85\"}]"} {"title":"Speed of the particle","description":"Incorrect","type":"incorrect","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"221,99,251,131\"}]"} {"title":"Speed of light in a vacuum","description":"Incorrect","type":"incorrect","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"234,161,261,191\"}]"}

Question

YP02Jindd9O7tb1I67tUvxgE7lHHO1/1oDy2XCrVnxC0x+AE1DGGKYdQzMk=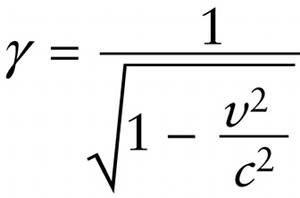

{"title":"Relativistic gamma for a particle moving at speed v","description":"Wrong","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"4,36,36,85\"}]"} {"title":"Speed of the particle","description":"Correct!","type":"correct","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"221,99,251,131\"}]"} {"title":"Speed of light in a vacuum","description":"Incorrect","type":"incorrect","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"234,161,261,191\"}]"}

Question

LKsWMxFHj37AMB3BUFJ6bTf1++1gh7A7XkYTYMO47/LNXVfY6VCFXSpXwPpfuBuZ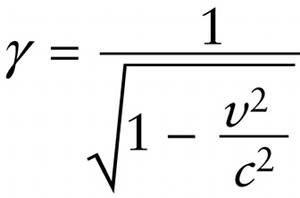

{"title":"Relativistic gamma for a particle moving at speed v","description":"Wrong","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"4,36,36,85\"}]"} {"title":"Speed of the particle","description":"Incorrect","type":"incorrect","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"221,99,251,131\"}]"} {"title":"Speed of light in a vacuum","description":"Correct!","type":"correct","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"234,161,261,191\"}]"}

Review

According to this expression, the maximum magnitude of momentum that an object of mass \(m\) can have is \(p = mc\), which would be attained only when an object is moving at the speed of light. This directly contradicts the notion that there should be no upper limit on momentum. Just as for kinetic energy, we need a new expression for momentum that’s consistent with the special theory of relativity.

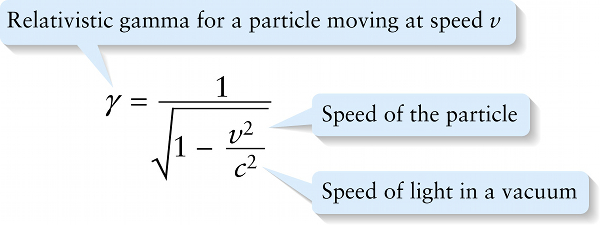
It’s possible to derive the correct expressions for \(K\) and \(\vec{p}\) by looking closely at what happens in an elastic collision, in which both mechanical energy and momentum are conserved (see Section 7-5). The derivation is beyond our scope, so we’ll just look at the results. For a particle moving with velocity \(\vec{v}\), both the expression for the kinetic energy and the expression for the momentum involve a quantity called relativistic gamma: