Chapter 28. Heisenberg uncertainty principle (28-7)

Question

qqiJuF+MUJKXFFSUdDgd1uWJPqUuwq6EVn5j0EkAQ1sY3rpdpriUX7dyiFaHzHEhh4/po+wsed+75sl5t4iZRq9LZvVOp52zSH2KOuXY5YmZ3A67

{"title":"The energy of a phenomenon is necessarily uncertain by an amount ΔE.","description":"Correct!","type":"correct","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"35,35,76,85\"}]"} {"title":"hbar = h over 2 pi = Planck’s constant divided by 2 pi","description":"Incorrect","type":"incorrect","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"198,2,240,51\"}]"} {"title":"The shorter the duration Δt of the phenomenon, the greater the energy uncertainty ΔE.","description":"Incorrect","type":"incorrect","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"107,45,135,84\"}]"}

Question

3a9ZiYjYsEP20DB5oe28lG7zr/uBPuRO6rioWRbE0MRVRp5b6+ZKTMhZBs6MvRUSyMFFarqnGtrvQg1ifo2j6PAiwYIFFL+UVCqjGw==

{"title":"The energy of a phenomenon is necessarily uncertain by an amount ΔE.","description":"Wrong","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"35,35,76,85\"}]"} {"title":"hbar = h over 2 pi = Planck’s constant divided by 2 pi","description":"Correct!","type":"correct","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"198,2,240,51\"}]"} {"title":"The shorter the duration Δt of the phenomenon, the greater the energy uncertainty ΔE.","description":"Incorrect","type":"incorrect","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"107,45,135,84\"}]"}

Question

wNumgJRLXZPL1slFjGRrkL405Xt20t+81WZAcmPjRsoyN7C7chY6UKYDzOnWWuFIKasSZ0NT8Y8BehDmKG7Yn7t48Sa2M2JhPhyASOeGALqyA4xCHx7WwQ3RK17mk64hqbESb6VixEKnh5+81Aborw==

{"title":"The energy of a phenomenon is necessarily uncertain by an amount ΔE.","description":"Wrong","type":"incorrect","color":"#99CCFF","code":"[{\"shape\":\"poly\",\"coords\":\"82,133\"},{\"shape\":\"rect\",\"coords\":\"10,16,12,16\"},{\"shape\":\"poly\",\"coords\":\"144,22\"},{\"shape\":\"rect\",\"coords\":\"35,35,76,85\"}]"} {"title":"hbar = h over 2 pi = Planck’s constant divided by 2 pi","description":"Incorrect","type":"incorrect","color":"#ffff00","code":"[{\"shape\":\"rect\",\"coords\":\"198,2,240,51\"}]"} {"title":"The shorter the duration Δt of the phenomenon, the greater the energy uncertainty ΔE.","description":"Correct!","type":"correct","color":"#00ff00","code":"[{\"shape\":\"rect\",\"coords\":\"107,45,135,84\"}]"}

Review

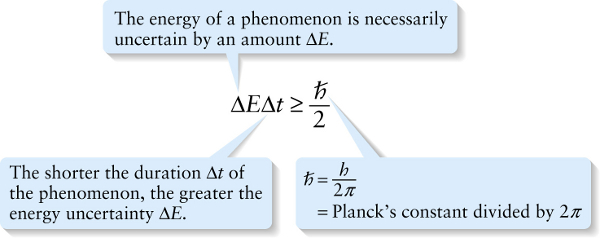
Another way to interpret Equation 28-7 is to say that it places limits on how precisely the law of conservation of energy must be obeyed. Suppose a system undergoes some kind of process that lasts for a time \(\Delta{t}\). Equation 28-7 says that the energy of the system is necessarily uncertain during that process, and the minimum energy uncertainty is given by \(\Delta{E} \Delta{t} = \hbar / 2\) or \(\Delta{E} = \hbar / (2 \Delta{t})\). So it’s fundamentally impossible to measure the energy of the system with an uncertainty less than \(\hbar / (2 \Delta{t})\). This means that during the process, the energy of the system could actually vary by as much as \(\hbar / (2 \Delta{t})\), and there would be no way that we could tell that the energy had changed value—that is, that the energy was not conserved. The shorter the duration \(\Delta{t}\) of the process, the greater the amount \(\hbar / (2 \Delta{t})\)by which energy conservation can be (temporarily) violated during that time \(\Delta{t}\). Stated another way, it’s acceptable to violate the law of conservation of energy by an amount \(\Delta{E}\), provided the duration of time during which the law is violated is no more than \(\Delta{t} = \hbar / (2 \Delta{E})\).