LESSON 64: STP: The Mole and Avogadro’s Law
331
THINK ABOUT IT
Suppose you have two balloons, one filled with helium and the other with carbon dioxide. The pressure, temperature, and volume of the two gases are identical. However, the masses of the balloons are different. While the helium balloon floats, the carbon dioxide balloon sinks. How can you figure out the number of gas particles in each balloon?
How do chemists keep track of the number of gas particles?
To begin to answer this question, you will explore
Counting Gas Particles
Avogadro’s Law
Counting Gas Particles
EXPLORING THE TOPIC
Counting Gas Particles
SPORTS CONNECTION
SPORTS
CONNECTION
A hot air balloon is not a closed container. The flame from a propane burner heats the air inside the balloon. As the gas is heated, it expands and some moles of gas molecules escape through the opening at the base. This decreases the number density, n/V, and the mass density, m/V, of the gas inside the balloon. The lower mass density of the captured gas causes the balloon to rise.

If you take two air samples of identical volume in the same room, you might predict that both samples will contain the same number of air molecules. A smaller air sample from this room will contain fewer air molecules than a larger air sample.
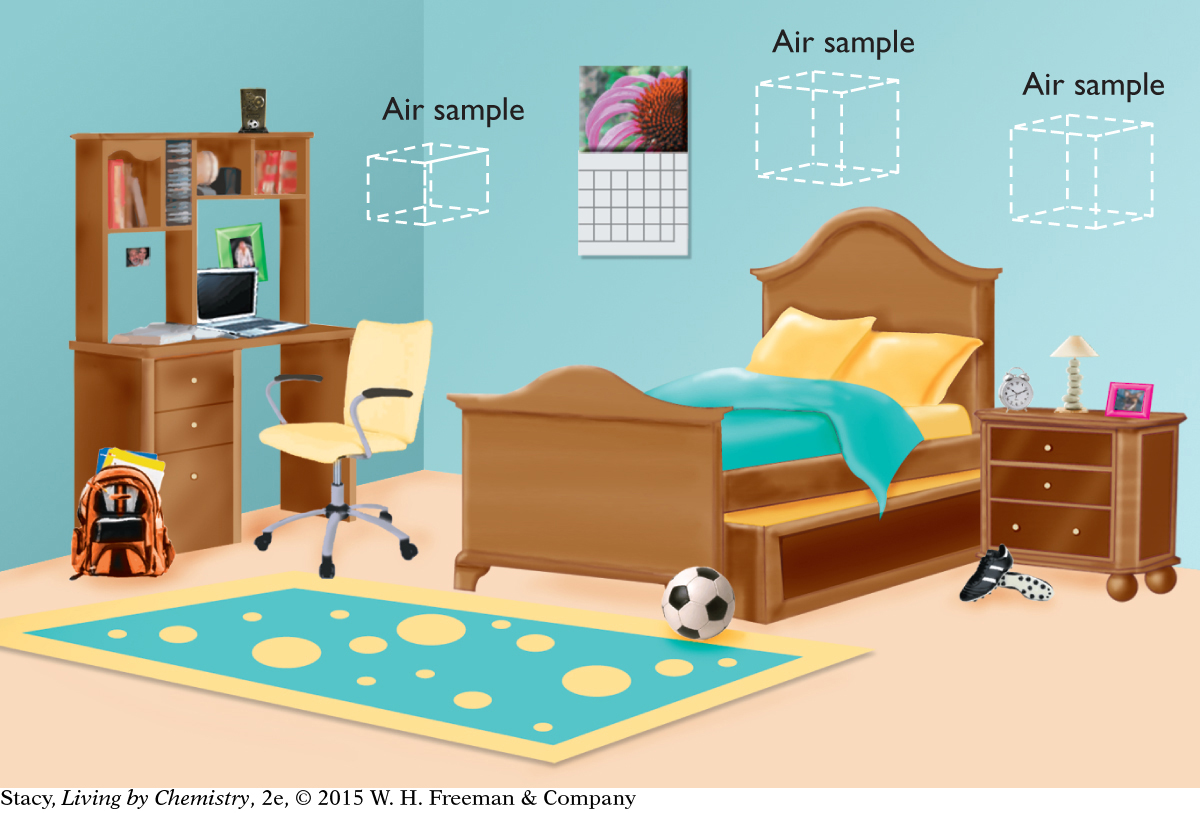
Because the air molecules are spread uniformly over the space the gas occupies, the number of air molecules, n, is proportional to the volume, V, the gas occupies.
332
A MOLE OF GAS PARTICLES
Gas particles are far too numerous to count individually. So chemists have defined a counting unit for small particles called a mole. The abbreviation for mole is mol. A mole represents a very large number of gas particles. A mole is equal to 602,000,000,000,000,000,000,000 particles. This is 602 sextillion! This number is also called Avogadro’s number, after Amedeo Avogadro, a 19th century Italian scientist.
The number of gas particles in a sample is referred to in terms of moles of gas. Keep in mind that when referring to 1 mole, 2 moles, or half a mole of gas particles, these quantities all refer to very large numbers. For example, 2 moles of gas particles is 1,204,000,000,000,000,000,000,000 particles (1,204 sextillion). Half a mole of gas particles is 301,000,000,000,000,000,000,000 (301 sextillion).
STANDARD TEMPERATURE AND PRESSURE, STP
Chemists have figured out how to calculate the number of gas particles in a sample of gas if they know the temperature, volume, and pressure of the sample. However, when comparing gases, it is convenient to define just one set of conditions. Chemists have chosen a gas pressure of 1 atm and a gas temperature of 273 K as the conditions for comparing gases. These conditions are called standard temperature and pressure, or STP. At STP, 1 mol of gas particles occupies a volume of 22.4 L.
Example 1
Two Moles of Air Molecules
Suppose you want to collect 2.0 mol of air molecules at STP. What size box do you need?
Solution
Because 1 mol of air molecules has a volume of 22.4 L at STP, 2 mol has a volume of 44.8 L. So, you need a box with a volume of 44.8 L.
Big Idea
Big Idea
The mole is a counting unit. One mole of gas particles at standard temperature and pressure occupies a volume of 22.4 liters.
Avogadro’s Law
Avogadro’s Law
Several cylinders each contain different gas samples at STP. The data for each cylinder is shown in the table on the next page. Notice that the volumes of the samples vary.
A few molecules (or atoms, in the case of helium and neon) are drawn in the table to help you visualize the gas. Obviously, only a few gas particles are drawn because it is impossible to draw 602 sextillion. However, the numbers of molecules and atoms are in correct proportion to each other so that you can make comparisons. Take a moment to look for patterns.
Notice that the mass of the gas sample depends on the identity of the gas. A hydrogen molecule has a mass of 2.0 amu, while each neon atom has a mass of 20 amu. So 0.25 mol of neon gas has ten times the mass of 0.25 mol of hydrogen gas.
333
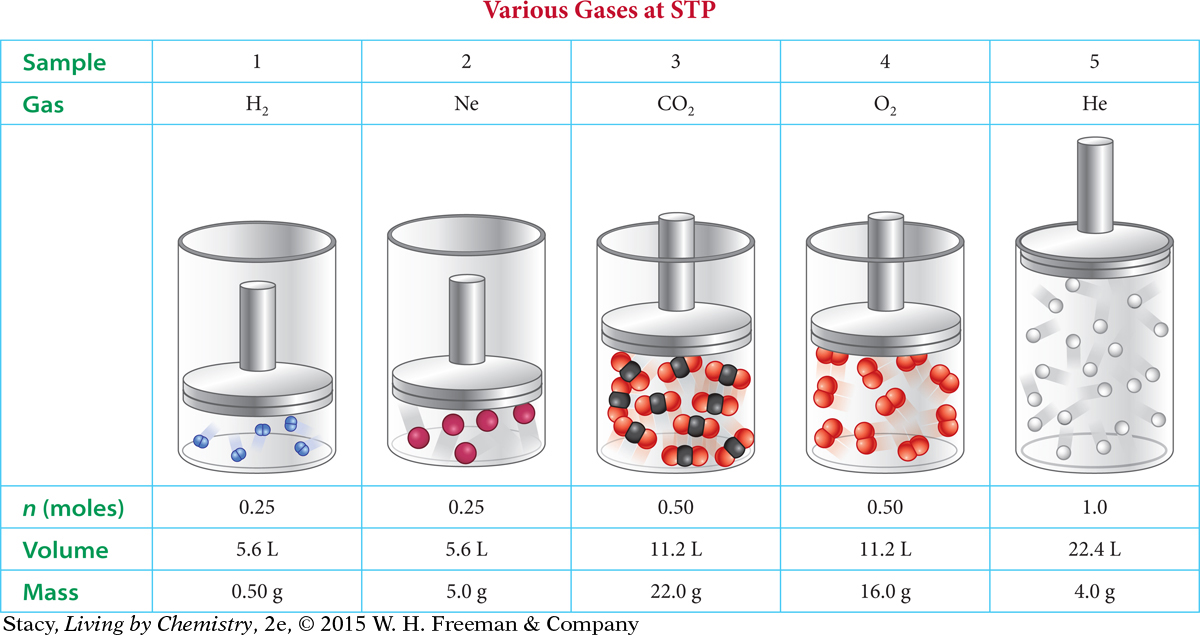
The volume of each cylinder is determined by the number of gas particles. For example, a 22.4-liter sample at STP always has twice as many gas particles as an 11.2-liter sample, regardless of the identity of the gas.
If two gases have the same pressure, volume, and temperature, then they have the same number of gas particles independent of the identity of the gases. This generalization is known as Avogadro’s law. Avogadro’s law is extremely useful. Amedeo Avogadro first proposed this hypothesis in 1811. The reverse is also true: If two gas samples with the same pressure and temperature have the same number of particles, then they occupy the same volume.
Big Idea
Big Idea
If two gas samples have the same pressure, temperature, and volume, they contain the same number of particles, even if you are comparing two different types of gases.
Example 2
Helium and Carbon Dioxide Balloons
Suppose you have two balloons, one filled with helium and the other with carbon dioxide. The pressure, temperature, and volume of the two gases are identical.
Why is the mass of the carbon dioxide balloon greater?
What do you know about the number of atoms in the balloons?
Solution
Even though the number of CO2 molecules is identical to the number of He atoms, the mass of the carbon dioxide balloon is greater because individual molecules of carbon dioxide, CO2, have a greater mass than atoms of helium, He.
There are more atoms in the CO2 balloon. The number of molecules of CO2 is equal to the number of atoms of He according to Avogadro’s law. Because each molecule of CO2 consists of three atoms, there are three times as many atoms in the CO2 balloon than in the He balloon.
334
LESSON SUMMARY
LESSON SUMMARY
How do chemists keep track of the number of gas particles?
KEY TERMS
mole
Avogadro’s number
standard temperature and pressure (STP)
Avogadro’s law
Gas particles move randomly and are distributed uniformly in the space they occupy. At standard temperature and pressure, STP, the gas pressure is 1.0 atm and the temperature is 273 K. At STP in a volume of 22.4 L, there is 1 mol of gas particles. One mole represents 602,000,000,000,000,000,000,000 particles. This is 602 sextillion, also called Avogadro’s number. Avogadro’s law states that equal volumes of any gas at the same pressure and temperature have the same number of gas particles, independent of the identity of the gas.
Exercises
Reading Questions
Explain why chemists invented the unit called a mole.
Explain Avogadro’s law.
Reason and Apply
Suppose you have 22.4 L of the following gases at STP: neon, Ne, argon, Ar, and xenon, Xe.
How many atoms are there in each gas sample?
What is the number density of atoms, n/V, for each sample?
Which sample has the largest mass? Explain your reasoning.
Which sample has the largest mass density, m/V?
Suppose you have 22.4 L of the following gases at STP: hydrogen, H2, nitrogen, N2, and carbon dioxide, CO2.
How many molecules are there in each gas sample?
What is the number density of molecules, n/V, for each sample?
Which sample has the largest mass? Explain your reasoning.
Which sample has the largest number of atoms? Explain your reasoning.
Which sample has the largest mass density, m/V?
Which has more atoms: 8.0 g of helium, He, or 40.0 g of argon, Ar? Explain your reasoning.
Which has more particles: a balloon filled with 10 L of oxygen, O2, gas, or a balloon filled with 15 L of hydrogen, H2, gas? Explain your reasoning. Assume STP.
At 25 °C, which balloon has the greater volume: an oxygen, O2, balloon at 1.2 atm with a mass of 16.0 g, or a helium, He, balloon at 1.2 atm with a mass of 2.0 g? Explain your reasoning.