LESSON 92: Mole Tunnel

LESSON 92
Mole Tunnel
Stoichiometry

THINK ABOUT IT

467

Sales of medications bring in billions of dollars per year. Most of these medications are produced through chemical reactions that are performed in a laboratory. In manufacturing the medications, it is important to know exactly how much reactant you need to prepare a specified mass of product. The balanced chemical equation for the reaction serves as a guide. However, the calculation requires conversion from mass to moles and back to mass.

How do you convert between grams and moles to determine the mass of product?

To answer this question, you will explore

Gram-Mole Conversions

Gram-Mole Conversions

CONSUMER CONNECTION

ENVIRONMENTAL

CONNECTION

Aluminum chloride, AlCl3, and other aluminum compounds are used in antiperspirants to control underarm wetness. When the aluminum ions enter the skin cells, they cause more water to pass into the cells. The cells swell and squeeze the sweat ducts closed, trapping the sweat inside the sweat glands.

image
GVictoria/Shutterstock

EXPLORING THE TOPIC

One type of medication available is an antacid made of aluminum hydroxide, Al(OH)3. It neutralizes excess acid in the stomach. To make aluminum hydroxide, many manufacturers start with aluminum chloride, which is commercially available in large quantities. When solutions of aluminum chloride are mixed with sodium hydroxide, a precipitate of aluminum hydroxide is formed. The balanced chemical equation for the reaction is

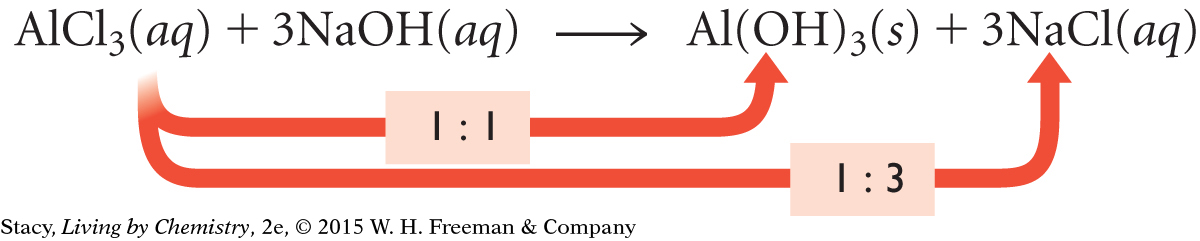
AlCl3(aq) + 3NaOH(aq) → Al(OH)3(s) + 3NaCl(aq)

The coefficients in the equation indicate how many moles to mix together. But in the laboratory, reactants are measured in grams, kilograms, or pounds.

GOING THROUGH THE MOLE TUNNEL

A chemical equation is like a recipe for making a product. It indicates how much of the reactants to mix. If you want to make aluminum hydroxide, Al(OH)3, you need one formula unit of aluminum chloride, AlCl3, for every three formula units of sodium hydroxide, NaOH. Before the recipe can be put into practice, it must be converted to mass. This is because in the laboratory, substances are weighed, not counted.

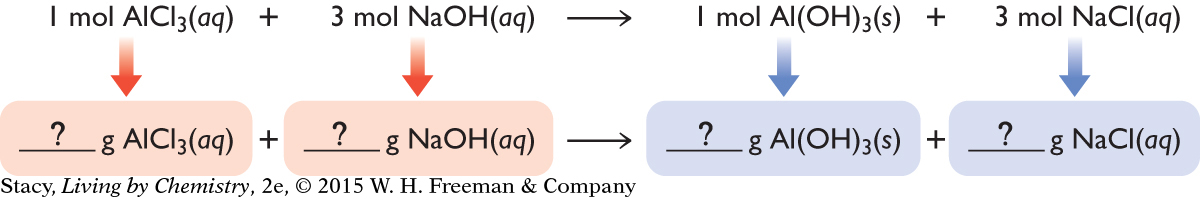
image

If you know the mass of reactants, you can determine the mass of products that can be made.

468

This requires a trip through the “mole tunnel.” Starting with grams of reactant and the balanced chemical equation, there are three steps you must take to convert from grams of reactants to grams of products.

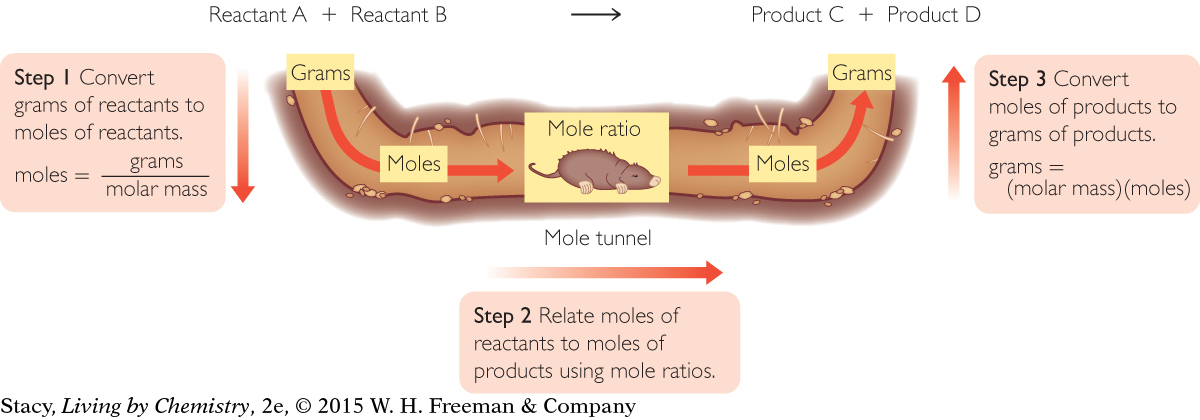
image

To go from one side of the equation to the other, you must go through moles, represented by the tunnel under the equation. The “mole tunnel” in the illustration is one way to remember these steps. These types of calculations, involving quantities of reactants and products, are referred to as gram-mole conversions, or stoichiometry calculations. The word stoichiometry comes from the Greek words stoikheion, meaning “element,” and metrein, meaning “to measure.”

The mole tunnel also works in reverse. If you want a certain mass of a product, you can determine the mass of reactants required.

Solving Stoichiometry Problems

Solving Stoichiometry Problems

HISTORY CONNECTION

HISTORY

CONNECTION

Early black-and-white photographs, called calotypes, were made on paper prepared with silver nitrate. The paper was loaded into a camera under near-total darkness, then exposed to a scene for 10–60 seconds to make a photo negative. Prints could then be produced from the negative. An early photograph of President Abraham Lincoln is shown.

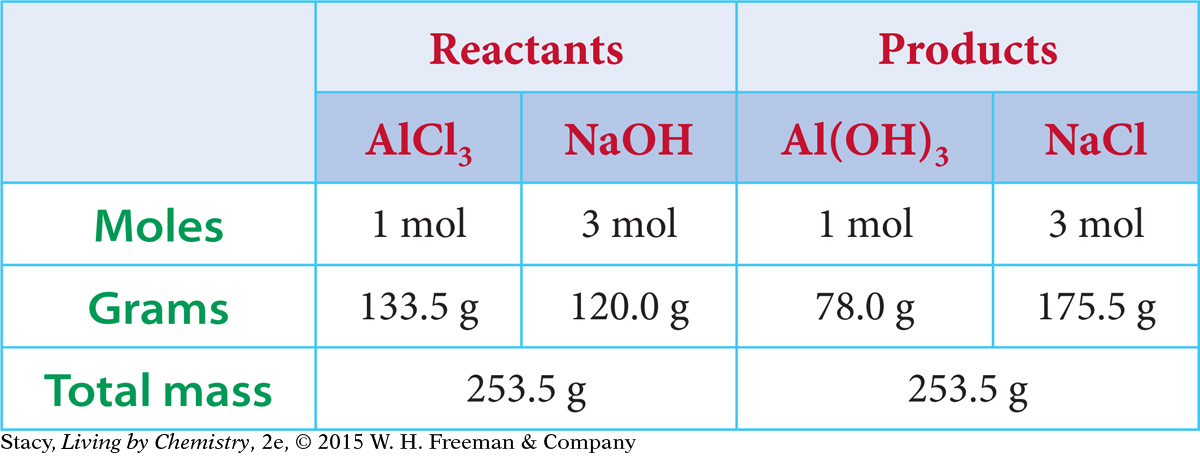
image
© CORBIS

The chemical equation for the formation of aluminum hydroxide, Al(OH)3, shows the reactants combining in a mole ratio of 1:3. The chemical equation also indicates that the mole ratio of the AlCl3 reactant to the Al(OH)3 product is 1:1. So, for every mole of AlCl3 that reacts, one mole of Al(OH)3 is produced. If you want to make 100 mol of Al(OH)3, you will need 100 mol of AlCl3 and 300 mol of NaOH.

The molar masses on the periodic table allow you to convert between grams and moles.

Molar Masses

Reactants: AlCl3 = 133.5 g/mol NaOH = 40.0 g/mol
Products: Al(OH)3 = 78.0 g/mol NaCl = 58.5 g/mol

Next, the mole amounts shown in the chemical equation can be converted to gram amounts. The table summarizes these calculations.

image

469

Example 1

From Moles to Grams

Suppose you have 5.00 mol of AlCl3 in solution and an unlimited supply of NaOH. How many grams of Al(OH)3 and NaCl can you make?

AlCl3(aq) + 3NaOH(aq) → Al(OH)3(s) + 3NaCl(aq)

Solution

Use mole ratios to determine the moles of product you can make.

image

So, with 5.00 mol of AlCl3, you can make 5.00 mol of Al(OH)3 and 15.0 mol of NaCl. Use molar mass to convert these quantities to grams.

Multiply the number of moles by the molar mass. 5.0 mol Al(OH)3 · 75.0 g/mol = 375 g
15.0 mol NaCl · 58.5 g/mol = 878 g

If you start with 5.0 mol AlCl3, you can react it with 15.0 mol NaOH to prepare 375 g Al(OH)3 and 878 g NaCl.

Example 2

From Grams to Grams

Suppose you have 500 g of AlCl3 in solution and an unlimited supply of NaOH. How many grams of each product can you make?

Solution

You cannot convert directly from grams of reactant to grams of product. You must use the “mole tunnel.”

Use molar mass to convert from grams to moles of reactants.

image

Use mole ratios to determine the moles of each product.

So 3.75 mol AlCl3 will make 3.75 mol Al(OH)3 and 11.25 mol NaCl.

Use molar mass to convert between moles and grams.

Multiply the number of moles by the molar mass. 3.75 mol Al(OH)3 · 75.0 g/mol = 281 g Al(OH)3
11.25 mol NaCl · 58.5 g/mol = 658 g NaCl

So, 500 g AlCl3 produces 281 g Al(OH)3 and 658 g NaCl.

LESSON SUMMARY

LESSON SUMMARY

470

KEY TERM

stoichiometry

How do you convert between grams and moles to determine the mass of product?

To determine the mass of product produced by a certain mass of reactant (and vice versa), it is necessary to convert mass to moles and then back to mass. This is because the balanced chemical equation is written in moles. Typically, when you’re working with chemical reactions, you know the grams of reactant you need to produce a certain number of grams of product. Calculations involving mole ratios and masses of reactants and products are referred to as gram-mole conversions, or stoichiometry calculations.

Exercises

Reading Questions

  1. Explain why you need to do gram-mole conversions when carrying out chemical reactions.

Reason and Apply

  1. Consider this balanced chemical equation.

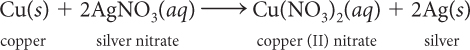
    image

    1. Find the mole ratio of AgNO3 to Cu(NO3)2.

    2. Find the mole ratio of AgNO3 to Ag.

    3. Suppose you have 6.0 mol of Cu. How many moles of AgNO3 are needed to react completely with 6.0 mol of Cu? How many mol of Cu(NO3)2 are produced?

    4. Suppose you want to make 30.0 g Ag. How many moles of Ag is that? How many moles of Cu do you need?

  2. Consider this balanced chemical equation.

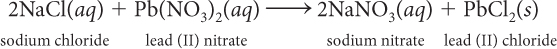
    image

    1. Suppose you want to make 30.0 g PbCl2. How many grams of NaCl do you need?

    2. Suppose you have 56 g Pb(NO3)2. How many grams of NaCl are needed to react completely with 56 g Pb(NO3)2? How many grams of PbCl2 are produced?

  3. An aqueous solution of sodium carbonate reacts with an aqueous solution of calcium sulfate to produce solid calcium carbonate and an aqueous solution of sodium sulfate.

    image

    1. What type of reaction is this?

    2. How many grams of each reactant are needed to produce 4500 g of CaCO3?