LESSON 93: Get the Lead Out
THINK ABOUT IT
471
Imagine you want to make as many cheese sandwiches as possible from a loaf of bread and a large package of sliced cheese. The package of cheese contains 15 slices. The loaf of bread contains 24 slices. Which ingredient will you run out of first, and how many sandwiches can you make?
Which reactant determines how much product you can make?
To answer this question, you will explore
Limiting Reactants
Solving Limiting Reactant Problems
Percent Yield
Limiting Reactants
EXPLORING THE TOPIC
Limiting Reactants
Mixing reactants to form products is a little like making cheese sandwiches from specific quantities of cheese and bread. In the example above, you could make 12 sandwiches before running out of bread. You would have 3 slices of cheese left over. In the world of chemistry, substances rarely come together in the exact mole ratios specified by a chemical equation. One of the reactants usually runs out first. As you learned in Lesson 91: Mole to Mole, the reactant that runs out is called the limiting reactant.
Imagine that you have a beaker with an aqueous solution of sulfuric acid containing a total of 4 mol of H2SO4. You add 112 g of solid potassium hydroxide, KOH. Will the addition of this amount of KOH(s) completely neutralize the H2SO4(aq)? Will either reactant be left over?
The balanced chemical equation for the reaction you are examining is
H2SO4(aq) + 2KOH(s) → K2SO4(aq) + 2H2O(aq)
You must first find out how many moles are represented by 112 g of KOH. The molar mass of KOH is 56 g/mol, so 112 g KOH is equal to 2 mol of KOH.
The chemical equation shows that you will need 2 mol of KOH to neutralize 1 mol of H2SO4. There are 4 mol of H2SO4 in the aqueous solution, and you have only 2 mol of KOH. You will be able to neutralize only 1 mol of H2SO4. There will be 3 mol of H2SO4 left over.
472
The illustration represents what happens in your beaker. Each unit represents 1 mol.
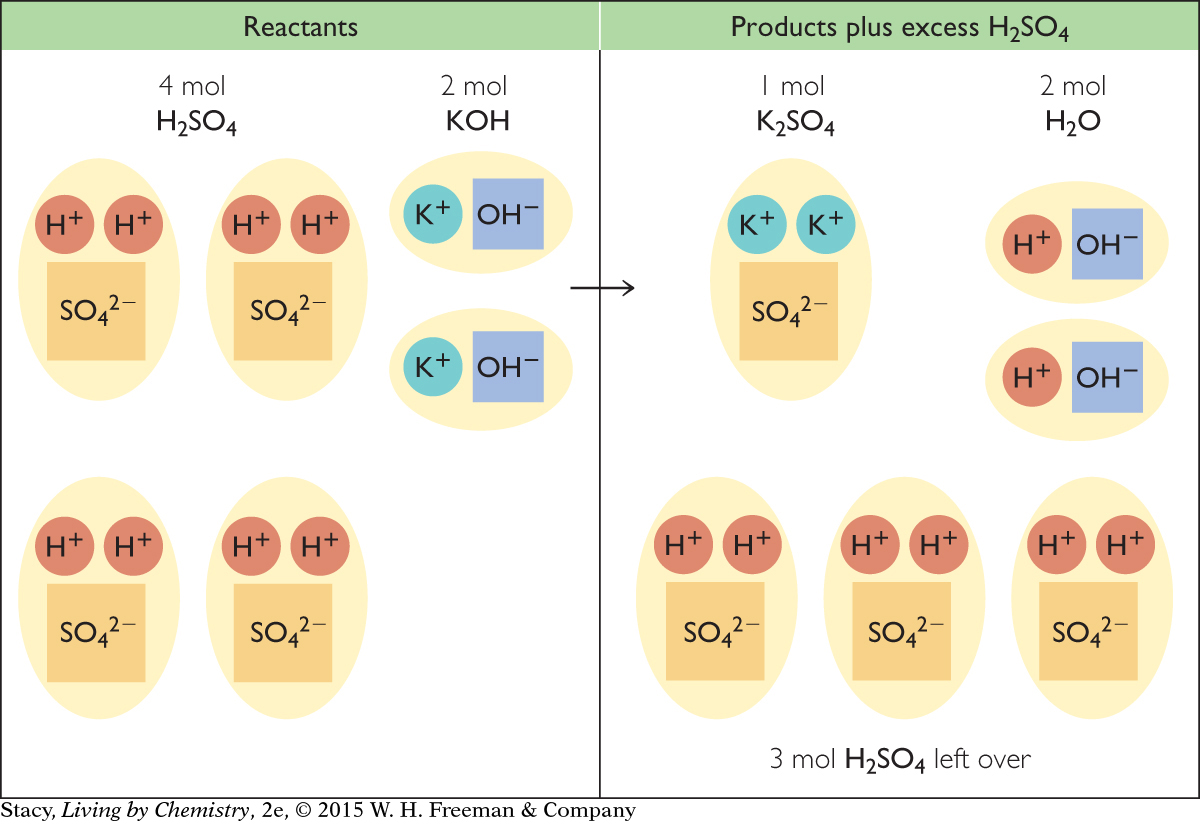
There is not enough KOH to react with all of the H2SO4. KOH is the limiting reactant, and at the end of the reaction, there will be excess H2SO4.
Solving Limiting Reactant Problems
Solving Limiting Reactant Problems
Knowing the identity of the limiting reactant allows you to figure out the maximum amount of product you can make with the substances you have. Mole ratios from the balanced chemical equation are the key to solving limiting reactant problems.
CONSUMER CONNECTION
CONSUMER
CONNECTION
Magnesium chloride, MgCl2, is used in the preparation of tofu from soy milk. When it is added to the soy milk, it causes the tofu to clot up and separate out.

Big Idea
Big Idea
The limiting reactant determines how much product you can make from a chemical reaction.
The steps to complete a limiting reactant problem are shown here.
Solving a Limiting Reactant Problem
Step 1: Write the balanced chemical equation.
Step 2: Determine the molar mass of each compound.
Step 3: Determine the number of moles of each reactant that you have.
Step 4: Use the mole ratio to identify the limiting reactant.
Step 5: Use the limiting reactant to determine the maximum amount of product.
473
Example 1
Preparing Magnesium Chloride
Adding hydrochloric acid, HCl, to magnesium hydroxide, Mg(OH)2, produces magnesium chloride, MgCl2, and water. Imagine you start with 10.0 g of Mg(OH)2 and 100 mL of 4.0 M HCl. How much MgCl2 will be produced?
Solution
Step 1: Write the balanced chemical equation.
Mg(OH)2(s) + 2HCl(aq) → MgCl2(aq) + 2H2O(l)
Step 2: Determine the molar mass of each compound.
| Reactants: Mg(OH)2 = 58.3 g/mol | HCl = 36.5 g/mol |
| Products: MgCl2 = 95.3 g/mol | H2O = 18.0 g/mol |
Step 3: Determine the number of moles of each reactant that you have.
Moles of HCl = (0.100 L)(4.0 mol/L) = 0.40 mol
Step 4: Use the mole ratio to identify the limiting reactant.
The reactants combine in a 1:2 ratio. So you need 0.17 mol · 2 = 0.34 mol of hydrochloric acid, HCl, to react with the 0.17 mol of magnesium hydroxide, Mg(OH)2.
You have 0.40 mol of HCl, so the limiting reactant is Mg(OH)2. When the reaction is complete, there will be 0.06 mol of HCl left over.
Step 5: Use the limiting reactant to determine the maximum amount of product.
For each mole of Mg(OH)2, 1 mol of MgCl2 is produced. So, 0.17 mol of MgCl2 is produced. Use the molar mass to determine the mass of MgCl2.
(0.17 mol) (95.3 g/mol) = 16.2 g MgCl2
So starting with 10.0 g Mg(OH)2 and 100 mL of 4.0 M HCl, you can make 16.2 g MgCl2.
Percent Yield
Percent Yield
Important to Know
The reactant present in the smallest amount is not necessarily the limiting reactant. The mole ratio specified by the coefficients of the balanced equation must be taken into consideration.
When you use the limiting reactant to calculate the amount of a product produced in a chemical reaction, you are calculating the theoretical yield for that product or what you should be able to produce in theory. For a variety of reasons, including experimental error, reactions rarely produce the predicted theoretical yield when run in the laboratory. The amount of a product produced when the reaction is run is called the actual yield for that product and is usually a bit less than the theoretical yield. When you compare the actual yield with the theoretical yield and express it as a percentage, it is called the percent yield.
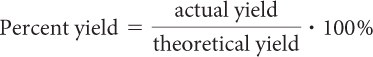
474
The percent yield tells you how successful or efficient your procedure was in the laboratory. The closer your percent yield is to 100%, the more efficient your procedure was.
Example 2
Percent Yield of MgCl2
Suppose that you ran the reaction from Example 1 and 15.4 g of MgCl2 are produced. What is the percent yield of your reaction?
Solution
Calculate the percent yield for the reaction you ran. The theoretical yield was 16.2 g MgCl2.

Your percent yield was very close to the theoretical yield.
LESSON SUMMARY
LESSON SUMMARY
Which reactant determines how much product you can make?
KEY TERMS
theoretical yield
actual yield
percent yield
In the real world, substances rarely come together in the exact mole ratios specified by a chemical equation. Usually, there is more of one reactant than is required and less of the other. If reactants are not present in the exact mole ratio, one reactant, the limiting reactant, will get used up, therefore limiting the amount of product that can be produced. There will be excess of the other reactant. The percent yield of product is the actual amount of product produced in a chemical reaction as compared with the theoretical yield expressed as a percent. The theoretical yield of a chemical reaction is determined by the limiting reagent and the molar ratios in the chemical equation.
INDUSTRY CONNECTION
INDUSTRY
CONNECTION
Pharmaceutical companies can calculate percent yield for each chemical reaction involved in creating a medicine. A low percent yield can be an indication of possible problems with the manufacturing process. Correcting these problems helps the manufacturer to make a safer, better product and to make the most of it at the lowest cost of raw materials.

Exercises
Reading Questions
How can you determine how much product you can make from two compounds?
What is percent yield?
475
Reason and Apply
Consider the reaction to remove mercury from a water source through precipitation:
Hg(NO3)2(aq) + 2NaCl(aq) → HgCl2(s) + 2NaNO3(aq)
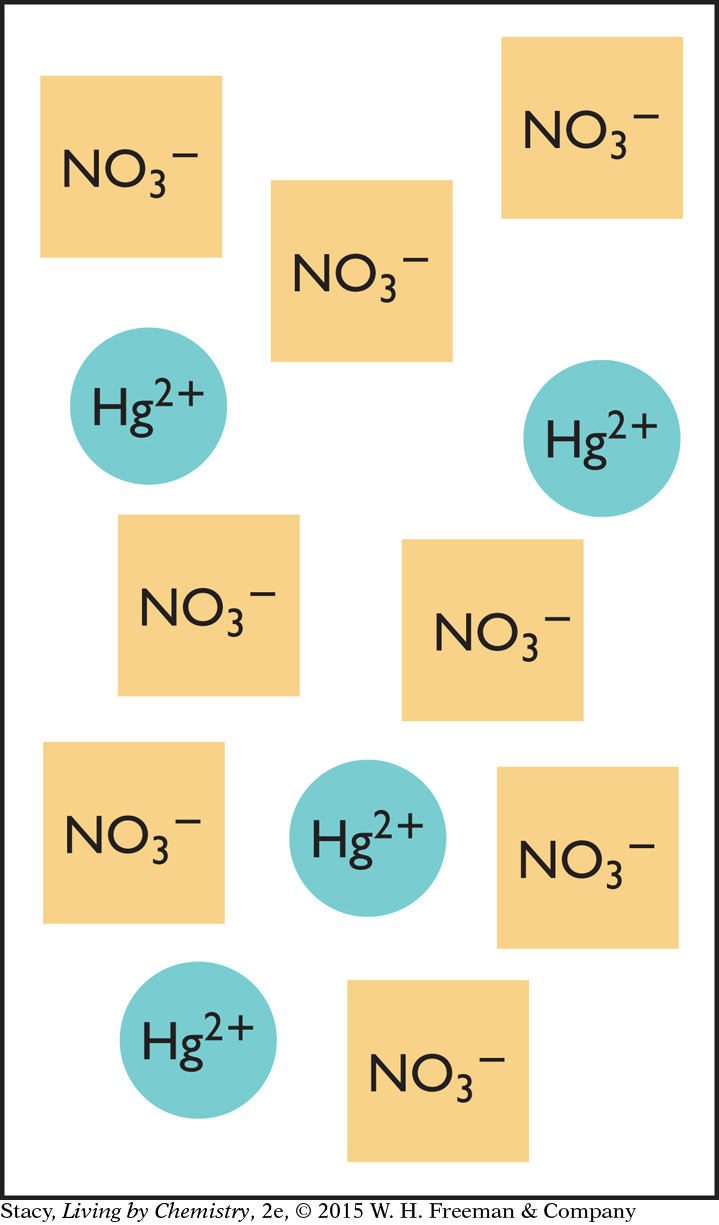
On your paper, draw a diagram that shows the correct amount of sodium chloride needed to react with the amount of mercury (II) nitrate represented in the box on the right.
What else is present in the beaker at the end of the reaction, besides the solid HgCl2?
Why aren’t there any mercury ions left over at the end?
Suppose you were trying to remove 50.3 g of mercury (II) nitrate, Hg(NO3)2, from a water supply using the reaction described in Exercise 3. How many grams of sodium chloride would you need to add?
Silver nitrate, AgNO3 reacts with sodium chloride, NaCl, in aqueous solution to form solid silver chloride, AgCl(s), and aqueous sodium nitrate, NaNO3(aq). Suppose you start with 6.3 g of AgNO3 and 4.5 g of NaCl.
Write a balanced chemical equation for this reaction.
How many moles of each reactant are you starting with?
What is the limiting reactant?
How many grams of each product do you expect to produce?
How many grams of excess reactant do you expect to have when the reaction is complete?
In the laboratory, you run a procedure for the reaction described in Exercise 5 and produce 2.9 g of silver chloride. What is the percent yield for your procedure?