15.2 Solving the Model
We have now looked at each of the pieces of the dynamic AD–AS model. As a quick summary, Table 15-1 lists the equations, variables, and parameters in the model. The variables are grouped according to whether they are endogenous (to be determined by the model) or exogenous (taken as given by the model).

448
The model’s five equations determine the paths of five endogenous variables: output Yt, the real interest rate rt, inflation πt, expected inflation Etπt+1, and the nominal interest rate it. In any period, the five endogenous variables are influenced by the four exogenous variables in the equations as well as the previous period’s inflation rate. Lagged inflation πt−1 is called a predetermined variable. That is, it is a variable that was endogenous in the past but, because it is fixed by the time when we arrive in period t, is essentially exogenous for the purposes of finding the current equilibrium.
449
We are almost ready to put these pieces together to see how various shocks to the economy influence the paths of these variables over time. Before doing so, however, we need to establish the starting point for our analysis: the economy’s long-run equilibrium.
The Long-Run Equilibrium
The long-run equilibrium represents the normal state around which the economy fluctuates. It occurs when there are no shocks (εt = υt = 0) and inflation has stabilized (πt = πt−1).
Straightforward algebra applied to the model’s five equations can be used to determine the long-run values of the five endogenous variables:
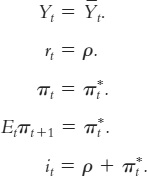
In words, the long-run equilibrium is described as follows: output and the real interest rate are at their natural values, inflation and expected inflation are at the target rate of inflation, and the nominal interest rate equals the natural rate of interest plus target inflation.
The long-run equilibrium of this model reflects two related principles: the classical dichotomy and monetary neutrality. Recall that the classical dichotomy is the separation of real from nominal variables and that monetary neutrality is the property according to which monetary policy does not influence real variables. The equations immediately above show that the central bank’s inflation target 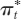 influences only inflation πt, expected inflation Etπt+1, and the nominal interest rate it. If the central bank raises its inflation target, then inflation, expected inflation, and the nominal interest rate all increase by the same amount. Monetary policy does not influence the real variables—output Yt and the real interest rate rt. In these ways, the long-run equilibrium of the dynamic AD–AS model mirrors the classical models we examined in Chapter 3, Chapter 4, Chapter 5, Chapter 6, Chapter 7, Chapter 8, and Chapter 9.
influences only inflation πt, expected inflation Etπt+1, and the nominal interest rate it. If the central bank raises its inflation target, then inflation, expected inflation, and the nominal interest rate all increase by the same amount. Monetary policy does not influence the real variables—output Yt and the real interest rate rt. In these ways, the long-run equilibrium of the dynamic AD–AS model mirrors the classical models we examined in Chapter 3, Chapter 4, Chapter 5, Chapter 6, Chapter 7, Chapter 8, and Chapter 9.
The Dynamic Aggregate Demand Curve
To study the behavior of this economy in the short run, it is useful to analyze the model graphically. Because graphs have two axes, we need to focus on two variables. We will use output Yt and inflation πt as the variables on the two axes because these are the variables of central interest. As in the conventional AD–AS model, output will be on the horizontal axis. But because the price level has now faded into the background, the vertical axis in our graphs will now represent the inflation rate.
450
To generate this graph, we need two equations that summarize the relationships between output Yt and inflation πt. These equations are derived from the five equations of the model we have already seen. To isolate the relationships between Yt and πt, however, we need to use a bit of algebra to eliminate the other three endogenous variables (rt, it, and Etπt+1).
The first relationship between output and inflation comes almost directly from the Phillips curve equation. We can get rid of the one endogenous variable in the equation (Et−1πt) by using the expectations equation (Et−1πt = πt−1) to substitute past inflation πt−1 for expected inflation Et−1πt. With this substitution, the equation for the Phillips curve becomes
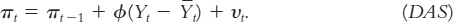
This equation relates inflation πt and output Yt for given values of two exogenous variables (natural output 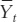 and a supply shock υt) and a predetermined variable (the previous period’s inflation rate πt−1).
and a supply shock υt) and a predetermined variable (the previous period’s inflation rate πt−1).
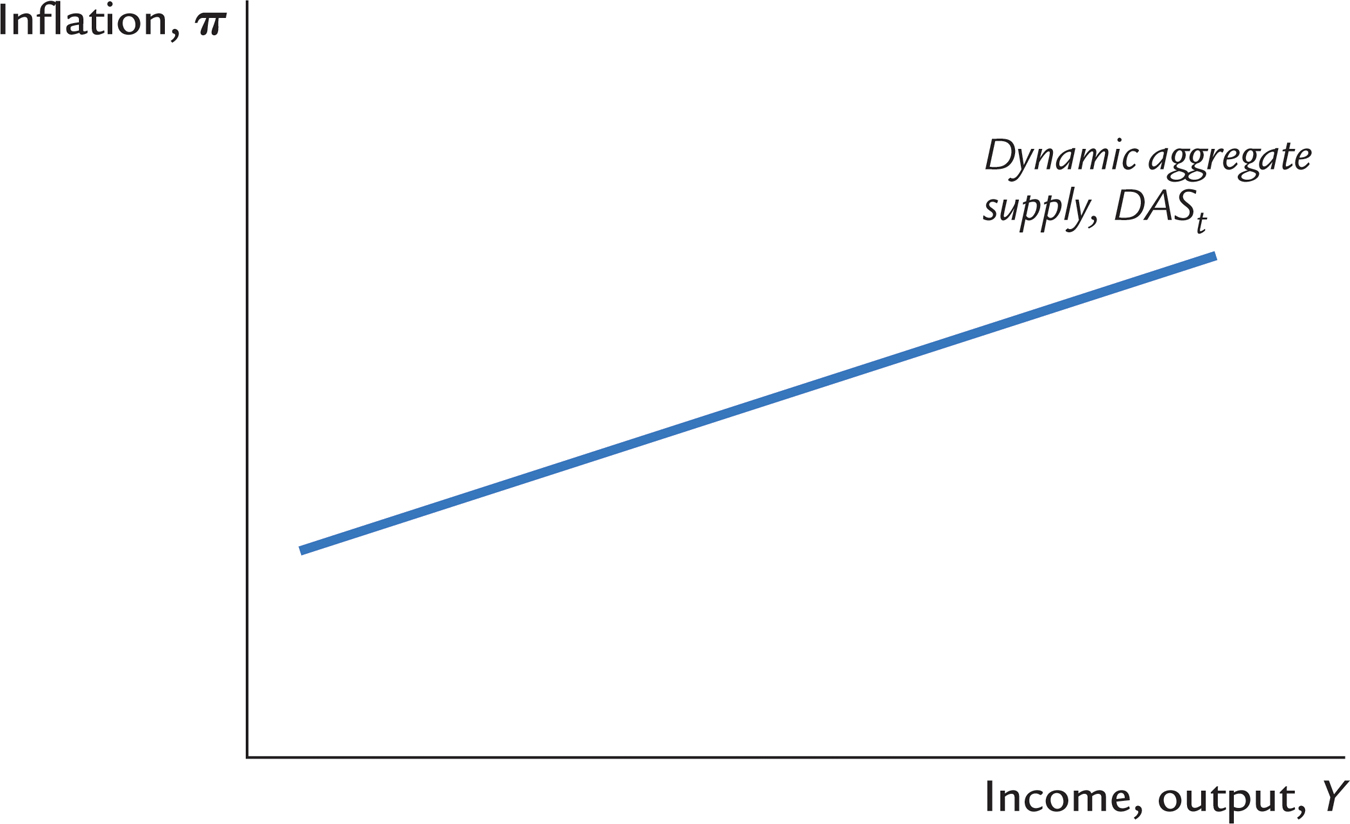
Figure 15-2 graphs the relationship between inflation πt and output Yt described by this equation. We call this upward-sloping curve the dynamic aggregate supply curve, or DAS. The dynamic aggregate supply curve is similar to the aggregate supply curve we saw in Chapter 14, except that inflation rather than the price level is on the vertical axis. The DAS curve shows how inflation is related to output in the short run. Its upward slope reflects the Phillips curve: other things equal, higher levels of economic activity are associated with higher marginal costs of production and, therefore, higher inflation.
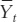 , and the supply shock υt. When these variables change, the curve shifts.
, and the supply shock υt. When these variables change, the curve shifts.
The DAS curve is drawn for given values of past inflation πt−1, the natural level of output 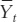 , and the supply shock υt. If any one of these three variables changes, the DAS curve shifts. One of our tasks ahead is to trace out the implications of such shifts. But first, we need another curve.
, and the supply shock υt. If any one of these three variables changes, the DAS curve shifts. One of our tasks ahead is to trace out the implications of such shifts. But first, we need another curve.
451
The Dynamic Aggregate Supply Curve
The dynamic aggregate supply curve is one of the two relationships between output and inflation that determine the economy’s short-run equilibrium. The other relationship is (no surprise) the dynamic aggregate demand curve. We derive it by combining four equations from the model and then eliminating all the endogenous variables other than output and inflation. Once we have an equation with only two endogenous variables (Yt and πt), we can plot the relationship on our two-dimensional graph.
We begin with the demand for goods and services:
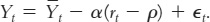
To eliminate the endogenous variable rt, the real interest rate, we use the Fisher equation to substitute it − Etπt+1 for rt:
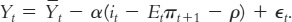
To eliminate another endogenous variable, the nominal interest rate it, we use the monetary-policy equation to substitute for it:
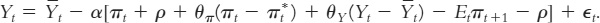
Next, to eliminate the endogenous variable of expected inflation Etπt+1, we use our equation for inflation expectations to substitute πt for Etπt+1:
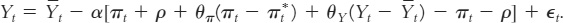
As was our goal, this equation has only two endogenous variables: output Yt and inflation πt. We can now simplify it. Notice that the positive πt and ρ inside the brackets cancel the negative ones. The equation then becomes
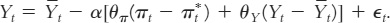
If we now bring like terms together and solve for Yt, we obtain
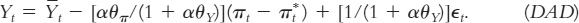
This equation relates output Yt to inflation πt for given values of three exogenous variables (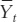 ,
, 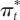 , and εt). In words, it says output equals the natural level of output when inflation is on target
, and εt). In words, it says output equals the natural level of output when inflation is on target 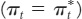 and there is no demand shock (εt = 0). Output rises above its natural level if inflation is below target
and there is no demand shock (εt = 0). Output rises above its natural level if inflation is below target 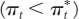 or if the demand shock is positive (εt > 0). Output falls below its natural level if inflation is above target
or if the demand shock is positive (εt > 0). Output falls below its natural level if inflation is above target 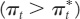 or if the demand shock is negative (εt < 0).
or if the demand shock is negative (εt < 0).
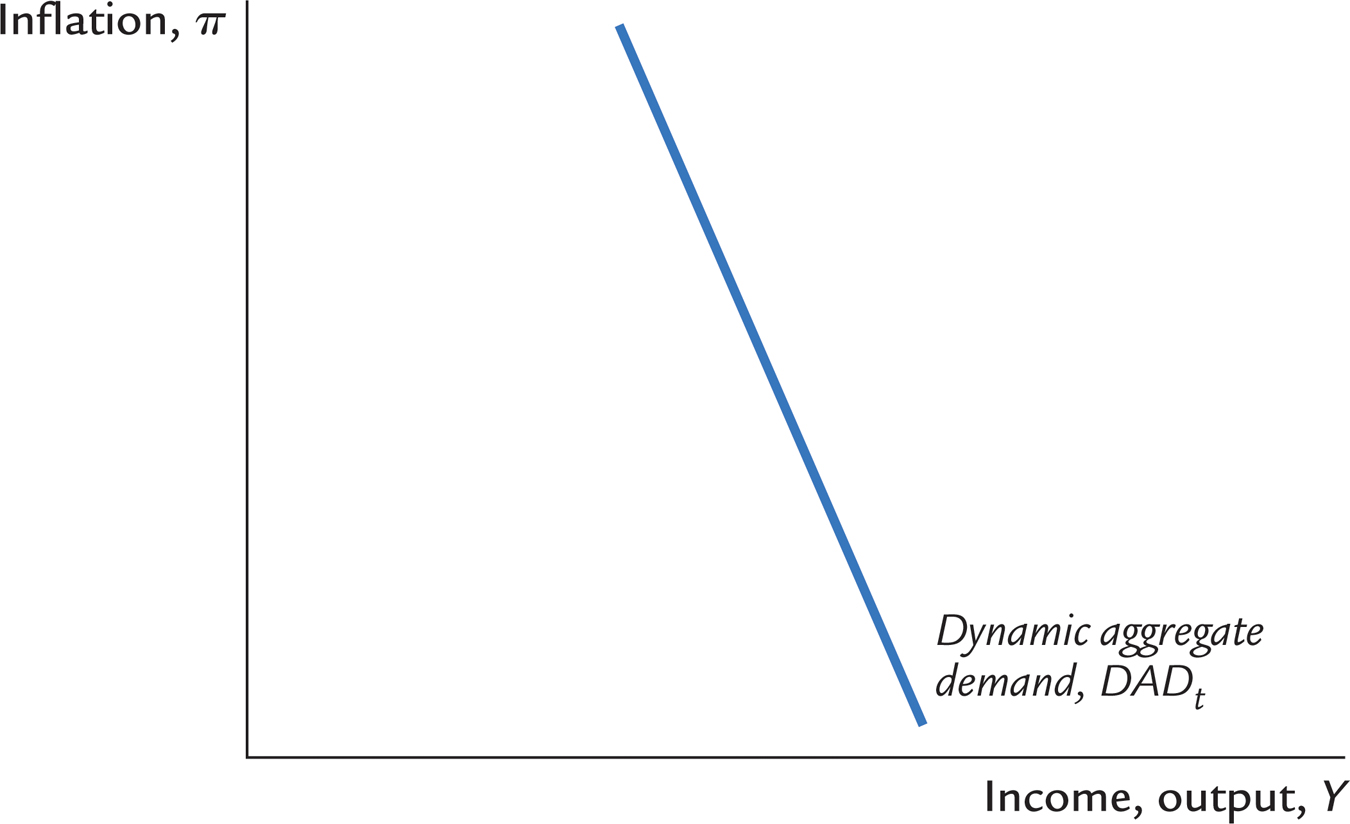
Figure 15-3 graphs the relationship between inflation πt and output Yt described by this equation. We call this downward-sloping curve the dynamic aggregate demand curve, or DAD. The DAD curve shows how the quantity of output demanded is related to inflation in the short run. It is drawn holding constant the exogenous variables in the equation: the natural level of output 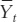 , the inflation target
, the inflation target 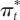 , and the demand shock εt. If any one of these three exogenous variables changes, the DAD curve shifts. We will examine the effect of such shifts shortly.
, and the demand shock εt. If any one of these three exogenous variables changes, the DAD curve shifts. We will examine the effect of such shifts shortly.
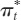 , and the demand shock εt. When these exogenous variables change, the curve shifts.
, and the demand shock εt. When these exogenous variables change, the curve shifts.
452
It is tempting to think of this dynamic aggregate demand curve as nothing more than the standard aggregate demand curve from Chapter 12 with inflation, rather than the price level, on the vertical axis. In some ways, they are similar: they both embody the link between the interest rate and the demand for goods and services. But there is an important difference. The conventional aggregate demand curve in Chapter 12 is drawn for a given money supply. By contrast, because the monetary-policy rule is used to derive the dynamic aggregate demand equation, the dynamic aggregate demand curve is drawn for a given rule for monetary policy. Under that rule, the central bank sets the interest rate based on macroeconomic conditions, and it allows the money supply to adjust accordingly.
The dynamic aggregate demand curve slopes downward because of the following mechanism. When inflation rises, the central bank responds by following its rule and increasing the nominal interest rate. Because the rule specifies that the central bank raise the nominal interest rate by more than the increase in inflation, the real interest rate rises as well. The increase in the real interest rate reduces the quantity of goods and services demanded. This negative association between inflation and quantity demanded, working through central bank policy, makes the dynamic aggregate demand curve slope downward.
The dynamic aggregate demand curve shifts in response to changes in fiscal and monetary policy. As we noted earlier, the shock variable εt reflects changes in government spending and taxes (among other things). Any change in fiscal policy that increases the demand for goods and services means a positive value of εt and a shift of the DAD curve to the right. Any change in fiscal policy that decreases the demand for goods and services means a negative value of εt and a shift of the DAD curve to the left.
Monetary policy enters the dynamic aggregate demand curve through the target inflation rate 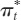 . The DAD equation shows that, other things equal, an increase in
. The DAD equation shows that, other things equal, an increase in 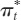 raises the quantity of output demanded. (There are two negative signs in front of
raises the quantity of output demanded. (There are two negative signs in front of 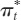 , so the effect is positive.) Here is the mechanism that lies behind this mathematical result: When the central bank raises its target for inflation, it pursues a more expansionary monetary policy by reducing the nominal interest rate, as dictated by the monetary-policy rule. For any given rate of inflation, the lower nominal interest rate in turn means a lower real interest rate, and a lower real interest rate stimulates spending on goods and services. Thus, output is higher for any given inflation rate, so the dynamic aggregate demand curve shifts to the right. Conversely, when the central bank reduces its target for inflation, it raises nominal and real interest rates, thereby dampening demand for goods and services and shifting the dynamic aggregate demand curve to the left.
, so the effect is positive.) Here is the mechanism that lies behind this mathematical result: When the central bank raises its target for inflation, it pursues a more expansionary monetary policy by reducing the nominal interest rate, as dictated by the monetary-policy rule. For any given rate of inflation, the lower nominal interest rate in turn means a lower real interest rate, and a lower real interest rate stimulates spending on goods and services. Thus, output is higher for any given inflation rate, so the dynamic aggregate demand curve shifts to the right. Conversely, when the central bank reduces its target for inflation, it raises nominal and real interest rates, thereby dampening demand for goods and services and shifting the dynamic aggregate demand curve to the left.
453
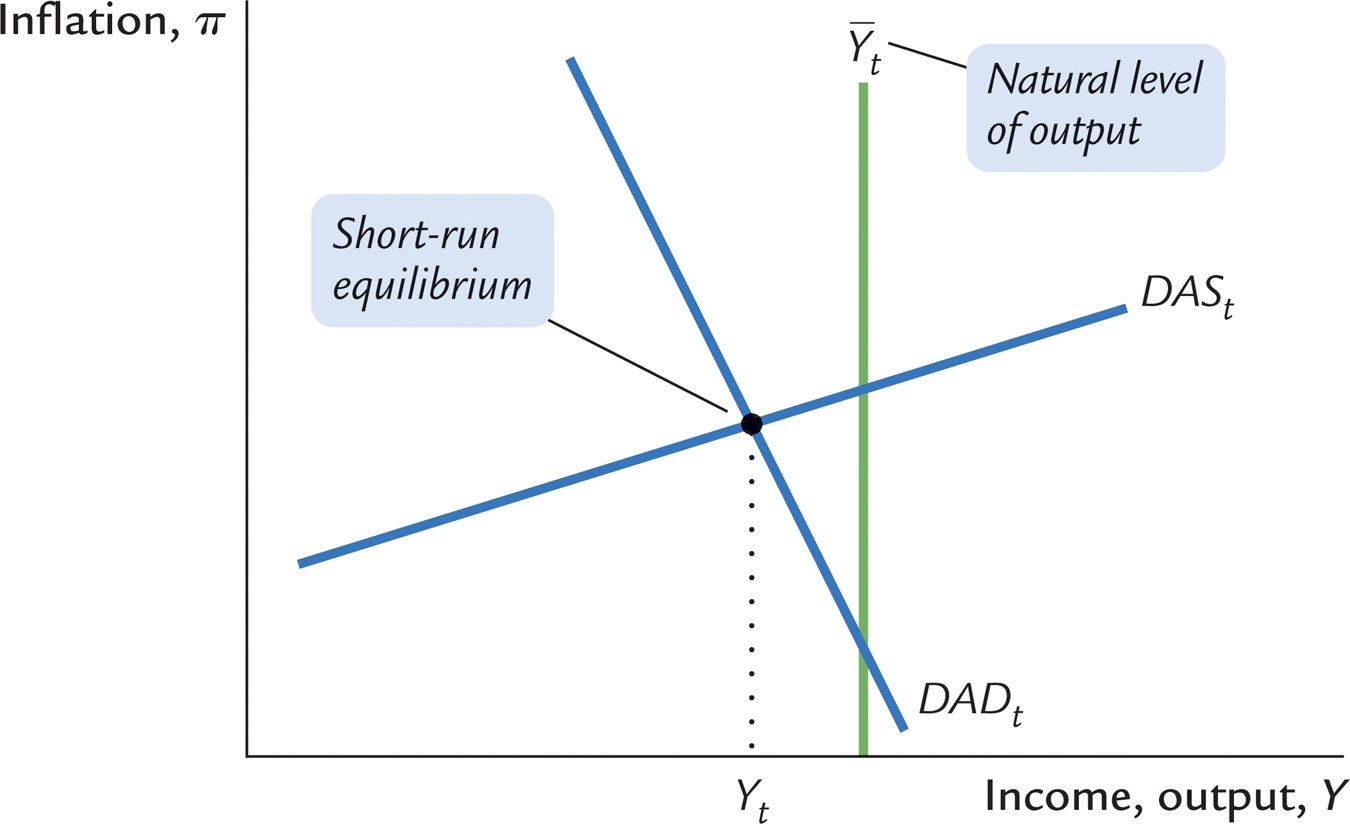
The Short-Run Equilibrium
The economy’s short-run equilibrium is determined by the intersection of the dynamic aggregate demand curve and the dynamic aggregate supply curve. The economy can be represented algebraically using the two equations we have just derived:
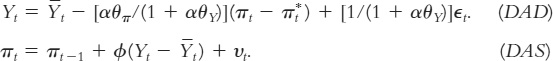
In any period t, these equations together determine two endogenous variables: inflation πt and output Yt. The solution depends on five other variables that are exogenous (or at least determined prior to period t). These exogenous (and predetermined) variables are the natural level of output 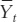 , the central bank’s target inflation rate
, the central bank’s target inflation rate 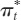 , the shock to demand εt, the shock to supply υt, and the previous period’s rate of inflation πt−1.
, the shock to demand εt, the shock to supply υt, and the previous period’s rate of inflation πt−1.
Taking these exogenous variables as given, we can illustrate the economy’s short-run equilibrium as the intersection of the dynamic aggregate demand curve and the dynamic aggregate supply curve, as in Figure 15-4. The short-run equilibrium level of output Yt can be less than its natural level 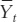 , as it is in this figure, greater than its natural level, or equal to it. As we have seen, when the economy is in long-run equilibrium, output is at its natural level
, as it is in this figure, greater than its natural level, or equal to it. As we have seen, when the economy is in long-run equilibrium, output is at its natural level 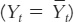 .
.
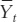 .
.
The short-run equilibrium determines not only the level of output Yt but also the inflation rate πt. In the subsequent period (t + 1), this inflation rate will become the lagged inflation rate that influences the position of the dynamic aggregate supply curve. This connection between periods generates the dynamic patterns that we examine in the next section. That is, one period of time is linked to the next through expectations about inflation. A shock in period t affects inflation in period t, which in turn affects the inflation that people expect for period t + 1. Expected inflation in period t + 1 in turn affects the position of the dynamic aggregate supply curve in that period, which in turn affects output and inflation in period t + 1, which then affects expected inflation in period t + 2, and so on.
These linkages of economic outcomes across time periods will become clear as we work through a series of examples.
454