Every day some workers lose or quit their jobs, and some unemployed workers are hired. This perpetual ebb and flow determines the fraction of the labor force that is unemployed. In this section we develop a model of labor-force dynamics that shows what determines the natural rate of unemployment.1

185

We start with some notation. Let L denote the labor force, E the number of employed workers, and U the number of unemployed workers. Because every worker is either employed or unemployed, the labor force is the sum of the employed and the unemployed:

L = E + U.

In this notation, the rate of unemployment is U/L.

To see what factors determine the unemployment rate, we assume that the labor force L is fixed and focus on the transition of individuals in the labor force between employment E and unemployment U. This is illustrated in Figure 7-2. Let s denote the rate of job separation, the fraction of employed individuals who lose or leave their jobs each month. Let f denote the rate of job finding, the fraction of unemployed individuals who find a job each month. Together, the rate of job separation s and the rate of job finding f determine the rate of unemployment.

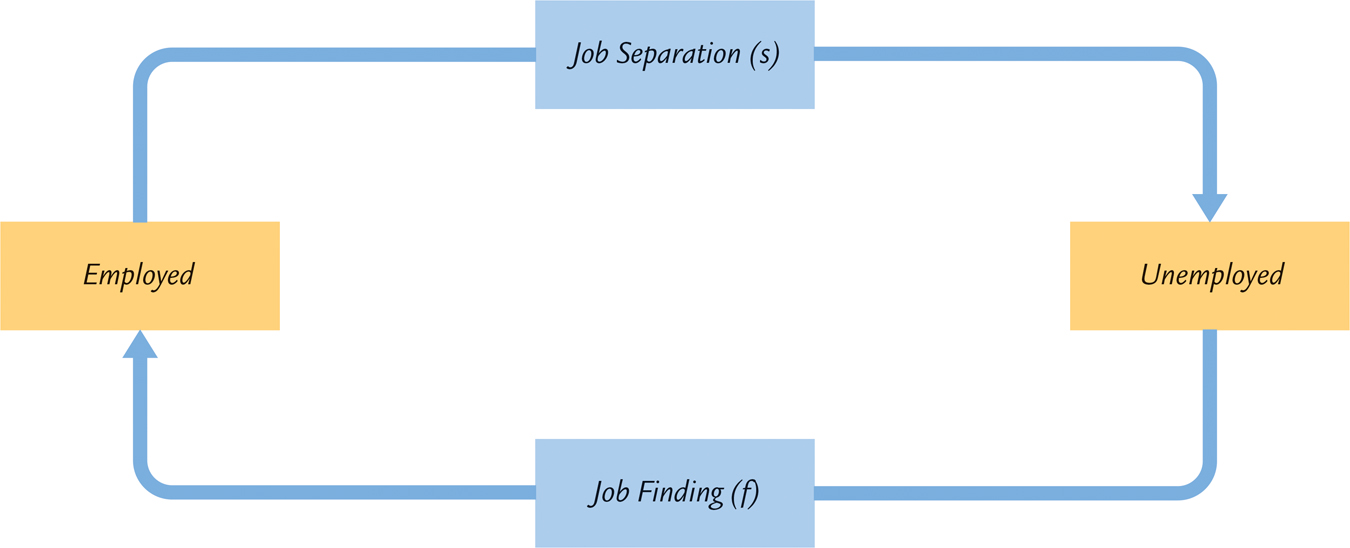
If the unemployment rate is neither rising nor falling—that is, if the labor market is in a steady state—then the number of people finding jobs fU must equal the number of people losing jobs sE. We can write the steady-state condition as

fU = sE.

186

We can use this equation to find the steady-state unemployment rate. From our definition of the labor force, we know that E = L − U; that is, the number of employed equals the labor force minus the number of unemployed. If we substitute (L − U) for E in the steady-state condition, we find

fU = s(L − U).

Next, we divide both sides of this equation by L to obtain

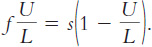
Now we can solve for the unemployment rate U/L to find

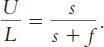
This can also be written as

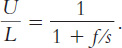
This equation shows that the steady-state rate of unemployment U/L depends on the rates of job separation s and job finding f. The higher the rate of job separation, the higher the unemployment rate. The higher the rate of job finding, the lower the unemployment rate.

Here’s a numerical example. Suppose that 1 percent of the employed lose their jobs each month (s = 0.01). This means that the average spell of employment lasts 1/0.01, or 100 months, about 8 years. Suppose further that 20 percent of the unemployed find a job each month (f = 0.20), so that the average spell of unemployment last 5 months. Then the steady-state rate of unemployment is

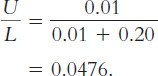
The rate of unemployment in this example is about 5 percent.

This simple model of the natural rate of unemployment has an important implication for public policy. Any policy aimed at lowering the natural rate of unemployment must either reduce the rate of job separation or increase the rate of job finding. Similarly, any policy that affects the rate of job separation or job finding also changes the natural rate of unemployment.

Although this model is useful in relating the unemployment rate to job separation and job finding, it fails to answer a central question: Why is there unemployment in the first place? If a person could always find a job quickly, then the rate of job finding would be very high and the rate of unemployment would be near zero. This model of the unemployment rate assumes that job finding is not instantaneous, but it fails to explain why. In the next two sections, we examine two underlying reasons for unemployment: job search and wage rigidity.

187