MORE PROBLEMS AND APPLICATIONS
Question 9.15
1. In the economy of Solovia, the owners of capital get two-thirds of national income, and the workers receive one-third.
The men of Solovia stay at home performing household chores, while the women work in factories. If some of the men started working outside the home so that the labor force increased by 5 percent, what would happen to the measured output of the economy? Does labor productivity—defined as output per worker—increase, decrease, or stay the same? Does total factor productivity increase, decrease, or stay the same?
In year 1, the capital stock was 6, the labor input was 3, and output was 12. In year 2, the capital stock was 7, the labor input was 4, and output was 14. What happened to total factor productivity between the two years?
Question 9.16
2. Labor productivity is defined as Y/L, the amount of output divided by the amount of labor input. Start with the growth-accounting equation and show that the growth in labor productivity depends on growth in total factor productivity and growth in the capital–labor ratio. In particular, show that
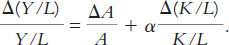
Hint: You may find the following mathematical trick helpful. If z = wx, then the growth rate of z is approximately the growth rate of w plus the growth rate of x. That is,
Δz/z ≈ Δw/w + Δx/x.
Question 9.17
3. Suppose an economy described by the Solow model is in a steady state with population growth n of 1.8 percent per year and technological progress g of 1.8 percent per year. Total output and total capital grow at 3.6 percent per year. Suppose further that the capital share of output is 1/3. If you used the growth-accounting equation to divide output growth into three sources—capital, labor, and total factor productivity—how much would you attribute to each source? Compare your results to the figures we found for the United States in Table 9-2.
1 Mathematical note: This model with technological progress is a strict generalization of the model analyzed in Chapter 8. In particular, if the efficiency of labor is constant at E = 1, then g = 0, and the definitions of k and y reduce to our previous definitions. In this case, the more general model considered here simplifies precisely to the Chapter 8 version of the Solow model.
2 Robert Barro and Xavier Sala-i-Martin, “Convergence Across States and Regions,” Brookings Papers on Economic Activity 1 (1991): 107–182; N. Gregory Mankiw, David Romer, and David N. Weil, “A Contribution to the Empirics of Economic Growth,” Quarterly Journal of Economics (May 1992): 407–437.
3 Robert E. Hall and Charles I. Jones, “Why Do Some Countries Produce So Much More Output per Worker Than Others?” Quarterly Journal of Economics 114 (February 1999): 83–116; Peter J. Klenow and Andres Rodriguez-Clare, “The Neoclassical Revival in Growth Economics: Has It Gone Too Far?” NBER Macroeconomics Annual (1997): 73–103.
4 Nicholas Bloom and John Van Reenen, “Measuring and Explaining Management Practices Across Firms and Countries,” The Quarterly Journal of Economics (2007) 122 (4): 1351–1408. In more recent work, Bloom, Van Reenan, and coauthors have extended their surveys to other nations. They report that, on average, American, Japanese, and German firms are the best managed, whereas firms in developing countries, such as Brazil, China, and India, tend to be poorly managed. See Nicholas Bloom, Christos Genakos, Raffaella Sadun, and John Van Reenen, “Management Practices Across Firms and Countries,” NBER Working Paper No. 17850, February 2012.
5 For more on this topic and some international evidence, see Andrew B. Abel, N. Gregory Mankiw, Lawrence H. Summers, and Richard J. Zeckhauser, “Assessing Dynamic Efficiency: Theory and Evidence,” Review of Economic Studies 56 (1989): 1–19.
6 Earlier in this chapter, when we were interpreting K as only physical capital, human capital was folded into the efficiency-of-labor parameter E. The alternative approach suggested here is to include human capital as part of K instead, so E represents technology but not human capital. If K is given this broader interpretation, then much of what we call labor income is really the return to human capital. As a result, the true capital share is much larger than the traditional Cobb–Douglas value of about 1/3. For more on this topic, see N. Gregory Mankiw, David Romer, and David N. Weil, “A Contribution to the Empirics of Economic Growth,” Quarterly Journal of Economics (May 1992): 407–437.
7 Paul Romer, “Crazy Explanations for the Productivity Slowdown,” NBER Macroeconomics Annual 2 (1987): 163–201.
8 Rafael La Porta, Florencio Lopez-de-Silanes, Andrei Shleifer, and Robert Vishny, “Law and Finance,” Journal of Political Economy 106 (1998): 1113–1155; Ross Levine and Robert G. King, “Finance and Growth: Schumpeter Might Be Right,” Quarterly Journal of Economics 108 (1993): 717–737.
9 Paulo Mauro, “Corruption and Growth,” Quarterly Journal of Economics 110 (1995): 681–712.
10 Daron Acemoglu, Simon Johnson, and James A. Robinson, “The Colonial Origins of Comparative Development: An Empirical Investigation,” American Economic Review 91 (December 2001): 1369–1401.
11 Jeffrey D. Sachs and Andrew Warner, “Economic Reform and the Process of Global Integration,” Brookings Papers on Economic Activity (1995): 1–95; Jeffrey A. Frankel and David Romer, “Does Trade Cause Growth?” American Economics Review 89 (June 1999): 379–399.
12 This section provides a brief introduction to the large and fascinating literature on endogenous growth theory. Early and important contributions to this literature include Paul M. Romer, “Increasing Returns and Long-Run Growth,” Journal of Political Econom 94 (October 1986): 1002–1037; and Robert E. Lucas, Jr., “On the Mechanics of Economic Development,” Journal of Monetary Economics 22 (1988): 3–42. The reader can learn more about this topic in the undergraduate textbook by David N. Weil, Economic Growth, 3rd ed. (Pearson, 2013).
13 For an overview of the empirical literature on the effects of research, see Zvi Griliches, “The Search for R&D Spillovers,” Scandinavian Journal of Economics 94 (1991): 29–47.
14 Robert J. Gordon, “Why Was Europe Left at the Station When America’s Productivity Locomotive Departed?” NBER Working Paper No. 10661, 2004.
15 Philippe Aghion and Peter Howitt, “A Model of Growth Through Creative Destruction,” Econometrica 60 (1992): 323–351.
16 Note the word approximately here. This answer is only an approximation because the marginal product of capital varies: it falls as the amount of capital increases. An exact answer would take into account the fact that each unit of capital has a different marginal product. If the change in K is not too large, however, the approximation of a constant marginal product is very accurate.
17 Mathematical note: To see that this is equivalent to the previous equation, note that we can multiply both sides of this equation by Y and thereby cancel Y from three places in which it appears. We can cancel the K in the top and bottom of the first term on the right-hand side and the L in the top and bottom of the second term on the right-hand side. These algebraic manipulations turn this equation into the previous one.
18 Robert M. Solow, “Technical Change and the Aggregate Production Function,” Review of Economics and Statistics 39 (1957): 312–320. It is natural to ask how growth in labor efficiency E relates to growth in total factor productivity. One can show that ΔA/A = (1 − α)ΔE/E, where α is capital’s share. Thus, technological change as measured by growth in the efficiency of labor is proportional to technological change as measured by the Solow residual.
19 For various views on the growth slowdown, see “Symposium: The Slowdown in Productivity Growth” in the Fall 1988 issue of the Journal of Economic Perspectives. For a discussion of the subsequent growth acceleration and the role of information technology, see “Symposium: Computers and Productivity” in the Fall 2000 issue of the Journal of Economic Perspectives.
20 Alwyn Young, “The Tyranny of Numbers: Confronting the Statistical Realities of the East Asian Growth Experience,” Quarterly Journal of Economics 101 (August 1995): 641–680.
21 To read more about this topic, see Edward C. Prescott, “Theory Ahead of Business Cycle Measurement,” and Lawrence H. Summers, “Some Skeptical Observations on Real Business Cycle Theory,” both in Quarterly Review, Federal Reserve Bank of Minneapolis (Fall 1986); N. Gregory Mankiw, “Real Business Cycles: A New Keynesian Perspective,” Journal of Economic Perspectives 3 (Summer 1989): 79–90; Bennett T. McCallum, “Real Business Cycle Models,” in Modern Business Cycle Theory, edited by R. Barro (Cambridge, MA: Harvard University Press, 1989), 16–50; and Charles I. Plosser, “Understanding Real Business Cycles,” Journal of Economic Perspectives 3 (Summer 1989): 51–77.
280