The principles of transmission genetics are statistical and are stated in terms of probabilities.
The element of chance in fertilization implies that the genotype of any particular progeny cannot be determined in advance. However, one can deduce the likelihood, or probability, that a specified genotype will occur. The probability of occurrence of a genotype must always lie between 0 and 1; a probability of 0 means that the genotype cannot occur, and a probability of 1 means that the occurrence of the genotype is certain. For example, in the cross Aa × AA, no offspring can have the genotype aa, so in this mating the probability of aa is 0. Similarly, in the mating AA × aa, all offspring must have the genotype Aa, so in this mating the probability of Aa is 1.
In many cases, the probability of a particular genotype is neither 0 nor 1, but some intermediate value. For one gene, the probabilities for a single individual can be deduced from the parental genotypes in the mating and the principle of segregation. For example, the probability of producing a homozygous recessive individual from the cross Aa × Aa is ¼ (see Fig. 16.7), and that from the cross Aa × aa is ½ (see Fig. 16.8a).
334
The genotype and phenotype probabilities for a single individual can also be inferred from observed data because the overall proportions of two (or more) genotypes among a large number of observations approximates the probability of each of the genotypes for a single observation. For example, in Mendel’s F2 data (see Table 16.1), the overall ratio of dominant:recessive is 2.98:1, or very nearly 3:1. This result implies that the probability that an individual F2 plant has the homozygous recessive phenotype is very close to ¼, which is the value inferred from the principle of segregation.
Sometimes it becomes necessary to combine the probabilities of two or more possible outcomes of a cross, as in determining the probability of a genotype occurring based on the probabilities of the gametes occurring. In such cases either of two rules are helpful:
Addition rule. This principle applies when the possible outcomes being considered cannot occur simultaneously. For example, suppose that a single offspring is chosen at random from the progeny of the mating Aa × Aa, and we wish to know the probability that the offspring is either AA or Aa. The key words here are “either” and “or.” Each of these outcomes is possible, but both cannot occur simultaneously in a single individual; the outcomes are mutually exclusive. When the possibilities are mutually exclusive, the addition rule states that the probability of either event occurring is given by the sum of their individual probabilities. In this example, the chosen offspring could either have genotype AA (with probability ¼, according to Fig. 16.7), or the offspring could have genotype Aa (with probability ½). Therefore, the probability that the chosen individual has either the AA or the Aa genotype is given by ¼ + ½ = ¾ (see Fig. 16.7). Alternatively, the ¾ could be interpreted to mean that, among a large number of offspring from the mating Aa × Aa, the proportion exhibiting the dominant phenotype will be very close to ¾. This interpretation is verified by the data in Table 16.1.
Multiplication rule. This principle applies when outcomes can occur simultaneously, and the occurrence of one has no effect upon the likelihood of the other. Events that do not influence one another are independent, and the multiplication rule states that the probability of two independent events occurring together is the product of their respective probabilities. This rule is widely used to determine the probabilities of successive offspring of a cross because each event of fertilization is independent of any other. For example, in the mating Aa × Aa, one may wish to determine the probability that, among four peas in a pod, the one nearest the stem is green and the others yellow. Here, the word “and” is a simple indicator that the multiplication rule should be used. Because each seed results from an independent fertilization, this probability is given by the product of the probability that the seed nearest the stem is aa and the probability that each of the other seeds is either AA or Aa, and hence the probability is ¼ × ¾ × ¾ × ¾ = 27/256, as shown for the top pod in Fig. 16.11.
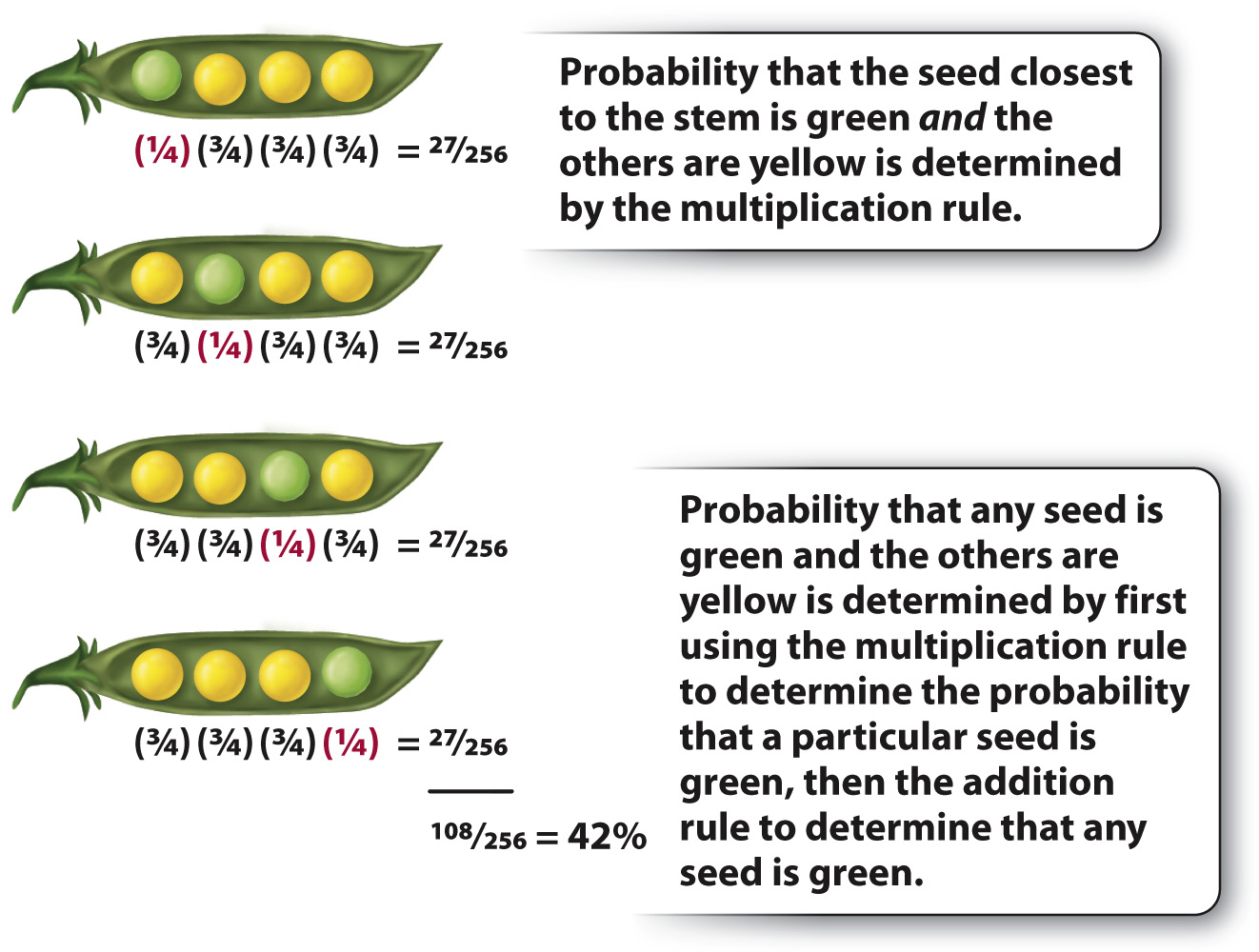
The addition and multiplication rules are very powerful when used in combination. Consider the following question: In the mating Aa × Aa, what is the probability that, among four seeds in a pod, exactly one is green? We have already seen in Fig. 16.11 that the multiplication rule gives the probability of the seed nearest the stem being green as 27/256. As illustrated in Fig. 16.11, there are only four possible ways in which exactly one seed can be green, each of which has a probability of 27/256, and these outcomes are mutually exclusive. Therefore, by the addition rule, the probability of there being exactly one green and three yellow seeds in a pod, occurring in any order, is given by 27/256 + 27/256 + 27/256 + 27/256 = 108/256, or approximately 42%.
Quick Check 4 What is the probability that any two peas are green and two are yellow in a pea pod with exactly four seeds?
Quick Check 4 Answer
The probability that the first pea is green is ¼; the probability that the second pea is green is also ¼; the probability that the third pea is yellow is ¾; and the probability that the fourth pea is yellow is ¾. We use the multiplication rule (because these are mutually exclusive events) to figure out that the probability of this configuration is ¼ × ¼ × ¾ × ¾ = 9/256. There are six different ways that a pod of four seeds can have two green seeds and two yellow seeds (the green seeds can be in positions 1 and 2; 1 and 3; 1 and 4; 2 and 3; 2 and 4; or 3 and 4). Each of these configurations occurs with a probability of 9/256 (as previously described). The probability of any of these occurring (either one configuration or another or another) is given by the addition rule, or 9/256 + 9/256 + 9/256 + 9/256 + 9/256 + 9/256 = (9/256) × 6 = 54/256, or about 21%.