11.3 Assess Your Understanding
Concepts and Vocabulary
Question
Multiple Choice If a smooth curve \(C\) is traced out by the vector function \(\mathbf{r}=\mathbf{r}(t)\), \(a\leq t\leq b,\) then the parameter \(t\) is the arc length if and only if [(a) \(\left\Vert \mathbf{r}(t)\right\Vert\;=1\), (b) \(\left\Vert \mathbf{r}^{\prime}(t)\right\Vert\;=0\), (c) \(\left\Vert \mathbf{r}^{\prime\prime}(t)\right\Vert\;=1\), (d) \(\left\Vert \mathbf{r}^{\prime}(t)\right\Vert\;=1]\) for all \(t.\)
Question
True or False The curvature \(\kappa\) of a straight line equals the slope of the line.
Question
True or False The curvature \(\kappa\) of a circle equals the radius of the circle.
Question
True or False The curvature \(\kappa\) of a twice differentiable smooth curve \(C\) equals the magnitude of the rate of change of the unit tangent vector \(\mathbf{T}\) with respect to arc length.
Question
True or False The curvature \(\kappa\) of a twice differentiable smooth curve \(C\): \(\mathbf{r}=\mathbf{r}(t)\) is \(\kappa\;=\;\dfrac{\left\Vert \mathbf{r}^{\prime} (t) \times \mathbf{r} ^{\prime \prime} (t) \right\Vert }{\left\Vert \mathbf{r}(t) \right\Vert ^{3}}.\)
Question
The curvature of a twice differentiable function \(y=f(x)\) is \(\kappa\) = _______.
Question
The curvature of the circle \(x^{2}+\left( y-2\right) ^{2}=9\) is \(\kappa\) = _______.
Question
True or False The radius \(\rho\) of the osculating circle at a point \(P\) of a smooth curve \(C\) equals \(\kappa,\) provided \(\kappa \neq 0.\)
Skill Building
In Problems 9–18, determine whether the parameter used for each curve is the arc length along the curve.
Question
\(\mathbf{r}(t)=4\cos t\mathbf{i}-4\sin t\mathbf{j},\quad 0\leq t\leq 2\pi\)
Question
\(\mathbf{r}(t)=\sin (3t)\mathbf{i}+\cos (3t)\mathbf{j,} \quad 0\leq t\leq 2\pi\)
Question
\(\mathbf{r}(t)=t^{2}\mathbf{i}+t\mathbf{j},\quad 0\leq t\leq 4\)
Question
\(\mathbf{r}(t)=t\mathbf{i}+t^{3}\mathbf{j,}\quad 0\leq t\leq 2\)
Question
\(\mathbf{r}(t)=(2t+1)\mathbf{i}+(3t-2)\mathbf{j},\quad 0\leq t\leq 5\)
Question
\(\mathbf{r}(t)=\mathbf{i}+t\mathbf{j},\quad 0\leq t\leq 1\)
Question
\(\mathbf{r}(t)=\left( \dfrac{2}{\sqrt{13}}t+1\right) \mathbf{i}+\left( \dfrac{3}{\sqrt{13}}t-2\right) \mathbf{j}\), \(0\leq t\leq 5\sqrt{13}\)
Question
\(\mathbf{r}(t)=\mathbf{i}+t^{2}\mathbf{j},\quad 0\leq t\leq 1\)
Question
\(\mathbf{r}(t)=\sin t\mathbf{i}+\cos t\mathbf{j}+t\mathbf{k}\)
Question
\(\mathbf{r}(t)=a\sin t\mathbf{i}+a\cos t\mathbf{j}+\sqrt{1-a^{2}}\kern1pt t\mathbf{k}\)
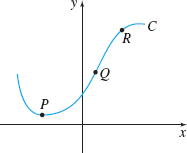
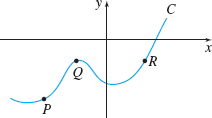
In Problems 19 and 20, rank the curvature of each curve at points \(P,\) \(Q\), and \(R\) from greatest to least.
Question
Question
In Problems 21–26, find the curvature \(\kappa\) of each plane curve traced out by the vector function \(\mathbf{r}=\mathbf{r}(t)\).
Question
\(\mathbf{r}(t)=t^{2}\mathbf{i}+\dfrac{2}{t}\mathbf{j}\)
Question
\(\mathbf{r}(t)=2t\mathbf{i}+t^{3}\mathbf{j}\)
Question
\(\mathbf{r}(t)=2\sin t \mathbf{i}+2\cos t\mathbf{j}\)
Question
\(\mathbf{r}(t)=\cos t\mathbf{i}+2\sin t\mathbf{j}\)
Question
\(\mathbf{r}(t)=(3t-t^{3})\mathbf{i}+3t^{2} \mathbf{j}\)
Question
\(\mathbf{r}(t)=(3t-t^{3})\mathbf{i}+(3t+t^{3})\mathbf{j}\)
783
In Problems 27–34, find the curvature \(\kappa\) of each space curve traced out by the vector function \(\mathbf{r}=\mathbf{r}(t)\).
Question
\(\mathbf{r}(t)=t\mathbf{i}+2t\mathbf{j}+t\mathbf{k}\)
Question
\(\mathbf{r}(t)=2t\mathbf{i}+t\mathbf{j}+3t\mathbf{k}\)
Question
\(\mathbf{r}(t)=\sin ( 2t) \mathbf{i}+\cos ( 2t) \mathbf{j}+t\mathbf{k}\)
Question
\(\mathbf{r}(t)=\sin t\mathbf{i}\,{+}\,\cos t\mathbf{j}\,{+}\,bt\mathbf{k}\)
Question
\(\mathbf{r}(t)=e^{t}\mathbf{i}+e^{-t}\mathbf{j}+\sqrt{2}t\mathbf{k}\)
Question
\(\mathbf{r}(t)=e^{t}\mathbf{i}+e^{2t}\mathbf{j}+e^{-t}\mathbf{k}\)
Question
\(\mathbf{r}(t)=\cos ^{3}t\mathbf{i}+\sin ^{3}t\mathbf{j}+\mathbf{k}\)
Question
\(\mathbf{r}(t)=4\cos ^{3}t\mathbf{i}+ 3\mathbf{j} + 4\sin^{3}t\mathbf{k}\)
In Problems 35–44, find the curvature \(\kappa\) of the graph of each function at the indicated point.
Question
\(y=x^{2}\) at \((1,1)\)
Question
\(y=2x-x^{2}\) at \((1,1)\)
Question
\(y=x^{2}-x^{3}\) at \((1,0)\)
Question
\(y=x^{-3/2}\) at \((1,1)\)
Question
\(y=\sqrt{x}\) at \(\big(2,\sqrt{2}\big)\)
Question
\(y=\dfrac{1}{\sqrt{x}}\) at \((1,1)\)
Question
\(4x^{2}+9y^{2}=36\) at \((0,2)\)
Question
\(y=\sec x-1\) at \(\left(\! \dfrac{\pi}{4}, \sqrt{2}-1\!\right)\)
Question
\(y=e^{x}\) at \((0,1)\)
Question
\(y=\ln (x+1)\) at \(( 2,\ln 3)\)
In Problems 45–52, find the radius of the osculating circle at the indicated point.
Question
\(y=x^{3}-6x\) at \((1,-5)\)
Question
\(y=\dfrac{1}{x^{2}}\) at \((-1,1)\)
Question
\(y=\sin x\) at \(\left( \dfrac{\pi }{2},1\right)\)
Question
\(y=e^{-x}\) at \((0,1)\)
Question
\(x^{2}+xy+y^{2}=3\) at \((1,1)\)
Question
\(y^{2}-y+x=0\) at \((0,0)\)
Question
\(y=\ln ( \sec x)\) at \(\left(\dfrac{\pi }{4}, \ln \sqrt{2}\right)\)
Question
\(y=\cosh x\) at \((0,1)\)
In Problems 53–62, find the radius of the osculating circle at the point corresponding to \(t\) on the curve \(C\) traced out by the vector function \(\mathbf{r}=\mathbf{r}(t)\).
Question
\(\mathbf{r}(t)=3t^{2}\mathbf{i}+(3t-t^{3})\mathbf{j,}\quad t=1\)
Question
\(\mathbf{r}(t)=t\mathbf{i}+t^{2}\mathbf{j}+t^{3}\mathbf{k},\quad t=1\)
Question
\(\mathbf{r}(t)=\sin t\mathbf{i}+\cos ( 2t) \mathbf{j},\quad t=\dfrac{\pi }{4}\)
Question
\(\mathbf{r}(t)=\sin t\mathbf{i}+\cos t\mathbf{j}+bt\mathbf{k,}\quad b>0,\quad t=\dfrac{\pi }{4}\)
Question
\(\mathbf{r}(t)=\sin ( 2t) \mathbf{i}+\cos ( 2t) \mathbf{j}+t\mathbf{k,}\quad t=\dfrac{\pi }{4}\)
Question
\(\mathbf{r}(t)=\cos ^{3}t\mathbf{i}+\sin ^{3}t\mathbf{j}+\mathbf{k,}\quad t=\dfrac{\pi }{3}\)
Question
\(\mathbf{r}(t)=e^{t}\mathbf{i}+e^{-t}\mathbf{j}+\sqrt{2}\kern1ptt\mathbf{k},\quad t=0\)
Question
\(\mathbf{r}(t)=a(3t-t^{3})\mathbf{i}+3at^{2}\mathbf{j}+a(3t+t^{3})\mathbf{k,}\quad a>0, \quad t=1\)
Question
\(\mathbf{r}(t)=4a\cos ^{3}t\mathbf{i}+4a\sin ^{3}t\mathbf{j}+3a\cos ( 2t) \mathbf{k,}\quad a>0,\quad t=\dfrac{\pi }{4}\)
Question
\(\mathbf{r}(t)=t\mathbf{i}+2t\mathbf{j}+\sqrt{1-5t^{2}}\kern1pt\mathbf{k},\quad -\dfrac{\sqrt{5}}{5} < t< \dfrac{\sqrt{5}}{5},\quad t=0\)
Applications and Extensions
Question
Radius of Curvature Show that the radius of curvature of the parabola \(y=ax^{2}+bx+c\) is a minimum at its vertex.
Question
Radius of Curvature Show that the radii of curvature at the ends of the axes of the ellipse \(b^{2}x^{2}+a^{2}y^{2}=a^{2}b^{2}\) are \(\dfrac{b^{2}}{a}\) and \(\dfrac{a^{2}}{b},\quad a>0, b>0.\)
Question
Maximum Curvature Find the point on the curve \(y=\ln x\) at which the curvature is maximum.
Question
Maximum Curvature Find the point on the curve \(y=e^{x}\) at which the curvature is maximum.
Question
Maximum Curvature Find the point(s) on the curve \(y=\dfrac{1}{3}x^{3}\) at which the curvature is maximum.
Question
Maximum Curvature Find the point(s) on the curve \(y=\sin x\) at which the curvature is maximum.
Question
Maximum Curvature Find \(\alpha >0\) so that \(\mathbf{r} (t)= \alpha \cos t\mathbf{i} + \alpha \sin t\mathbf{j}+t\mathbf{k}\) has maximum curvature.
Question
Curvature of a Plane Curve What is the curvature at a point of inflection of a plane curve?
Question
Curvature of a Catenary Show that the curvature of the catenary \(y=a\cosh \dfrac{x}{a}\), \(a>0\), at any point \((x,y)\) is \(\dfrac{a}{y^{2}}\).
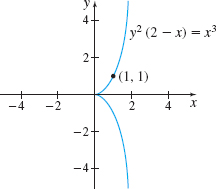
Question
Curvature of a Cissoid Find the curvature of the cissoid \(y^{2}(2-x)=x^{3}\) at the point \((1,1)\). See the figure.
Question
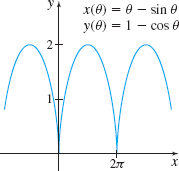
Curvature of a Cycloid Find the curvature of the cycloid \(x( \theta)\;=\;\theta -\sin \theta\) and \(y( \theta )\;=\;1-\cos \theta\) at the highest point of an arch. See the figure.
Question
- Find the curvature of the curve \(\mathbf{r} (t)=(1-t^{3})\mathbf{i}+t^{2}\mathbf{j}\).
 Graph \(\mathbf{r}=\mathbf{r}(t)\). Where is the curvature undefined? Do you see any geometric reason for this?
Graph \(\mathbf{r}=\mathbf{r}(t)\). Where is the curvature undefined? Do you see any geometric reason for this?
Question
Curvature of a Spiral Find the curvature of the spiral \(\mathbf{r}(t)=e^{-t}\cos t\mathbf{i}+e^{-t}\sin t\mathbf{j}\) shown in the figure. How does the curvature behave when \(t\rightarrow \infty\)? Do you see any geometric reason for this?
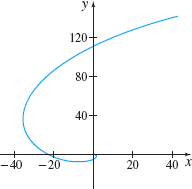
784
Question
Curvature Find the curvature \(\kappa\) of the curve \(\mathbf{r}(t)=2a\cos t\mathbf{i}+2a\sin t\mathbf{j}+bt^{2}\mathbf{k},\qquad a>0, b>0.\)
Question
Curvature Show that the curvature of an ellipse \(\dfrac{x^{2}}{ a^{2}}+\dfrac{y^{2}}{b^{2}}=1,\) \(a> b,\) is a maximum at the points \( \left( \pm a,0\right)\) and is a minimum at the points \(\left( 0,\pm b\right).\)
Source: Contributed by the students at the University of Missouri.
Question
Curvature Show that the circular helix \(\mathbf{r}( t)\;=\;a\cos t\mathbf{i}+a\sin t\mathbf{j}+t\mathbf{k}\) has constant curvature.
Source: Contributed by the students at the University of Missouri.
Question
Write a vector equation for the curve \(C\) traced out by \( \mathbf{r}(t)=2t\mathbf{i}+(2t-1)\mathbf{j}+t\mathbf{k}\), \(0\leq t\leq 2\), using arc length \(s\) as the parameter. [Hint: For each \(t\), \(0\leq t\leq 2\), calculate the length \(s(t)\) of the curve from \(0\) to \(t\).]
Question
Compare the solutions of Problems 13 and 15. Then show how to change the parameter \(t\) of the line \(\mathbf{r}(t)=(at+b)\mathbf{i}+(ct+d) \mathbf{j}\), where either \(a\neq 0\) or \(c\neq 0\), to one that is arc length as measured along the line.
Question
Suppose a smooth curve \(C\) is traced out by a twice differentiable vector function \(\mathbf{r}=\mathbf{r}(t)\), \(a\leq t\leq b\). If the curvature \(\kappa \neq 0\) at a point \(P\) on \(C\), show that the position vector \(\mathbf{C}\) of the center of the osculating circle at \(P\) is given by \begin{equation*} \mathbf{C}(t)=\mathbf{r}(t)+\rho \mathbf{N}(t) \end{equation*} where \(\mathbf{N}\) is the principal unit normal vector to \(C\) at \(P\), and \(\rho\;=\;\dfrac{1}{\kappa }\).
Question
Use the result of Problem 81 to find the center and radius of the osculating circle for the helix \(\mathbf{r}(t)=a\sin t\mathbf{i}+a\cos t\mathbf{j}+a^{2}t\mathbf{k}\), \(a>0;\) \begin{equation*} {\bf (a)}\enspace at\enspace t=\dfrac{\pi }{2}.\qquad {\bf (b)}\enspace at\enspace t=\pi. \end{equation*}
Use the following discussion for Problems 83–87. Suppose \(C\) is a smooth curve traced out by the twice differentiable vector function \(\mathbf{r}=\mathbf{r}(s),\) \(\ a\leq s\leq b,\) where \(s\) is arc length as measured along \(C\) . Define the binormal vector \(\mathbf{B}\) of \(C\) as \(\mathbf{B}(s)=\mathbf{T}(s)\times \mathbf{N}(s)\).
Question
Show that the three vectors \(\mathbf{T}, \mathbf{N}\), and \(\mathbf{B}\) form a collection of mutually orthogonal unit vectors at each point on \(C\).
Question
Show that \(\dfrac{d\mathbf{T}}{ds}=\kappa (s)\mathbf{N}(s)\).
Question
Show that \(\dfrac{d\mathbf{B}}{ds}\) is orthogonal to both \(\mathbf{B}(s)\) and \(\mathbf{T}(s).\)
Question
If the torsion \(\tau (s)\) of \(C\) is defined by the equation \(\dfrac{d\mathbf{B}}{ds}=-\tau \mathbf{N}\), show that \(\dfrac{d\mathbf{N}}{ds}=\tau \mathbf{B}-\kappa \mathbf{T}\).
Question
Find \(\kappa, \mathbf{T}, \mathbf{N}\), and \(\mathbf{B}\) for \(\mathbf{r}(s)=\dfrac{1}{\sqrt{2}}\left[ \sin s\mathbf{i}+\cos s\mathbf{j}+s\mathbf{k}\right].\)
Challenge Problems
Question
Curvature of a Polar Curve Show that the formula for the curvature of a polar curve \(r=f(\theta )\) is \begin{eqnarray*} \\[-24pt] \kappa\;=\;\frac{\left\vert r^{2}+2\!\left( \dfrac{dr}{d\theta }\right) ^{2}-r\left( \dfrac{d^{2}r}{d\theta ^{2}}\right) \right\vert }{\left[ r^{2}+\left( \dfrac{dr}{d\theta }\right) ^{2}\right] ^{3/2}} \end{eqnarray*}
In Problems 89–94, use the result of Problem 88 to find the curvature of each polar curve.
Question
\(r=2\cos ( 2\theta )\) at \(\theta\;=\;\dfrac{\pi }{12}\)
Question
\(r=e^{a\theta }\) at \(\theta\;=\;\dfrac{\pi }{2}, a>0\)
Question
\(r=a\theta\) at \(\theta\;=\;1\) and \(a>0\)
Question
\(r=1-\cos \theta\) at \(\theta\;=\;0\)
Question
\(r=3-2\sin \theta\) at \(\theta\;=\;\dfrac{\pi }{6}\)
Question
\(r=2+3\cos \theta\) at \(\theta\;=\;\dfrac{\pi }{3}\)
Question
Use the figure below to show that the coordinates \((h,k)\) of the center of curvature of \(y=f(x)\) are \begin{equation*} h=x-\rho \sin \phi\qquad k=y+\rho \cos \phi \end{equation*} where \(\rho \) is the radius of curvature. Show that \begin{equation*} \sin \phi\;=\;\frac{y^{\prime} }{\sqrt{1+( y^{\prime} ) ^{2}}}\qquad \hbox{and}\qquad \cos \phi\;=\;\frac{1}{\sqrt{1+( y^{\prime} ) ^{2}}} \end{equation*} so \begin{equation*} h=x-\frac{y^{\prime} \left[ 1+( y^{\prime} ) ^{2}\right] }{y^{\prime \prime}}\qquad \hbox{and}\qquad k=y+\frac{1+( y^{\prime} ) ^{2}}{y^{\prime \prime} } \end{equation*}
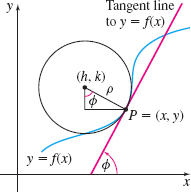
In Problems 96–100, use the result obtained in Problem 95 to find the center of curvature of each function.
Question
\(y=x^{2}\) at \(x=1\)
Question
\(y=\sin x\) at \(x=\dfrac{\pi }{2}\)
Question
\(y=\dfrac{x}{x+1}\) at \((0, 0)\)
Question
\(x^{3}+y^{3}=4xy\) at \((2, 2)\)
Question
\(xy=4\) at \(x=2\)
Question
As a point \(P\) moves along a curve \(C\), the center of curvature corresponding to \(P\) traces out a curve \(C_{1}\) called the evolute of \(C\); conversely, \(C\) is the involute of \(C_{1}\). Show that parametric equations of the evolute of \(y=\dfrac{1}{2}x^{2}\) are \(h=-x^{3}\), \(k=\dfrac{3}{2}x^{2}+1\). Then eliminate the parameter \(x\) to obtain \begin{equation*} h^{2}=\frac{8}{27}\left( k-1\right) ^{3} \end{equation*}
Question
Refer to Problem 101. Find parametric equations and a rectangular equation for the evolute of \(\mathbf{r}( t)\;=\;a\cos t\mathbf{i}+b\sin t\mathbf{j}\), \(a>0, b>0, 0\leq t\leq 2\pi \).
