Question

Multiple Choice Suppose \(z=f(x,y)\) is a function with domain \(D,\) and \((x_{0},y_{0})\) is an interior point of \(D\). If \( f(x_{0},y_{0})\geq f(x,y)\) for all points \((x,y)\) within some disk centered at \((x_{0},y_{0})\), then \(f\) has [(a) a local maximum, (b) a local minimum, (c) a saddle point, (d) none of these] at \((x_{0},y_{0})\).

Question

If \(z=f(x,y)\) is a function of two variables whose domain is an open set \(D\) containing the point \((x_{0},y_{0})\), then the point \( (x_{0},y_{0})\) is a critical point of \(f\) if ________ or if \(f_{x}(x_{0},y_{0})\) or \( f_{y}(x_{0},y_{0})\) does not exist.

Question

True or False It is possible for a function \(z=\) \( f( x,y) \) to have a local extremum at a point \(( x_{0},y_{0}) \) that is not a critical point.

Question

Multiple Choice If \(f_{x}( x_{0},y_{0}) =0\) and \(f_{y}( x_{0},y_{0}) =0,\) but \(f\) does not have a local extremum at \((x_{0},y_{0}),\) then the point \(( x_{0},y_{0},f( x_{0},y_{0}) ) \) is called [(a) a local maximum value, (b) a local minimum value, (c) a saddle point, (d) none of these].

Question

True or False The Extreme Value Theorem for Functions of Two Variables guarantees that a function \(z=f(x,y)\) of two variables, that is continuous on a closed, bounded set \(D\), has an absolute maximum and an absolute minimum on \(D\).

Question

If \(z=f(x,y)\) is a function of two variables that is continuous on a closed, bounded set \(D,\) then the absolute extrema are either at the critical points of \(f\) in \(D\) or on the ________ of \(D\).

Question

\(f(x,y)=x^{4}-2x^{2}+y^{2}+5\)

Question

\(f(x,y)=x^{2}-y^{2}+6x-2y+4\)

Question

\(f(x,y)=4xy-x^{4}-y^{4}+2\)

Question

\(f(x,y)=x^{3}+6xy+3y^{2}+3\)

Question

\(f(x,y)=e^{-x}( x^{2}+y)\)

Question

\(f(x,y)=xy+\dfrac{2}{x}+\dfrac{4}{y}\)

Question

\(z=x^{2}+y^{2}-2x+4y+2\)

Question

\(z=x^{2}+y^{2}-4x+2y-4\)

Question

\(z=x^{2}+4y^{2}-4x+8y-1\)

Question

\(z=2x^{2}-y^{2}+4x-4y+8\)

Question

\(z=x^{3}-6xy+y^{3}\)

Question

\(z=x^{2}-3xy-y^{2}\)

Question

\(z=x^{2}+3xy-y^{2}+4y-6x\)

Question

\(z=2x^{2}+xy+y^{2}-2x+3y+6\)

Question

\(z=x^{3}+3xy+y^{3}\)

Question

\(z=x^{3}-3xy-y^{3}\)

Question

\(z=x^{3}+x^{2}y+y^{2}\)

Question

\(z=3y^{3}-x^{2}y+x\)

889

Question

\(z=\dfrac{y}{x+y}\)

Question

\(z=\dfrac{x}{x+y}\)

Question

\(z=\cos x+\cos y\)

Question

\(z=x^{2}+4-4x\,\cos y\)

Question

\(z=y^{2}-6y\,\cos x+6\)

Question

\(z=x^{2}-6x\,\sin y+2\)

Question

\(z=e^{xy}\)

Question

\(z=xye^{-(x+y)}\)

Question

\(z=f(x,y)=x^{2}-4xy+4y;\quad D{:}\) \(\ 0\leq x\leq 2,\) \(0\leq y\leq 1\)

Question

\(z=f(x,y)=x^{2}-4xy+4y;\quad D{:}\) \(\ 0\leq x\leq 3,\) \(0\leq y\leq 2\)

Question

\(z=f(x,y)=x^{2}+2x+y^{2}-2y;\quad D{:}\) \(x^{2}+y^{2}\leq 4\)

Question

\(z=f(x,y)=x^{2}-4x+y^{2}-4y;\quad D{:}\) \(\ x^{2}+y^{2}\leq 9\)

Question

\(z=f(x,y)=xy + 4x-3y+5;\quad D\): the closed triangular region whose vertices are \((0,0),(0,4)\), and \((5,4)\)

Question

\(z=f(x,y)=3xy-3x-6y+1;\quad D\): the closed triangular region whose vertices are \((0,0),(5,0)\), and \((0,3)\)

Question

Find the local extrema and saddle points, if any, of \(f(x,y)=\sin x\,\sin y\).

Question

Find the highest point of the paraboloid \(x^{2}+y^{2}-6x+4y+ z+12=0\) as follows:

1. Find the vertex of the paraboloid without using calculus.
2. Express \(z\) as a function of \(x\) and \(y\) and use calculus to find its maximum value. See the figure.

Question

Geography The surface of a mountain is modeled by the graph of the function \(z=2xy-2x^{2}-y^{2}-8x+6y+4\) where \(z\) is the height in kilometers. See the figure. If sea level is the \(xy\)-plane, how high is the mountain above sea level?

Question

Geometry 

1. Find the point on the plane \(3x+2y+z=14\) that is nearest the origin.
2. What is the distance of the point found in (a) from the origin?

Question

Constructing a Box A rectangular box open at the top has a fixed surface area \(S\). Find the dimensions that make its volume a maximum. Express the length, width, and depth of the box in terms of \(S\).

Question

Constructing a Box Rework Problem 43 if the box is closed.

Question

Constructing a Box Rework Example 7 if the box is closed.

Question

Minimizing Cost Find the dimensions of an open rectangular box having a volume of 80 cubic meters if the cost per square meter of the material to be used is \($\)4 for the bottom, \($\)3 for one of the sides, and \($\)2 for the three remaining sides, and the total cost is to be minimized.

Question

Standard Post Regulations The U.S. Postal Service regulations state that individual packages can be up to 130 in. in combined length and girth (the perimeter of a cross section at the widest spot) before additional charges apply.

1. Find the dimensions of maximum volume of a rectangular box that can be sent without additional charges.
2. Find the dimensions of maximum volume if the package is cylindrical.

Question

Pharmacology A patient's reaction to an injection of \(x\) units of a certain drug, \(t\) hours after the injection, is given by \[ y=x^{2}(a-x)te^{-t}\qquad 0\leq x\leq a\quad 0\leq t \]

where \(a>0\) is a constant. Find the values of \(x\) and \(t\), if any, that will maximize \(y\).

Question

Pharmacology Two drugs A and B are used simultaneously to treat a disease. A patient's reaction \(R\) to \(x\) units of Drug A and \(y\) units of Drug B is \[ R( x,y) =x^{2}y^{2}( a-x) ( b-y) \qquad 0\leq x\leq a\quad 0\leq y\leq b \]

1. For a fixed amount \(x\) of Drug A, what amount \(y\) of Drug B produces the maximum reaction?
2. For a fixed amount \(y\) of Drug B, what amount \(x\) of Drug A produces the maximum reaction?
3. If amounts \(x\) and \(y\) are both variable, what amount of each maximizes the reaction?

Question

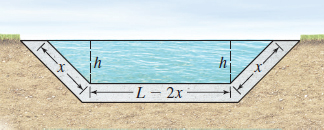
Agriculture An open irrigation channel is to be made into a symmetric form with three straight sides, as indicated in the figure.

1. If the total length of the three sides is \(L\), find a channel design that will allow the maximum possible flow.
2. Is this design preferable to an open semicircular channel? Give the reasons why.

Question

Fuel Cost See the figure. A fuel reservoir at \(D\) is planned to service plants located at \(A,B\), and \(C\). The cost, in thousands of dollars, of connecting the plants to \(D\) is determined by the formula \[ F=6x^{2}+6y^{2}-4x-6y+5 \]

Find the location of \(D\) that will minimize this cost.

890

Question

Minimizing Cost The cost of material for the sides of a rectangular shipping container is \(a\) cents per square foot; the cost of the top and bottom material is \(\dfrac{3}{2}a\) cents per square foot. If the volume is to be \(\dfrac{3}{2}\) cubic feet, what dimensions of the container will minimize its cost?

Question

Metal Detector A metal detector is used to locate an underground pipe. When several meter readings of the detector are compared, the reading at an arbitrary point \((x,y),\) where \(x\geq 0\) and \(y\geq 0,\) is modeled by \[ M=y(x-x^{2})-y^{2} \]

Find the point \((x,y)\) with the highest reading.

Question

Maximizing Profit A steel manufacturer produces two grades of steel, \(x\) kilograms of Grade A and \(y\) kilograms of Grade B. The cost \(C\) and revenue \(R\) in dollars are given by \[ \begin{eqnarray*} C& =&\frac{1}{20}x^{2}+700x+y^{2}-150y-\frac{1}{2}xy \\[4pt] R& =&2700x-\frac{3}{20}x^{2}+1000y-y^{2}+\frac{1}{2}xy+10{,}000 \end{eqnarray*} \]

If \(P=\) Profit \(=R-C\), find the production (in kilograms) of Grades A and B that maximizes the manufacturer's profit.

Question

Economics The demand functions for two products are \(p=12-x\) and \(q=8-y,\) where \(p\) and \(q\) are the respective prices (in thousands of dollars) of each product, and \(x\) and \(y\) are the respective amounts (in thousands of units) of each product sold. If the joint cost function is \[ C( x,y) =x^{2}+2xy+3y^{2} \]

find the quantities \(x\) and \(y\) and the prices \(p\) and \(q\) that maximize profit. What is the maximum profit?

Question

Economics The labor cost of a firm is modeled by the function \[ Q( x,y) =x^{2}+y^{2}-xy-3x-6y-5 \]

where \(x\) is the number of workdays required by a skilled worker and \(y\) is the number of workdays required by a semiskilled worker. Find the values of \(x\) and \(y\) for which the labor cost is a minimum.

Question

Second Partial Derivative Test Suppose \[ g(x,y)=Ax^{2}+2Bxy+ Cy^{2} \]

where \(A,\) \(B\), and \(C\) are constants. Show that:

1. If \(AC-B^{2}>0,\) \(A\neq 0\), then \(g(x,y)\) has a maximum at \((0,0)\) if \(A<0\) and a minimum at \((0,0)\) if \(A>0\).
2. If \(AC-B^{2}<0\), then \(g(x,y)\) has both positive and negative values at points \((x,y)\) near \((0,0),\) and \((0,0,0)\) is a saddle point for \(z=g(x,y)\).

Question

Find the distance between the skew lines \(L_{1}\): \(x=t-6\), \( y=t\), \(z=2t\) and \(L_{2}\): \(x=t\), \(y=t\), \(z=-t\).

Question

Method of Least Squares A scientist plots the points \( (x_{1},y_{1}),\ldots ,(x_{n},y_{n})\) from her experimental data. Her theory tells her that the points should lie on a straight line, but they do not; perhaps as a result of experimental error. She is looking for the line \(y=mx+b\) that “best” fits the data. The most often used criterion is the least-squares fit, in which \(m\) and \(b\) are chosen to minimize \[ \sum_{k=1}^{n}(mx_{k}+b-y_{k})^{2} \]

1. Show that the minimizing values of \(m\) and \(b\) are \[ \begin{eqnarray*} m& =&\dfrac{n\sum\limits_{k=1}^{n}x_{k}y_{k}-\sum\limits_{k=1}^{n}x_{k}\sum \limits_{k=1}^{n}y_{k}}{n\sum\limits_{k=1}^{n}x_{k}^{2}-\left( \sum\limits_{k=1}^{n}x_{k}\right) ^{2}} \\[4pt] b& =&\dfrac{1}{n}\left( \sum\limits_{k=1}^{n}y_{k}-m\sum_{k=1}^{n}x_{k}\right) \end{eqnarray*} \]
2. Find the least-squares estimate \(y=mx+b\) for the points \( (0,2),(1,1),(2,2),(3,4)\), and \((4,4)\). Plot the points and draw the line.

Question

Let \(z=f(x,y)\) have continuous second-order partial derivatives. If \(\mathbf{u}=u_{1}\mathbf{i}+u_{2}\mathbf{j}\) is a unit vector, there is a directional derivative \(D_{\mathbf{u}}f(x,y)=g(x,y)\). If \( g(x,y)\) is differentiable and if \(\mathbf{v}=v_{1}\mathbf{i}+v_{2}\mathbf{j}\) is a unit vector, then \(D_{\mathbf{v} }g(x,y)=u_{1}v_{1}f_{xx}+ (u_{1}v_{2}+u_{2}v_{1})f_{xy}+u_{2}v_{2}f_{yy}\). If we let \(A=f_{xx}(x_{0},y_{0})\), \( B=f_{xy}(x_{0},y_{0})\), and \(C=f_{yy}(x_{0},y_{0})\), then we have an expression in the components \(u_{1}\) and \(u_{2}\) of \(\mathbf{u}\), and \(v_{1}\) and \(v_{2}\) of \(\mathbf{v}\). (See Section 13.1, Problem 77.)

1. In particular, if \(\mathbf{u}=\mathbf{v}\), show that the second-order directional derivative at \((x_{0},y_{0})\) is given by \( Au_{1}^{2}+2Bu_{1}u_{2}+Cu_{2}^{2}\).
2. Let \(f_{x}(x_{0},y_{0})=0=f_{y}(x_{0},y_{0})\). Show that if \( A>0 \) and \(AC-B^{2}>0\), then \(z=f(x,y)\) has a local minimum at \( (x_{0},y_{0}) \); if \(A<0\) and \(AC-B^{2}>0\), then \(z=f(x,y)\) has a local maximum at \((x_{0},y_{0})\); and if \(AC-B^{2}<0\), then \(z=f(x,y)\) has a saddle point at \((x_{0},y_{0})\).
3. Show by examples that if \(AC-B^{2}=0\), we may have any of the above three possibilities.