Question

To convert a point \(P=\left( x,y,z\right) \) from rectangular coordinates to cylindrical coordinates \(\left( r,\theta ,z\right) \), use the equations: x =_____, y =_____, and z =_____.

Question

Expressed in cylindrical coordinates, the circular cone \( x^{2}+y^{2}=4z^{2}\) has the form _____.

Question

In cylindrical coordinates \(( r,\theta ,z) \), the differential \(dV\) of volume is _____

Question

True or False In cylindrical coordinates, \( \iiint\limits_{\kern-3ptE}\,r\,dr\,d\theta dz\) equals the volume of the solid \(E.\)

Question

\((-\sqrt{3},-1,-5)\)

Question

\((-1,\sqrt{3},4)\)

Question

\((1,1,\sqrt{2})\)

Question

\((2,-2,4)\)

Question

\(( 2,0,4) \)

Question

\(\left( -1,0,\dfrac{1}{2}\right) \)

Question

\((0,3,4) \)

Question

\(( 0,1,-3) \)

Question

\(\left( 2,\dfrac{\pi }{6},-5\right) \)

Question

\(\left( 4,\dfrac{\pi }{3},3\right) \)

Question

\(( 1,0,8) \)

Question

\(\left( 4,\dfrac{\pi }{6},2\right) \)

Question

\(\left( 2,\dfrac{\pi }{2},0\right) \)

Question

\( \left( -3,\dfrac{\pi }{2},1\right) \)

Question

\(\displaystyle\int_{-1}^{1}\int_{-\sqrt{1-x^{2}}}^{\sqrt{1-x^{2}} }\int_{0}^{\sqrt{1-x^{2}-z^{2}}}dy\,dz\,dx\)

Question

\(\displaystyle\int_{0}^{1}\int_{0}^{\sqrt{1-z^{2}}}\int_{0}^{\sqrt{1-y^{2}-z^{2}} }{\it dx}\,{\it dy}\,{\it dz}\)

Question

\(\displaystyle\int_{0}^{2}\int_{-\sqrt{4-x^{2}}}^{\sqrt{4-x^{2}}}\int_{0}^{ \sqrt{x^{2}+y^{2}}}{\it dz}\,{\it dy}\,{\it dx}\)

Question

\(\displaystyle\int_{0}^{3}\int_{-\sqrt{9-x^{2}}}^{\sqrt{9-x^{2}}}\int_{0}^{\sqrt{ x^{2}+z^{2}}}{\it dy}\,{\it dz}\,{\it dx}\)

Question

\(\displaystyle\int_{\pi /6}^{\pi /2}\int_{0}^{3}\int_{0}^{r\sin \theta }r\csc ^{3}\theta \,{\it dz}\,dr\,d\theta \)

Question

\(\displaystyle\int_{\pi /6}^{\pi /2}\int_{0}^{1}\int_{0}^{\sin \theta }r\cos \theta \sin \theta \,{\it dz}\,dr\,d\theta \)

Question

\(\displaystyle\int_{0}^{\pi /3}\int_{0}^{1}\int_{0}^{e^{-1}}r\,{\it dz}\,dr\,d\theta \)

Question

\(\displaystyle\int_{0}^{\pi /3}\int_{0}^{\sin \theta }\int_{0}^{r\sin \theta }r\,{\it dz}\,dr\,d\theta \)

Question

\(\iiint\limits_{\kern-3ptE}{\it dV},\) where \(E\) is the solid enclosed by the planes \(z=1\) and \(z=4,\) and the cylinders \(x^{2}+y^{2}=1\) and \(x^{2}+y^{2}=9\).

Question

\(\iiint\limits_{\kern-3ptE}{\it dV},\) where \(E\) is the solid enclosed by the \(xy\)-plane, \(z=3,\) and the cylinder \(x^{2}+y^{2}=4\).

Question

\(\iiint\limits_{\kern-3ptE}y{\it dV},\) where \(E\) is the solid enclosed by the planes \(z=1\) and \(z=x+3,\) and the cylinders \(x^{2}+y^{2}=1\) and \( x^{2}+y^{2}=4\).

Question

\(\iiint\limits_{\kern-3ptE}x{\it dV}\), where \(E\) is the solid enclosed by the planes \(z=0\) and \(z=x,\) and the cylinder \(x^{2}+y^{2}=9\).

Question

\(\iiint\limits_{\kern-3ptE}xy{\it dV},\) where \(E\) is the solid enclosed by the surfaces \(z=1-x-y\) and \(z=3-x-y\), whose projection onto the \(xy\)-plane is the circle \(x^{2}+y^{2}=1\).

Question

\(\iiint\limits_{\kern-3ptE}xy{\it dV},\) where \(E\) is the solid enclosed by the surfaces \(z=0\) and \(z=x^{2}+y^2\), whose projection onto the \(xy\)-plane is the circle \(x^{2}+y^{2}=4\).

Question

Volume Find the volume of the solid enclosed by the intersection of the sphere \(x^{2}+y^{2}+z^{2}=9\) and the cylinder \(x^{2}+y^{2}=2\).

Question

Volume Find the volume of the solid enclosed by the intersection of the sphere \(x^{2}+y^{2}+z^{2}=4\) and the cylinder \( x^{2}+y^{2}=2x\).

Question

Volume Find the volume \(V\) of the solid enclosed by \( z=x^{2}+y^{2}\) and \(z=16-x^{2}-y^{2}\).

Question

Volume Find the volume \(V\) of the solid enclosed by \( z=x^{2}+y^{2}\) and \(z=2-x\).

Question

Volume Find the volume \(V\) of the solid enclosed by \(z^{2}=4x\) and \( x^{2}+y^{2}=2x.\)

Question

Mass Find the mass of a homogeneous solid of mass density \( \rho \) in the shape of a sphere of radius \(a\).

Question

Mass Find the mass of a solid in the shape of a sphere of radius \(a\), if the mass density \(\rho \) is proportional to the square of the distance from the center.

Question

Mass Find the mass \(M\) of an object in the shape of a right circular cylinder of height \(h\) and radius \(a\), if its mass density is proportional to the square of the distance from the axis of the cylinder.

Question

Moments of Inertia Find the moments of inertia \(I_{x}\) and \( I_{y}\) for the solid region enclosed by the hemisphere \(z=\sqrt{9-x^{2}-y^{2} }\) and the \(xy\)-plane, if the mass density is proportional to the distance from the \(xy\)-plane.

Question

Center of Mass Find the center of mass of a homogeneous solid in the first octant enclosed by the surface \(z=xy\) and the cylinder \( x^{2}+y^{2}=4\).

Question

Center of Mass Find the center of mass of a homogeneous solid enclosed by the surface \(x^{2}+y^{2}=4z\) and the plane \( z=2 \).

Question

Center of Mass Find the center of mass of a homogeneous solid enclosed by the inside of the sphere \(x^{2}+y^{2}+z^{2}=12\) and above the paraboloid \(z=x^{2}+y^{2}\).

Question

Center of Mass Find the center of mass of a homogeneous solid enclosed by the paraboloid \(z=x^{2}+y^{2}\) and the plane \(z=4\).

Question

Mass Use cylindrical coordinates to find the mass of the homogeneous solid bounded on the sides by \(x^{2}+y^{2}=1\), on the bottom by the \(xy\)-plane, and on the top by \(x^{2}+y^{2}+z^{2}=2\).

Question

Joint Between Two Rods Find the mass of the intersection of two rods with constant mass density \(\rho \) that is formed by the cylinders \( x^{2}+y^{2}=1\) and \(x^{2}+z^{2}=1.\)

Question

Volume of a Mountain The height of a mountain (in \(\text{km}\)) can be approximated by \(z=5.3e^{-( x^{2}+y^{2}) }.\)

1. Sketch the mountain over the region \(x^{2}+y^{2}\leq 4.\)
2. Find the volume of the mountain over the region \(x^{2}+y^{2}\leq 4.\)

Question

\(\displaystyle\int_{0}^{2\pi }\int_{0}^{4}\int_{-r}^{\sqrt{16-r^{2}} }z^{2}r^{5}\cos ^{4}\theta \,{\it dz}\,dr\,d\theta \)

Question

\(\displaystyle\int_{0}^{\pi /2}\int_{0}^{2}\int_{-r^{2}}^{9}z^{2}r^{4}\sin ^{4}\theta \,{\it dz}\,dr\,d\theta \)

Question

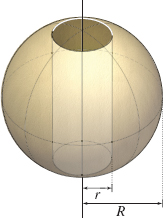
Volume A circular hole of radius \(r\) is drilled through a sphere of radius \(R>r,\) as shown in the figure.

1. Find \(r\) in terms of \(R\) so that the hole removes exactly half of the volume of the sphere. (Hint: set up a volume integral in cylindrical coordinates for the hole.)
2. Find \(r\) rounded to three decimal places if \(R=10\text{cm}.\)

Question

Volume Find the volume enclosed on the top by the sphere \( x^{2}+y^{2}+z^{2}=5\) and on the bottom by the paraboloid \(x^{2}+y^{2}=4z\).

Question

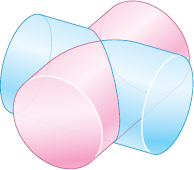
Volume of a Joint Two pipes intersect at right angles as shown in the figure. Find the inner radius \(r\) of the pipes to ensure the volume of the intersecting joint is \(10\text{m}^{3}.\)