1.4 Assess Your Understanding
115
Concepts and Vocabulary
Question
\(\lim\limits_{x\rightarrow 0}\) sin \(x =\) __________
Question
True or False \(\lim\limits_{x\rightarrow 0}\dfrac{\cos x-1}{x} = 1\).
Question
The Squeeze Theorem states that if the functions \(f,g\), and \(h\) have the property \(f(x)\leq g(x)\leq h(x)\) for all \(x\) in an open interval containing \(c\), except possibly at \(c\), and if \(\lim\limits_{x\rightarrow c}f(x) = \) \(\lim\limits_{x\rightarrow c}h(x) = L\), then \(\lim\limits_{x\rightarrow c}g(x) =\) _________.
Question
True or False \(f(x) = \csc x\) is continuous for all real numbers except \(x = 0\).
Skill Building
In Problems 5-8, use the Squeeze Theorem to find each limit.
Question
Suppose \(-x^{2}+1\leq g(x) \leq x^{2}+1\) for all \(x\) in an open interval containing \(0\). Find \(\lim\limits_{x\rightarrow 0}g(x)\).
Question
Suppose \(-(x-2) ^{2}-3\leq g(x)\leq (x-2) ^{2}-3\) for all \(x\) in an open interval containing \(2\). Find \(\lim\limits_{x\rightarrow 2}g(x)\).
Question
Suppose \(\cos x\leq g(x) \leq 1\) for all \(x\) in an open interval containing \(0\). Find \(\lim\limits_{x\rightarrow 0}g(x)\).
Question
Suppose \(-x^{2}+1\leq g(x) \leq \sec x\) for all \(x\) in an open interval containing \(0\). Find \(\lim\limits_{x\rightarrow 0}g(x)\).
In Problems 9-22, find each limit.
Question
\({\lim\limits_{x\rightarrow 0}}({x^{3}+\sin x})\)
Question
\({\lim\limits_{x\rightarrow 0}}({x^{2}-\cos x})\)
Question
\({\lim\limits_{x\rightarrow \pi /3}}({\cos x+\sin x})\)
Question
\({\lim\limits_{x\rightarrow \pi /3}}({\sin x-\cos x})\)
Question
\({\lim\limits_{x\rightarrow 0}}\ \dfrac{\cos x}{1+\sin x}\)
Question
\({\lim\limits_{x\rightarrow 0}}\ \dfrac{\sin x}{1+\cos x}\)
Question
\(\lim\limits_{x\rightarrow 0}\dfrac{3}{1+e^{x}}\)
Question
\(\lim\limits_{x \rightarrow 0}\dfrac{e^{x}-1}{1+e^{x}}\)
Question
\(\lim\limits_{x\rightarrow 0}(e^{x}\sin x)\)
Question
\(\lim\limits_{x\rightarrow 0}(e^{-x}\tan x)\)
Question
\(\lim\limits_{x\rightarrow 1}\ln \left(\dfrac{e^{x}}{x}\right)\)
Question
\(\lim\limits_{x\rightarrow 1}\ln \left(\dfrac{x}{e^{x}}\right)\)
Question
\(\lim\limits_{x\rightarrow 0}\dfrac{e^{2x}}{1+e^{x}}\)
Question
\(\lim\limits_{x\rightarrow 0}\dfrac{1-e^{x}}{1-e^{2x}}\)
In Problems 23-34, find each limit.
Question
\({\lim\limits_{x\rightarrow 0}}\dfrac{\sin (7x)}{x}\)
Question
\({\lim\limits_{x\rightarrow 0}} \dfrac{\sin \dfrac{{x}}{3}}{x}\)
Question
\(\lim\limits_{\theta \rightarrow 0}\dfrac{\theta +3\sin \theta}{2\theta}\)
Question
\({\lim\limits_{x\rightarrow 0}}\ \dfrac{2x-5\sin (3x)}{x}\)
Question
\({\lim\limits_{\theta \rightarrow 0}}\ \dfrac{\sin \theta}{\theta +\tan \theta}\)
Question
\(\lim\limits_{\theta \rightarrow 0}\dfrac{\tan \theta}{\theta}\)
Question
\(\lim\limits_{\theta \rightarrow 0}\dfrac{5}{\theta \cdot \csc \theta}\)
Question
\(\lim\limits_{\theta \rightarrow 0}\dfrac{\sin (3\theta)}{\sin (2\theta)}\)
Question
\(\lim\limits_{\theta \rightarrow 0}\dfrac{1-\cos ^{2}\theta}{\theta}\)
Question
\(\lim\limits_{\theta \rightarrow 0}\dfrac{ \cos (4\theta)-1}{2\theta}\)
Question
\(\lim\limits_{\theta \rightarrow 0}(\theta \cdot \cot \theta)\)
Question
\(\lim\limits_{\theta \rightarrow 0}\left[\sin \theta \left(\dfrac{\cot \theta -\csc \theta}{\theta}\right) \right] \)
In Problems 35-38, determine whether \(f\) is continuous at the number \(c\).
Question
\(f(x)=\left\{ \begin{array}{c@{\quad}l} 3\cos x & \hbox{ if } x\lt 0 \\[4pt] 3 & \hbox{ if }x=0 \\[4pt] x+3 & \hbox{ if }x>0 \end{array} \right. \quad at\quad c=0 \)
Question
\(f(x)=\left\{ \begin{array}{c@{\quad}l} \cos x & \hbox{ if }x\lt 0 \\[4pt] 0 & \hbox{ if }x=0 \\[4pt] e^{x} & \hbox{ if }x\gt 0 \end{array} \right. \quad at\quad c=0\)
Question
\(f(\theta )=\left\{ \begin{array}{l@{\quad}l} \sin \theta & \hbox{ if }\theta \leq\dfrac{\pi }{4} \\[8pt] \cos \theta & \hbox{ if }\theta >\dfrac{\pi }{4} \end{array} \right. at\quad c=\dfrac{\pi }{4}\)
Question
\(f(x)=\left\{ \begin{array}{c@{\quad}l} \tan ^{-1}x & \hbox{ if }x\lt 1 \\[4pt] \ln x & \hbox{ if }x\geq 1 \end{array} \right. at\quad c=1\)
In Problems 39-46, determine where \(f\) is continuous.
Question
\(f(x) = \sin \left(\dfrac{x^{2}-4x}{x-4}\right)\)
Question
\(f(x) = \cos \left(\dfrac{x^{2}-5x+1}{2x}\right)\)
Question
\(f(\theta) = \dfrac{1}{1+\sin \theta}\)
Question
\(f(\theta) = \dfrac{1}{1+\cos^{2}\theta}\)
Question
\(f(x) = \dfrac{\ln x}{x-3}\)
Question
\(f(x) = \ln (x^{2}+1)\)
Question
\(f(x) = e^{-x}\sin x\)
Question
\(f(x) = \dfrac{e^{x}}{1+\sin ^{2}x}\)
Applications and Extensions
In Problems 47-50, use the Squeeze Theorem to find each limit.
Question
\(\lim\limits_{x\rightarrow 0}\left(x^{2}\sin \dfrac{1}{x}\right)\)
Question
\(\lim\limits_{x\rightarrow 0}\left[ x\left(1-\cos \dfrac{1}{x}\right) \right]\)
Question
\(\lim\limits_{x\rightarrow 0}\left[ x^{2}\left(1-\cos \dfrac{1}{x}\right) \right]\)
Question
\(\lim\limits_{x\rightarrow 0}\left[ \sqrt{x^{3}+3x^{2}}\sin \left(\dfrac{1}{x}\right) \right]\)
In Problems 51-54, show that each statement is true.
Question
\(\lim\limits_{x\rightarrow 0}\dfrac{\sin (ax)}{\sin (bx)} = \dfrac{a}{b}\); \(b\neq0\)
Question
\(\lim\limits_{x\rightarrow 0}\dfrac{\cos (ax)}{\cos (bx)} = 1\qquad\)
Question
\(\lim\limits_{x\rightarrow 0}\dfrac{\sin (ax)}{bx} = \dfrac{a}{b}\); \(b\neq 0\)
Question
\(\lim\limits_{x\rightarrow 0}\dfrac{1-\cos (ax)}{bx} = 0\) \(a\neq 0\), \(b\neq 0\)
Question
Projectile Motion An object is propelled from ground level at an angle \(\theta \), \(\dfrac{\pi}{4}\lt \theta \lt \dfrac{\pi}{2}\), up a ramp that is inclined to the horizontal at an angle of \(45^{\circ}\). See the figure. If the object has an initial velocity of 10 feet/second, the equations of the horizontal position \(x = x(\theta)\) and the vertical position \(y = y(\theta)\) of the object after \(t\) seconds are given by \begin{equation*} x = x(\theta) = (10\cos \theta) t \quad \hbox{and} \quad y = y(\theta) = -16t^{2}+ (10\sin \theta) t \end{equation*}
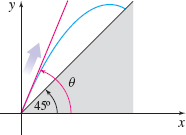
116
For \(t\) fixed,
- Find \(\lim\limits_{\theta \rightarrow {\pi}/{4}^{+}}x(\theta)\) and \(\lim\limits_{\theta \rightarrow {\pi}/{4} ^{+}}y (\theta)\).
- Are the limits found in (a) consistent with what is expected physically?
- Find \(\lim\limits_{\theta \rightarrow {\pi}/{2}^{-}}x(\theta)\) and \(\lim\limits_{\theta \rightarrow {\pi}/{2}^{-}}y(\theta)\).
- Are the limits found in (c) consistent with what is expected physically?
Question
Show that \(\lim\limits_{x\rightarrow 0}\dfrac{1-\cos x}{ x^{2}} = \dfrac{1}{2}\).
Question
Squeeze Theorem If \(0\leq f(x)\leq 1\) for every number \(x\), show that \(\lim\limits_{x\rightarrow 0}[ x^{2}f(x)] = 0\).
Question
Squeeze Theorem If \(0\leq f(x)\leq M\) for every \(x\), show that \(\lim\limits_{x\rightarrow 0}[ x^{2}f(x)] = 0\).
Question
The function \(f(x) = \dfrac{\sin (\pi x)}{x}\) is not defined at \(0\). Decide how to define \(f(0)\) so that \(f\) is continuous at \(0\).
Question
Define \(f(0)\) and \(f(1)\) so that the function \(f(x) = \dfrac{\sin (\pi x)}{x(1-x)}\) is continuous on the interval $[0,1]\).
Question
Is \(f(x)=\left\{ \begin{array}{c@{\quad}l} \dfrac{\sin x}{x} & \hbox{if }x\neq 0 \\[10pt] 1 & \hbox{if }x=0 \end{array} \right.\) continuous at 0?
Question
Is \(f(x) = {\left\{ \begin{array}{c@{\quad}l} \dfrac{1-\cos x}{x} & \hbox{if }x\neq 0 \\[10pt] 0 & \hbox{if }x = 0 \end{array} \right.}\) continuous at \(0\)?
Question
Squeeze Theorem Show that \(\lim\limits_{x\rightarrow0}\left[x^{n}\sin \left(\dfrac{1}{x}\right) \right] = 0\), where \(n\) is a positive integer. (Hint: Look first at Problem 57.)
Question
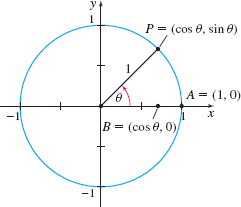
Prove \(\lim\limits_{\theta \rightarrow 0}\sin \theta = 0.\) (Hint: Use a unit circle as shown in the figure, first assuming \(0\lt \theta \,\lt \dfrac{\pi}{2}\). Then use the fact that \(\sin \theta\) is less than the length of the arc \(AP\), and the Squeeze Theorem, to show that \(\lim\limits_{\theta \rightarrow 0^{+}}\sin \theta = 0\). Then use a similar argument with \(-\dfrac{\pi}{2}\lt \theta \lt 0\) to show \(\lim\limits_{\theta \rightarrow 0^{-}}\sin \theta = 0.)\)
Question
Prove \(\lim\limits_{\theta \rightarrow 0}\cos \theta = 1\). Use either the proof outlined in Problem 64 or a proof using the result \(\lim\limits_{\theta \rightarrow 0}\sin \theta = 0\) and a Pythagorean identity.
Question
Without using limits, explain how you can decide whether \(f(x) = \cos (5x^{3}+2x^{2}-8x+1)\) is continuous.
Question
Explain the Squeeze Theorem. Draw a graph to illustrate your explanation.
Challenge Problems
Question
Use the Sum Formulas \(\sin (a+b) = \sin a\cos b+\cos a\sin b\) and \(\cos (a+b) = \cos a\cos b-\sin a\sin b\) to show that the sine function and cosine function are continuous on their domains.
Question
Find \(\lim\limits_{x\rightarrow 0}\dfrac{\sin x^{2}}{x}\).
Question
Squeeze Theorem If \(f(x)=\left\{ \begin{array}{l@{\quad}l} 1 & \hbox{if }x\hbox{ is rational} \\[3pt] 0 & \hbox{if }x\hbox{ is irrational} \end{array} \right.\) show that \(\lim\limits_{x\rightarrow 0}[ xf(x)] =0\).
Question
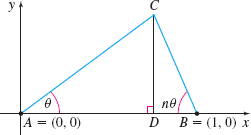
Suppose points \(A\) and \(B\) with coordinates \((0,0)\) and \((1,0)\), respectively, are given. Let \(n\) be a number greater than \(0\), and let \(\theta \) be an angle with the property \(0\lt \theta \lt \dfrac{\pi}{1+n}\). Construct a triangle \(ABC\) where \(\overline{AC}\) and \(\overline{AB}\) form the angle \(\theta \), and \(\overline{CB}\) and \(\overline{AB}\) form the angle \(n\,\theta \) (see the figure below). Let \(D\) be the point of intersection of \(\overline{AB}\) with the perpendicular from \(C\) to \(\overline{AB}\). What is the limiting position of \(D\) as \(\theta \) approaches \(0\)?