Question

True or False The derivative is used to find instantaneous velocity.

Question

True or False The derivative can be used to find the rate of change of a function.

Question

The notation \(f^\prime (c) \) is read \(f\) ______ of \(c\); \(f^\prime (c) \) represents the ______ of the tangent line to the graph of \(f\) at the point ______.

Question

True or False If it exists, \( \lim\limits_{x\rightarrow 3}\dfrac{f( x) -f(3) }{x-3}\) is the derivative of the function \(f\) at \(3.\)

Question

If \(f( x) =x^{2}-3,\) then \(f^\prime (3)\) =______.

Question

Velocity, the slope of a tangent line, and the rate of change of a function are three different interpretations of the mathematical concept called the ______.

Question

Approximating Velocity An object in rectilinear motion moves according to the equation \(s=10t^{2}\) (\(s\) in centimeters). Approximate the velocity of the object at time \(t_{0}=3\) seconds by letting \( \Delta t\) first equal \(0.1\) second, then \(0.01\) second, and finally \(0.001\) second. What limit does the velocity appear to be approaching? Organize the results in a table.

Question

Approximating Velocity An object in rectilinear motion moves according to the equation \(s=5-t^{2}\) (\(s\) in centimeters and \(t\) in seconds). Approximate the velocity of the object at time \(t_{0}=1\) by letting \(\Delta t\) first equal 0.1, then 0.01, and finally 0.001. What limit does the velocity appear to be approaching? Organize the results in a table.

Question

Rectilinear Motion As an object in rectilinear motion moves, the distance \(s\) (in meters) that it moves in \(t_{0}\) seconds is given by \(s=f(t)=3t^{2}+4t.\) Find the velocity \(v\) at \(t_{0}=0\). At \(t_{0}=2\). At any time \(t_{0}\).

Question

Rectilinear Motion As an object in rectilinear motion moves, the distance \(s\) (in meters) that it moves in \(t\) seconds is given by \( s=f(t)=2t^{3}+4\). Find the velocity \(v\) at \(t_{0}=0\). At \(t_{0}=3\). At any time \(t_{0}\).

Question

Rectilinear Motion As an object in rectilinear motion moves, its distance \(s\) from the origin at time \(t\) is given by the equation \( s=s( t) =3t^{2}-\dfrac{1}{t}\), where \(s\) is in centimeters and \(t\) is in seconds. Find the velocity \(v\) of the object at \(t_{0}=1\) and \(t_{0}=4\).

Question

Rectilinear Motion As an object in rectilinear motion moves, its distance \(s\) from the origin at time \(t\) is given by the equation \( s=s( t) =\sqrt{4t}\), where \(s\) is in centimeters and \(t\) is in seconds. Find the velocity \(v\) of the object at \(t_{0}=1\) and \(t_{0}=4\).

Question

\(f(x)=3x^{2}\) at \((-2,12)\)

Question

\(f(x)=x^{2}+2\) at \((-1,3)\)

Question

\(f(x)=x^{3}\) at \((-2,-8)\)

Question

\(f(x)=x^{3}+1\) at \((1,2)\)

Question

\(f(x)={\dfrac{1}{x}}\) at \((1,1)\)

Question

\(f(x)=\sqrt{x}\) at \((4,2)\)

Question

\({f(x)=\dfrac{1}{x+5}}\) at \({\left(1,\dfrac{1}{6}\right) }\)

Question

\({f(x)=\dfrac{2}{x+4}}\) at \({\left(1,\dfrac{2}{5}\right) }\)

Question

\({f(x)=\dfrac{1}{\sqrt{x}},}\) at \({(1,1)}\)

Question

\(f(x)=\dfrac{1}{x^2}\) at \({(1,1)}\)

Question

\(f(x)=5x-2\) at (a) \(c=0,\) (b) \(c=2,\) (c) \(c\) any real number

Question

\(f(x)=x^{2}-1\) at (a) \(c=-1\), (b) \(c=1\), (c) \(c\) any real number

Question

\({f(x)=\dfrac{x^{2}}{x+3}}\) at (a) \(c=0\), (b) \(c=1\), (c) \(c\) any real number, \(c\neq -3\)

Question

\({f(x)=\dfrac{x}{x^{2}-1}}\) at (a) \(c=0\), (b) \(c=2\), (c) \(c\) any real number, \(c\neq \pm 1\)

Question

\(f(x)=2x+3\) at \(1\)

Question

\(f(x)=3x-5\) at \(2\)

Question

\(f(x)=x^{2}-2\) at 0

Question

\(f(x)=2x^{2}+4\) at \(1\)

Question

\(f(x)=3x^{2}+x+5\) at \(-1\)

Question

\(f(x)=2x^{2}-x-7\) at \(-1\)

Question

\(f(x)=\sqrt{x}\) at \(4\)

Question

\(f(x)={\dfrac{1}{x^{2}}}\) at 2

Question

\({f(x)=\dfrac{2-5x}{1+x}}\) at 0

Question

\({f(x)=\dfrac{2+3x}{2+x}}\) at \(1\)

Question

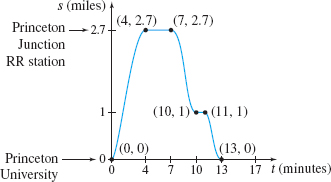
The Princeton Dinky is the shortest rail line in the country. It runs for 2.7 miles, connecting Princeton University to the Princeton Junction railroad station. The Dinky starts from the university and moves north toward Princeton Junction. Its distance from Princeton is shown in the graph where the time \(t\) is in minutes and the distance \(s\) of the Dinky from Princeton University is in miles.

1. When is the Dinky headed toward Princeton University?
2. When is it headed toward Princeton Junction?
3. When is the Dinky stopped?
4. Find its average speed on a trip from Princeton to Princeton Junction.
5. Find its average speed for the round trip shown in the graph, that is, from \(t=0\) to \(t=13.\)
   

Question

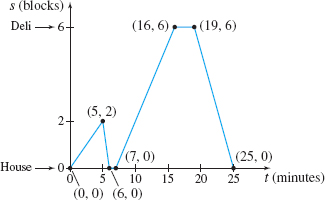
Barbara walks to the deli, which is six blocks east of her house. After walking two blocks, she realizes she left her phone on her desk, so she runs home. After getting the phone, closing and locking the door, Barbara starts on her way again. At the deli, she waits in line to buy a bottle of vitaminwater\(^{\rm TM}\), and then she jogs home. The graph below represents Barbara’s journey. The time \(t\) is in minutes and \(s\) is Barbara’s distance, in blocks, from home.

1. At what times is she headed toward the deli?
2. At what times is she headed home?
3. When is the graph horizontal? What does this indicate?
4. Find Barbara’s average speed from home until she starts back to get her phone.
5. Find Barbara’s average speed from home to the deli after getting her phone.
6. Find her average speed from the deli to home.
   

Question

Slope of a Tangent Line The equation of the tangent line to the graph of a function \(f\) at \((2,6)\) is \(y=-3x+12\). What is \(f^\prime (2)\)?

Question

Slope of a Tangent Line The equation of the tangent line of a function \(f\) at \((3,2)\) is \(y=\dfrac{1}{3}x+1\). What is \(f^\prime (3)\)?

Question

Tangent Line Does the tangent line to the graph of \(y=x^{2}\) at \((1, 1)\) pass through the point \((2, 5)\)?

153

Question

Tangent Line Does the tangent line to the graph of \(y=x^{3}\) at \((1,1)\) pass through the point \((2,5)\)?

Question

Respiration Rate A human being’s respiration rate \(R\) (in breaths per minute) is given by \(R=R\left( p\right) = 10.35+0.59p\), where \(p\) is the partial pressure of carbon dioxide in the lungs. Find the rate of change in respiration when \(p=50\).

Question

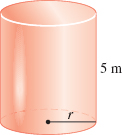
Instantaneous Rate of Change The volume \(V\) of the right circular cylinder of height \(5\)m and radius \(r\)m shown in the figure is \(V=V( r) =5\pi r^{2}.\) Find the instantaneous rate of change of the volume with respect to the radius when \(r=3{m}\).

Question

Market Share During a month-long advertising campaign, the total sales \(S\) of a magazine is modeled by the function \(S(x) =5x^{2}+100x+10{,}000,\) where \(x\) represents the number of days since the campaign began, \(0 ≤ x ≤ 30.\)

1. What is the average rate of change of sales from \(x=10\) to \(x=20\) days?
2. What is the instantaneous rate of change of sales when \(x=10\) days?

Question

Demand Equation The demand equation for an item is \( p=p( x) =90-0.02x,\) where \(p\) is the price in dollars and \(x\) is the number of units (in thousands) made.

1. Assuming all units made can be sold, find the revenue function \( R( x) =xp( x)\).
2. Marginal Revenue Marginal revenue is defined as the additional revenue earned by selling an additional unit. If we use \( R^\prime (x)\) to measure the marginal revenue, find the marginal revenue when 1 million units are sold.

Question

Gravity If a ball is dropped from the top of the Empire State Building, 1002 ft above the ground, the distance \(s\) (in feet) it falls after \(t\) seconds is \(s(t)=16t^{2}\).

1. What is the average velocity of the ball for the first 2s?
2. How long does it take for the ball to hit the ground?
3. What is the average velocity of the ball during the time it is falling?
4. What is the velocity of the ball when it hits the ground?

Question

Velocity A ball is thrown upward. Its height \(h\) in feet is given by \(h(t)=100t-16t^{2}\), where \(t\) is the time elapsed in seconds.

1. What is the velocity \(v\) of the ball at \(t=0{s}\), \(t=1{s}\), and \(t=4{s}\)?
2. At what time \(t\) does the ball strike the ground?
3. At what time \(t\) does the ball reach its highest point? (Hint: At the time the ball reaches its maximum height, it is stationary. So, its velocity \(v= 0\).)

Question

Gravity A rock is dropped from a height of 88.2 m and falls toward Earth in a straight line. In \(t\) seconds the rock falls \( 4.9t^{2}\) meters.

1. What is the average velocity of the rock for the first 2s?
2. How long does it take for the rock to hit the ground?
3. What is the average velocity of the rock during its fall?
4. What is the velocity \(v\) of the rock when it hits the ground?

Question

Velocity At a certain instant, the speedometer of an automobile reads \(V\) mi/h. During the next \(\dfrac{1}{4}\) s the automobile travels 20 ft. Estimate \(V\) from this information.

Question

Volume of a Cube A metal cube with each edge of length \(x\) centimeters is expanding uniformly as a consequence of being heated.

1. Find the average rate of change of the volume of the cube with respect to an edge as \(x\) increases from 2.00 to 2.01cm.
2. Find the instantaneous rate of change of the volume of the cube with respect to an edge at the instant when \(x=2{cm}\).

Question

Rate of Change Show that the rate of change of a linear function \(f( x) =mx+b\) is the slope \(m\) of the line \(y=mx+b.\)

Question

Rate of Change Show that the rate of change of a quadratic function \(f( x) =ax^{2}+bx+c\) is a linear function of \(x\).

Question

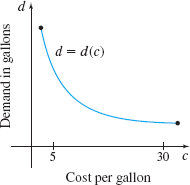
Business The graph represents the demand \(d\) (in gallons) for olive oil as a function of the cost \(c\) in dollars per gallon of the oil.

1. Interpret the derivative \(d^\prime (c)\).
2. Which is larger, \(d^\prime ( 5)\) or \(d^\prime (30)?\) Give an interpretation to \(d^\prime (5)\) and \( d^\prime (30)\).
   

Question

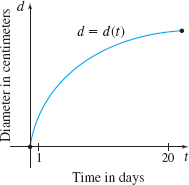
Agriculture The graph represents the diameter \(d\) (in centimeters) of a maturing peach as a function of the time \(t\) (in days) it is on the tree.

1. Interpret the derivative \(d^\prime (t)\) as a rate of change.
2. Which is larger, \(d^\prime (1)\) or \(d^\prime (20)?\)
3. Interpret both \(d^\prime (1)\) and \(d^\prime (20)\).