2.3 Assess Your Understanding
Concepts and Vocabulary
Question
\(\dfrac{d}{dx}\pi ^{2}\)=_____; \(\dfrac{d}{dx}x^{3}\)=_____.
Question
When \(n\) is a positive integer, the Simple Power Rule states that \(\dfrac{d}{dx}x^{n}\)= _____.
Question
True or False The derivative of a power function of degree greater than 1 is also a power function.
Question
If \(k\) is a constant and \(f\) is a differentiable function, then \(\dfrac{d}{dx}[ kf( x) ]\) = _____.
Question
The derivative of \(f( x) =e^{x}\) is _____.
Question
True or False The derivative of an exponential function \(f( x) =a^{x}\), where \(a>0\) and \(a\neq 1\), is always a constant multiple of \(a^{x}\).
Skill Building
In Problems 7–26, find the derivative of each function using the formulas of this section. (a, b, c, and d, when they appear, are constants.)
Question
\(f(x)=3x+\sqrt{2}\)
Question
\(f(x)=5x-\pi\)
Question
\(f(x)=x^{2}+3x+4\)
Question
\(f(x)=4x^{4}+2x^{2}-2\)
Question
\(f(u)=8u^{5}-5u+1\)
Question
\(f(u)=9u^{3}-2u^{2}+4u+4\)
Question
\(f(s)=as^{3}+\dfrac{3}{2}s^{2}\)
Question
\(f(s)=4-\pi s^{2}\)
Question
\(f(t)={\dfrac{1}{3}}(t^{5}-8)\)
Question
\(f(x)=\dfrac{1}{5}(x^{7}-3x^{2}+2)\)
Question
\(f(t)={\dfrac{t^{3}+2}{5}}\)
Question
\(f(x)={\dfrac{x^{7}-5x}{9}}\)
Question
\(f(x)={\dfrac{x^{3}+2x+1}{7}}\)
Question
\(f(x)={\dfrac{1}{a}}(ax^{2}+bx+c)\)
170
Question
\(f(x)=ax^{2}+bx+c\)
Question
\(f(x)=ax^{3}+bx^{2}+cx+d\)
Question
\(f( x) =4e^{x}\)
Question
\(f( x) =-\dfrac{1}{2}e^{x}\)
Question
\(f ( u ) =5u^{2}-2e^{u}\)
Question
\(f ( u ) =3e^{u}+10\)
In Problems 27–32, find each derivative.
Question
\(\dfrac{d}{dt}\left( \sqrt{3}t+\dfrac{1}{2}\right)\)
Question
\({\dfrac{d}{dt}\left( \dfrac{2t^{4}-5}{8}\right) }\)
Question
\({\dfrac{dA}{dR}}\) if \(A( R) =\pi R^{2}\)
Question
\({\dfrac{dC}{dR}}\) if \(C=2\pi R\)
Question
\({\dfrac{dV}{dr}}\) if \(V=\dfrac{4}{3}\pi r^{3}\)
Question
\({\dfrac{dP}{dT}}\) if \(P=0.2T\)
In Problems 33–36:
- Find the slope of the tangent line to the graph of each function f at the indicated point.
- Find an equation of the tangent line at the point.
 Graph f and the tangent line found in (b) on the same set of axes.
Graph f and the tangent line found in (b) on the same set of axes.
Question
\(f(x)=x^{3}+3x-1\) at \((0,-1)\)
Question
\(f(x)=x^{4}+2x-\) 1 at \((1,2)\)
Question
\(f( x) =e^{x}+5x\) at \(( 0,1)\)
Question
\(f( x) =4-e^{x}\) at \(( 0,3)\)
In Problems 37–42:
- Find the points, if any, at which the graph of each function f has a horizontal tangent line.
- Find an equation for each horizontal tangent line.
- Solve the inequality \(f^\prime ( x) > 0\).
- Solve the inequality \(f^\prime ( x) < 0\).
 Graph \(f\) and any horizontal lines found in (b) on the same set of axes.
Graph \(f\) and any horizontal lines found in (b) on the same set of axes.
- Describe the graph of \(f\) for the results obtained in parts (c) and (d).
Question
\(f(x)=3x^{2}-12x+4\)
Question
\(f(x)=x^{2}+4x-3\)
Question
\(f( x) =x+e^{x}\)
Question
\(f( x) =2e^{x}-1\)
Question
\(f(x)=x^{3}-3x+2\)
Question
\(f( x) =x^{4}-4x^{3}\)
Question
Rectilinear Motion At \(t\) seconds, an object in rectilinear motion is \(s\) meters from the origin, where \(s( t) =t^{3}-t+1\). Find the velocity of the object at \(t=0\) and at \(t=5\).
Question
Rectilinear Motion At \(t\) seconds, an object in rectilinear motion is \(s\) meters from the origin, where \(s( t)=t^{4}-t^{3}+1\). Find the velocity of the object at \(t=0\) and at \(t=1\).
Rectilinear Motion In Problems 45 and 46, each function describes the distance s from the origin at time t of an object in rectilinear motion:
- Find the velocity \(v\) of the object at any time \(t\).
- When is the object at rest?
Question
\(s(t)=2-5t+t^{2}\)
Question
\(s(t)=t^{3}-\dfrac{9}{2}t^{2}+6t+4\)
In Problems 47 and 48, use the graphs to find each derivative.
Question
Let \(u( x) =f( x) +g( x)\) and \(v( x) =f( x) -g( x)\).
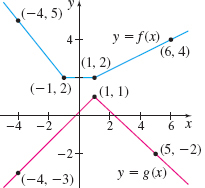
- \(u^\prime ( 0) \)
- \(u^\prime (4) \)
- \(v^\prime ( -2) \)
- \(v^\prime (6) \)
- \(3u^\prime (5) \)
- \(-2v^\prime (3) \)
Question
Let \(F( t) =f( t) +g( t)\) and \(G( t) =g( t) -f( t) \).
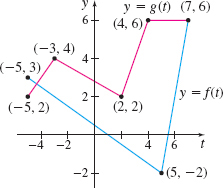
- \(F' ( 0) \)
- \(F' ( 3) \)
- \(F' ( -4) \)
- \(G' ( -2) \)
- \(G' ( -1) \)
- \(G' ( 6) \)
In Problems 49 and 50, for each function f:
- Find \(f^\prime ( x) \) by expanding \(f( x) \) and differentiating the polynomial.
 Find \(f^\prime ( x)\) using a CAS.
Find \(f^\prime ( x)\) using a CAS.
- Show that the results found in parts (a) and (b) are equivalent.
Question
\(f( x) =( 2x-1) ^{3}\)
Question
\(f( x) =( x^{2}+x) ^{4}\)
Applications and Extensions
In Problems 51–56, find each limit.
Question
\({\lim\limits_{h\rightarrow 0}\dfrac{5\left( \dfrac{1}{2}+h\right) ^{8}-5\left( \dfrac{1}{2}\right) ^{8}}{h}}\)
Question
\({\lim\limits_{h\rightarrow 0}\dfrac{6(2+h)^{5}-6(2)^{5}}{h}}\)
Question
\(\lim\limits_{h\rightarrow 0}\dfrac{\sqrt{3}(8+h) ^{5}-\sqrt{3}( 8) ^{5}}{h}\)
Question
\(\lim\limits_{h\rightarrow 0}\dfrac{\pi ( 1+h)^{10}-\pi }{h}\)
Question
\(\lim\limits_{h\rightarrow 0}\dfrac{a( x+h) ^{3}-ax^{3}}{h}\)
Question
\(\lim\limits_{h\rightarrow 0}\dfrac{b( x+h)^{n}-bx^{n}}{h}\)
In Problems 57–62, find an equation of the tangent line(s) to the graph of the function f that is (are) parallel to the line L.
Question
\({f(x)=3x^{2}-x}\); \({L}\): \({y=5x}\)
Question
\({f(x)=2x^{3}+1}\); \({L}\): \({y=6x-1}\)
Question
\(f( x) =e^{x}\); \(L\): \(y-x-5=0\)
Question
\(f( x) =-2e^{x}\); \({L}\): \(y+2x-8=0\)
Question
\(f(x)=\dfrac{1}{3}x^{3}-x^{2}\); \({L}\): \({y=3x-2}\)
Question
\({f(x)=x^{3}-x}\); \(L\): \({x+y=0}\)
171
Question
Tangent Lines Let \(f(x)=4x^{3}-3x-1\).
- Find an equation of the tangent line to the graph of \(f\) at \(x=2\).
- Find the coordinates of any points on the graph of \(f\) where the tangent line is parallel to \(y=x+12\).
- Find an equation of the tangent line to the graph of \(f\) at any points found in (b).
 Graph \(f\), the tangent line found in (a), the line \(y=x+12\), and any tangent lines found in (c) on the same screen.
Graph \(f\), the tangent line found in (a), the line \(y=x+12\), and any tangent lines found in (c) on the same screen.
Question
Tangent Lines Let \(f(x)=x^{3}+2x^{2}+x-1\).
- Find an equation of the tangent line to the graph of \(f\) at \(x=0\).
- Find the coordinates of any points on the graph of \(f\) where the tangent line is parallel to \(y=3x-2\).
- Find an equation of the tangent line to the graph of \(f\) at any points found in (b).
 Graph \(f\), the tangent line found in (a), the line \(y=3x-2\), and any tangent lines found in (c) on the same screen.
Graph \(f\), the tangent line found in (a), the line \(y=3x-2\), and any tangent lines found in (c) on the same screen.
Question
Tangent Line Show that the line perpendicular to the \(x\)-axis and containing the point \((x,y)\) on the graph of \(y=e^{x}\) and the tangent line to the graph of \(y=e^{x}\) at the point \((x,y)\) intersect the \(x\)-axis 1 unit apart. See the figure.
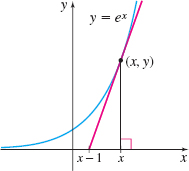
Question
Tangent Line Show that the tangent line to the graph of \(y=x^{n}\) at \((1,1)\) has \(y\)-intercept \(1-n\).
Question
Tangent Lines If \(n\) is an odd positive integer, show that the tangent lines to the graph of \(y=x^{n}\) at \((1,1)\) and at \((-1,-1)\) are parallel.
Question
Tangent Line If the line \(3x-4y=0\) is tangent to the graph of \(y=x^{3}+k\) in the first quadrant, find \(k\).
Question
Tangent Line Find the constants \(a,b\), and \(c\) so that the graph of \(y=ax^{2}+bx+c\) contains the point \((-1,1)\) and is tangent to the line \(y=2x\) at \((0,0)\).
Question
Tangent Line Let \(T\) be the line tangent to the graph of \(y=x^{3}\) at the point \(\left(\dfrac{1}{2},\dfrac{1}{8}\right)\). At what other point \(Q\) on the graph of \(y=x^3\) does the line \(T\) intersect the graph? What is the slope of the tangent line at \(Q\)?
Question
Military Tactics A dive bomber is flying from right to left along the graph of \(y=x^{2}\). When a rocket bomb is released, it follows a path that is approximately along the tangent line. Where should the pilot release the bomb if the target is at \((1,0)\)?
Question
Military Tactics Answer the question in Problem 71 if the plane is flying from right to left along the graph of \(y=x^{3}\).
Question
Fluid Dynamics The velocity \(v\) of a liquid flowing through a cylindrical tube is given by the Hagen–Poiseuille equation \(v=k(R^{2}-r^{2})\), where \(R\) is the radius of the tube, \(k\) is a constant that depends on the length of the tube and the velocity of the liquid at its ends, and \(r\) is the variable distance of the liquid from the center of the tube.
- Find the rate of change of \(v\) with respect to \(r\) at the center of the tube.
- What is the rate of change halfway from the center to the wall of the tube?
- What is the rate of change at the wall of the tube?

Question
Rate of Change Water is leaking out of a swimming pool that measures 20 ft by 40 ft by 6 ft. The amount of water in the pool at a time \(t\) is \(W( t) =35{,}000-20t^{2}\) gallons, where \(t\) equals the number of hours since the pool was last filled. At what rate is the water leaking when \(t=2{h}\)?
Question
Luminosity of the Sun The luminosity \(L\) of a star is the rate at which it radiates energy. This rate depends on the temperature \(T\) and surface area \(A\) of the star’s photosphere (the gaseous surface that emits the light). Luminosity is modeled by the equation \(L=\sigma AT^{4}\), where \(\sigma\) is a constant known as the Stefan–Boltzmann constant, and \(T\) is expressed in the absolute (Kelvin) scale for which 0 K is absolute zero. As with most stars, the Sun’s temperature has gradually increased over the 6 billion years of its existence, causing its luminosity to slowly increase.
- Find the rate at which the Sun’s luminosity changes with respect to the temperature of its photosphere. Assume that the surface area \(A\) remains constant.
- Find the rate of change at the present time. The temperature of the photosphere is presently 5800 K (\(10{,}000{^{\circ}{\rm F}}\)), the radius of the photosphere is \(r=6.96\times 10^{8}{m}\), and \(\sigma =5.67\times 10^{-8}\dfrac{{W}}{{m}^{2}{K}^{4}}\).
- Assuming that the rate found in (b) remains constant, how much would the luminosity change if its photosphere temperature increased by 1 K (1 °C or 1.8 °F)? Compare this change to the present luminosity of the Sun.
Question
Medicine: Poiseuille’s Equation The French physician Poiseuille discovered that the volume \(V\) of blood (in cubic centimeters per unit time) flowing through an artery with inner radius \(R\) (in centimeters) can be modeled by \[ V( R) =kR^{4} \]
where \(k=\dfrac{\pi }{8\nu l}\) is constant (here, \(\nu\) represents the viscosity of blood and \(l\) is the length of the artery).
- Find the rate of change of the volume \(V\) of blood flowing through the artery with respect to the radius \(R\).
- Find the rate of change when \(R=0.03\) and when \(R=0.04\).
- If the radius of a partially clogged artery is increased from 0.03 cm to 0.04 cm, estimate the effect on the rate of change of the volume \(V\) with respect to \(R\) of the blood flowing through the enlarged artery.
- How do you interpret the results found in (b) and (c)?
172
Question
Derivative of the Area Let \(f(x)=mx\), \(m>0\). Let \(F(x)\), \(x>0\), be defined as the area of the shaded region in the figure. Find \(F^{\prime }(x)\).
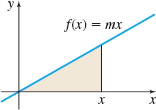
Question
The Difference Rule Prove that if \(f\) and \(g\) are differentiable functions and if \(F(x)=f(x)-g(x)\), then \(F' (x)=f^\prime(x) -g^\prime (x)\).
Question
Simple Power Rule Let \(f(x)=x^{n}\), where \(n\) is a positive integer. Use a factoring principle to show that \[ f^\prime ( c) =\lim\limits_{x\rightarrow c}\dfrac{f( x) -f( c) }{x-c} =nc^{n-1}. \]
Normal Lines Problems 80-87 involve the following discussion.
The normal line to the graph of a function \(f\) at a point \((c,f(c))\) is the line through \((c,f(c))\) perpendicular to the tangent line to the graph of \(f\) at \((c,f(c))\). See the figure. If \(f\) is a function whose derivative at \(c\) is \(f^{\prime }(c)\neq 0\), the slope of the normal line to the graph of \(f\) at \((c,f(c))\) is \(-\dfrac{1}{f^\prime (c)}\). Then an equation of the normal line to the graph of \(f\) at \((c,f(c))\) is \(y-f(c)=-\dfrac{1}{f^\prime (c)} (x-c)\).
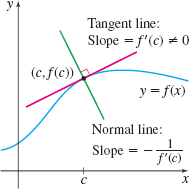
In Problems 80-85, find the slope of the normal line to the graph of each function at the indicated point. Graph each function and show this normal line.
Question
\(f(x)=x^{2}+1\) at \((1,2)\)
Question
\(f(x)=x^{2}-1\) at \((-1,0)\)
Question
\(f(x)=x^{2}-2x\) at \((-1,3)\)
Question
\(f(x)=2x^{2}+x\) at \((1,3)\)
Question
\(f(x)=\dfrac{1}{x}\) at \((1,1)\)
Question
\(f(x)=\sqrt{x}\) at \((4,2)\)
Question
Normal Lines For what nonnegative number \(b\) is the line given by \(y=-\dfrac{1}{3}x+b\) normal to the graph of \(y=x^{3}\)?
Question
Normal Lines Let \(N\) be the line normal to the graph of \(y=x^{2}\) at the point \((-2,4)\). At what other point \(Q\) does \(N\) meet the graph?
Challenge Problems
Question
Tangent Line Find \(a,b,c,d\) so that the tangent line to the graph of the cubic \(y=ax^{3}+bx^{2}+cx+d\) at the point \((1,0)\) is \(y=3x-3\) and at the point \((2,9)\) is \(y=18x-27\).
Question
Tangent Line Find the fourth degree polynomial that contains the origin and to which the line \(x+2y=14\) is tangent at both \(x=4\) and \(x=-2\).
Question
Tangent Lines Find equations for all the lines containing the point \(\left( 1,4\right)\) that are tangent to the graph of \(y=x^{3}-10x^{2}+6x-2\). At what points do each of the tangent lines touch the graph?
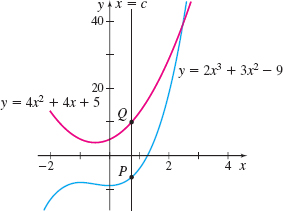
Question
The line \(x=c\), where \(c>0\), intersects the cubic \(y=2x^{3}+3x^{2}-9\) at the point \(P\) and intersects the parabola \(y=4x^{2}+4x+5\) at the point \(Q\), as shown in the figure below.
- If the line tangent to the cubic at the point \(P\) is parallel to the line tangent to the parabola at the point \(Q\), find the number \(c\).
- Write an equation for each of the two tangent lines described in (a).
Question
\(f(x)=Ax^{2}+B\), \(A>0\).
- Find \(c\), \(c>0\), in terms of \(A\) so that the tangent lines to the graph of \(f\) at \((c,f(c))\) and \((-c,f(-c))\) are perpendicular.
- Find the slopes of the tangent lines in (a).
- Find the coordinates, in terms of \(A\) and \(B\), of the point of intersection of the tangent lines in (a).
