Question

The derivative of a composite function \(( f\circ g) ( x) \) can be found using the ______ Rule.

Question

True or False If \(y=f( u) \) and \(u=g( x) \) are differentiable functions, then \(y=f( g( x) ) \) is differentiable.

Question

True or False If \(y=f( g( x) ) \) is a differentiable function, then \(y^\prime =\) \(f^\prime ( g^\prime ( x)) .\)

Question

To find the derivative of \(y=\tan (1+\cos x)\), using the Chain Rule, begin with \(y\) = ______ and \(u\) = ______.

Question

If \(y=( x^{3}+4x+1) ^{100}\), then \(y^\prime =\) ______.

Question

If \(f( x) =e^{3x^{2}+5}\), then \(f^\prime (x) =\) ______.

Question

True or False The Chain Rule can be applied to multiple composite functions.

Question

\(\dfrac{d}{dx}\sin x^{2}=\) ______.

Question

\(y=u^{5}\), \(u=x^{3}+1\)

Question

\(y=u^{3}\), \(u=2x+5\)

Question

\({y=\dfrac{u}{u+1}}\), \({u=x^{2}+1}\)

Question

\({y=\dfrac{u-1}{u}}\), \({u=x^{2}-1}\)

Question

\({y=(u+1)^{2}}\), \({u=\dfrac{1}{x}}\)

Question

\({y=(u^{2}-1)^{3}}\), \({u=\dfrac{1}{x+2}}\)

Question

\(f(x)=(3x+5)^{2}\)

Question

\(f(x)=(2x-5)^{3}\)

Question

\(f(x)=(6x-5)^{-3}\)

Question

\(f(t)=(4t+1)^{-2}\)

Question

\(g(x)=(x^{2}+5)^{4}\)

Question

\(F(x)=(x^{3}-2)^{5}\)

Question

\(f(u)=\left( u-\dfrac{1}{u}\right)^{3}\)

Question

\({f(x)=\left( {x+\dfrac{1}{x}}\right) ^{3}}\)

Question

\(g(x) =( 4x+e^{x}) ^{3}\)

Question

\(F(x) =( e^{x}-x^{2}) ^{2}\)

Question

\(f(x)=\tan ^{2}x\)

Question

\(f(x)=\sec ^{3}x\)

Question

\(f( z) =( \tan z+\cos z) ^{2}\)

Question

\(f( z) =( e^{z}+2\sin z) ^{3}\)

Question

\(y=(x^{2}+4)^{2}(2x^{3}-1)^{3}\)

Question

\(y=(x^{2}-2)^{3}(3x^{4}+1)^{2}\)

Question

\(y=\left( \dfrac{\sin x}{x}\right) ^{2}\)

Question

\(y=\left( \dfrac{x+\cos x}{x}\right) ^{5}\)

Question

\(y=\sin ( 4x) \)

Question

\(y=\cos (5x) \)

Question

\(y=2\sin (x^{2}+2x-1)\)

Question

\(y=\dfrac{1}{2}\cos (x^{3}-2x+5)\)

Question

\(y=\sin \dfrac{1}{x}\)

Question

\(y=\sin \dfrac{3}{x}\)

Question

\(y=\sec ( 4x) \)

Question

\(y=\cot (5x) \)

Question

\(y=e^{1/x}\)

Question

\(y=e^{1/x^{2}}\)

Question

\(y=\dfrac{1}{x^{4}-2x+1}\)

Question

\(y=\dfrac{3}{x^{5}+2x^{2}-3}\)

Question

\(y=\dfrac{100}{1+99e^{-x}}\)

Question

\(y=\dfrac{1}{1+2e^{-x}}\)

Question

\(y=2^{\sin x}\)

Question

\(y=(\sqrt{3}) ^{\cos x}\)

Question

\(y=6^{\sec x}\)

Question

\(y=3^{\tan x}\)

Question

\(y=5xe^{3x}\)

Question

\(y=x^{3}\,e^{2x}\)

Question

\(y=x^{2}\sin (4x) \)

Question

\(y=x^{2}\cos (4x) \)

Question

\(y=e^{-ax}\sin (bx)\)

Question

\(y=e^{ax}\cos (-bx)\)

Question

\(y=\dfrac{e^{ax}-1}{e^{ax}+1}\)

Question

\(y=\dfrac{e^{-ax}+1}{e^{bx}-1}\)

Question

\(y=u^{3}\), \(u=3v^{2}+1\), \(v=\dfrac{4}{x^{2}}\)

Question

\(y=3u\), \(u=3v^{2}-4\), \(v=\dfrac{1}{x}\)

Question

\({y=u^{2}+1}\), \({u=\dfrac{4}{v}},\) \({v=x^{2}}\)

Question

\({y=u^{3}-1}\), \({u=-\dfrac{2}{v}}\), \({v=x^{3}}\)

Question

\(y=e^{-2x}\cos (3x)\)

Question

\(y=e^{\pi x}\tan (\pi x)\)

Question

\(y=\cos(e^{x^{2}})\)

Question

\(y=\tan(e^{x^{2}})\)

Question

\(y=e^{\cos (4x)}\)

Question

\(y=e^{\csc ^{2}x}\)

Question

\(y=4\sin ^{2}(3x)\)

Question

\(y=2\cos ^{2}(x^{2})\)

Question

\(y=(x^{3}+1)^{2}\)

Question

\(y=(x^{2}-2)^{3}\)

Question

\(f(x) =(x^{2}-2x+1)^{5}\) at \((1,0)\)

Question

\(f(x) =(x^{3}-x^{2}+x-1)^{10}\) at \((0,1)\)

Question

\(f(x) {=\dfrac{x}{(x^{2}-1)^{3}}}\) at \({\left({2,\dfrac{2}{27}}\right)}\)

Question

\(f(x) {=\dfrac{x^{2}}{(x^{2}-1)^{2}}}\) at \({\left(2,\dfrac{4}{9}\right) }\)

Question

\(f( x) =\sin (2x) +\cos \dfrac{x}{2}\) at \((0,1) \)

Question

\(f(x) =\sin ^{2}x+\cos ^{3}x\) at \(\left( \dfrac{\pi }{2},1\right)\)

Question

\({\dfrac{d\,^{2}}{dx^{2}}\cos (x^{5})}\)

Question

\({\dfrac{d\,^{3}}{dx^{3}}\sin ^{3}x}\)

Question

Suppose \(h=f\circ g\). Find \(h^\prime (1)\) if \(f^\prime (2)=6,\) \(f(1)=4,\) \(g(1)=2\), and \(g^\prime (1)=-2\).

Question

Suppose \(h=f\circ g\). Find \(h^\prime (1)\) if \(f^\prime (3)=4,\) \(f(1)=1,\) \(g(1)=3\), and \(g^\prime (1)=3\).

Question

Suppose \(h=g\circ f\). Find \(h^\prime (0)\) if \(f(0)=3,\) \(f^\prime (0)=-1,\) \(g(3)=8\), and \(g^\prime (3)=0\).

Question

Suppose \(h=g\circ f\). Find \(h^\prime (2)\) if \(f(1)=2,\) \(f^\prime (1)=4,\) \(f(2)=-3,\) \(f^\prime (2)=4,\,\ g(-3)=1\), and \(g^\prime (-3)=3\).

Question

If \(y=u^{5}+u\) and \(u=4x^{3}+x-4\), find \(\dfrac{dy}{dx}\) at \(x=1\).

Question

If \(y=e^{u}+3u\) and \(u=\cos x\), find \(\dfrac{dy}{dx}\) at \(x=0\).

Question

\({\dfrac{d}{dx} f(x^{2}\,{+}\,1)}\) (Hint: Let \(u\,{=}\,x^{2}\,{+}\,1\).)

Question

\({\dfrac{d}{dx} f(1-x^{2})\qquad }\)

Question

\(\dfrac{d}{dx} f \!\left(\dfrac{x+1}{x-1}\right) \)

Question

\({\dfrac{d}{dx} f\!\left(\dfrac{1-x}{1+x}\right)}\)

Question

\({\dfrac{d}{dx} f(\sin x)}\)

Question

\({\dfrac{d}{dx}f(\tan x)}\)

Question

\({\dfrac{d^{2}}{dx^{2}} f(\cos x)}\)

Question

\(\dfrac{d^{2}}{dx^{2}} f(e^{x})\)

Question

Rectilinear Motion The distance \(s\), in meters, of an object from the origin at time \(t ≥ 0\) seconds is given by \(s=s( t) = A\cos (\omega t+\phi \)), where \(A,\) \(\omega ,\) and \(\phi \) are constant.

1. Find the velocity \(v\) of the object at time \(t\).
2. When is the velocity of the object \(0\)?
3. Find the acceleration \(a\) of the object at time \(t.\)
4. When is the acceleration of the object \(0?\)

Question

Rectilinear Motion A bullet is fired horizontally into a bale of paper. The distance \(s\) (in meters) the bullet travels into the bale of paper in \(t\) seconds is given by \[ s=s( t) =8-(2-t)^{3},\quad 0 ≤ t≤ 2. \]

1. Find the velocity \(v\) of the bullet at any time \(t.\)
2. Find the velocity of the bullet at \(t=\) \(1\) and at \(t=2\).

   207

3. Find the acceleration \(a\) of the bullet at any time \(t\).
4. Find the acceleration of the bullet at \(t=1\) and at \(t=2\).
5. How far into the bale of paper did the bullet travel?

Question

Rectilinear Motion Find the acceleration \(a\) of a car if the distance \(s\), in feet, it has traveled along a highway at time \(t≥ 0\) seconds is given by \[ s( t) =\frac{80}{3}\left[ {{t+\frac{3}{\pi }\sin }}\left( {{\frac{\pi }{6}t}}\right) \right] \]

Question

Rectilinear Motion An object moves in rectilinear motion so that at time \(t≥ 0\) seconds, its distance from the origin is \(s(t) =\sin e^{t}\), in feet.

1. Find the velocity \(v\) and acceleration \(a\) of the object at any time \(t\).
2. At what time does the object first have zero velocity?
3. What is the acceleration of the object at the time \(t\) found in (b)?

Question

Resistance The resistance \(R\) (measured in ohms) of an 80-meter-long electric wire of radius \(x\) (in centimeters) is given by the formula \(R=R(x) =\dfrac{0.0048}{x^{2}}\). The radius \(x\) is given by \(x=0.1991 + 0.000003T\) where \(T\) is the temperature in Kelvin. How fast is \(R\) changing with respect to \(T\) when \(T=320{\,{\rm{K}}}\)?

Question

Pendulum Motion in a Car The motion of a pendulum swinging in the direction of motion of a car moving at a low, constant speed, can be modeled by \[ s=s(t)=0.05\sin (2t)+3t \ \ \ \ \ \ 0≤ t≤ \pi \] where \(s\) is the distance in meters and \(t\) is the time in seconds.

1. Find the velocity \(v\) at \(t=0\), \(t=\dfrac{\pi }{8}\), \(t=\dfrac{\pi }{4}\), \(t=\dfrac{\pi }{2}\), and \(t=\pi \).
2. Find the acceleration \(a\) at the times given in (a).
3. Graph \(s=s(t)\), \(v=v(t)\), and \(a=a(t)\) on the same screen.

Source: Mathematics students at Trine University.

Question

Economics The function \(A(t) =102-90\,e^{-0.21t}\) represents the relationship between \(A\), the percentage of the market penetrated by second-generation smart phones, and \(t\), the time in years, where \(t=0\) corresponds to the year 2010.

1. Find \(\lim\limits_{t\rightarrow \infty}A(t) \) and interpret the result.
2. Graph the function \(A=A( t) ,\) and explain how the graph supports the answer in (a).
3. Find the rate of change of \(A\) with respect to time.
4. Evaluate \(A^\prime (5) \) and \(A^\prime (10) \) and\ interpret these results.
5. Graph the function \(A^\prime =A^\prime ( t) ,\) and explain how the graph supports the answers in (d).

Question

Meteorology The atmospheric pressure at a height of \(x\) meters above sea level is \(P(x)=10^{4}e^{-0.00012x}\) kilograms per square meter. What is the rate of change of the pressure with respect to the height at \(x=500\) m? At \(x=750\) m?

Question

Hailstones Hailstones originate at an altitude of about \(3000{\,{\rm{m}}}\), although this varies. As they fall, air resistance slows down the hailstones considerably. In one model of air resistance, the speed of a hailstone of mass \(m\) as a function of time \(t\) is given by \(v(t)=\dfrac{mg}{k}( 1-e^{-kt/m}) {\,{\rm{m}}}/\!{\,{\rm{s}}}\), where \(g=9.8{\,{\rm{m}}}/\!{\,{\rm{s}}}^{2}\) is the acceleration due to gravity and \(k\) is a constant that depends on the size of the hailstone and the conditions of the air.

1. Find the acceleration \(a(t)\) of a hailstone as a function of time \(t\).
2. Find \(\lim\limits_{t\rightarrow \infty} v(t) \). What does this limit say about the speed of the hailstone?
3. Find \(\lim\limits_{t\rightarrow \infty} a(t)\). What does this limit say about the acceleration of the hailstone?

Question

Mean Earnings The mean earnings \(E\), in dollars, of workers 18 years and over are given in the table below:

1. Find the exponential function of best fit and show that it equals \(E=E( t) =9296(1.05)^{t}\), where \(t\) is the number of years since 1974.
2. Find the rate of change of \(E\) with respect to \(t.\)
3. Find the rate of change at \(t=26\) (year 2000).
4. Find the rate of change at \(t=31\) (year 2005).
5. Find the rate of change at \(t=36\) (year 2010).
6. Compare the answers to (c), (d), and (e). Interpret each answer and explain the differences.

Question

Rectilinear Motion An object moves in rectilinear motion so that at time \(t>0\) its distance \(s\) from the origin is \(s=s( t) .\) The velocity \(v\) of the object is \(v=\dfrac{ds}{dt}, \) and its acceleration is \(a=\dfrac{dv}{dt}=\dfrac{d^{2}s}{dt^{2}}.\) If the velocity \(v=v ( s) \) is expressed as a function of \(s\), show that the acceleration \(a\) can be expressed as \(a=v\dfrac{dv}{ds}.\)

Question

Student Approval Professor Miller’s student approval rating is modeled by the function \(Q( t) =21+\dfrac{10\sin \left(\dfrac{2\pi t}{7}\right) }{\sqrt{t}-\sqrt{20}},\) where \(0≤ t≤ 16\) is the number of weeks since the semester began.

1. Find \(Q^\prime ( t) \).
2. Evaluate \(Q^\prime (1) ,\) \(Q^\prime (5) ,\) and \(Q^\prime \left( 10\right) .\)
3. Interpret the results obtained in (b).
4. Use graphing technology to graph \(Q( t) \) and \(Q^\prime ( t) .\)
5. How would you explain the results in (d) to Professor Miller?

Source: Mathematics students at Millikin University, Decatur, Illinois.

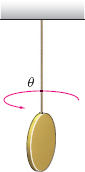
Question

Angular Velocity If the disk in the figure is rotated about the vertical through an angle \(\theta \), torsion in the wire attempts to turn the disk in the opposite direction. The motion \(\theta \) at time \(t\) (assuming no friction or air resistance) obeys the equation \[ \theta ( t) =\dfrac{\pi }{3}\cos \left( \dfrac{1}{2}\sqrt{\dfrac{2k}{5}}t\right) \] where \(k\) is the coefficient of torsion of the wire.

1. Find the angular velocity \(\omega =\dfrac{d\theta }{dt}\) of the disk at any time \(t.\)
2. What is the angular velocity at \(t=3?\)

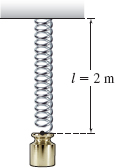
Question

Harmonic Motion A weight hangs on a spring making it \(2{\,{\rm{m}}} \) long when it is stretched out (see the figure). If the weight is pulled down and then released, the weight oscillates up and down, and the length \(l\) of the spring after \(t\) seconds is given by \(l( t) =2+\cos \left(2\pi t\right) \).

1. Find the length \(l\) of the spring at the times \(t=0,\dfrac{1}{2},1,\dfrac{3}{2},\) and \(\dfrac{5}{8}\).
2. Find the velocity \(v\) of the weight at time \(t=\dfrac{1}{4}\).
3. Find the acceleration \(a\) of the weight at time \(t=\dfrac{1}{4}\).

Question

Find \(F^\prime (1)\) if \(f(x)=\sin x\) and \(F(t)=f(t^{2}-1)\).

Question

Normal Line Find the point on the graph of \(y=e^{-x}\) where the normal line to the graph passes through the origin.

Question

Use the Chain Rule and the fact that \({\cos x=\sin \left(\dfrac{\pi }{2}-x\right) }\) to show that \(\dfrac{d}{dx}\cos x=-\sin x.\)

Question

If \(y=e^{2x}\), show that \(y^{\prime\prime} -4y=0\).

Question

If \(y=e^{-2x}\), show that \(y^{\prime\prime} -4y=0\).

Question

If \(y=Ae^{2x}+Be^{-2x}\), where \(A\) and \(B\) are constants, show that \(y^{\prime\prime} -4y=0\).

Question

If \(y=Ae^{ax}+Be^{-ax}\), where \(A\), \(B\), and \(a\) are constants, show that \(y^{\prime\prime} -a^{2}y=0\).

Question

If \(y=Ae^{2x}+Be^{3x}\), where \(A\) and \(B\) are constants, show that \(y^{\prime\prime} -5y^\prime +6y=0\).

Question

If \(y=Ae^{-2x}+Be^{-x}\), where \(A\) and \(B\) are constants, show that \(y^{\prime\prime} +3y^\prime +2y=0\).

Question

If \(y=A~\sin ( \omega t) +B~\cos (\omega t) \), where \(A, B\), and \(\omega \) are constants, show that \(y^{\prime \prime }+\omega ^{2}y=0\).

Question

Show that \(\dfrac{d}{dx}f(h(x))=2xg(x^{2}) \), if \(\dfrac{d}{dx}f(x)=g(x)\) and \(h(x)=x^{2}\).

Question

Find the \(n\)th derivative of \(f(x)=(2x+3)^{n}\).

Question

Find a general formula for the \(n\)th derivative of \(y\).

1. \(y=e^{ax}\)
2. \(y=e^{-ax}\)

Question

1. What is \(\dfrac{d^{10}}{dx^{10}}\sin (ax)\)?
2. What is \(\dfrac{d^{25}}{dx^{25}}\sin (ax) ?\)
3. Find the \(n\)th derivative of \(f(x)=\sin (ax)\).

Question

1. What is \(\dfrac{d^{11}}{dx^{11}}\cos (ax)\)?
2. What is \(\dfrac{d^{12}}{dx^{12}}\cos (ax) ?\)
3. Find the \(n\)th derivative of \(f(x)=\cos (ax)\).

Question

If \(y=e^{-at}~[ A~\sin ( \omega t) +B~\cos ( \omega t) ] \), where \(A\), \(B\), \(a\), and \(\omega \) are constants, find \(y^\prime \) and \(y^{\prime\prime} \).

Question

Show that if a function \(f\) has the properties:

• \(f(u+v)=f(u)f(v)\) for all choices of \(u\) and \(v\)
• \(f(x)=1+xg(x)\), where \(\lim\limits_{x\rightarrow 0}g(x)=1,\)

then \(f^\prime =f\).

Question

Find the \(n\)th derivative of \(f(x)=\dfrac{1}{3x-4}\).

Question

Let \(f_{1}(x),\ldots ,f_{n}(x)\) be \(n\) differentiable functions. Find the derivative of \(y=f_{1}(f_{2}(f_{3}\) \((\ldots (f_{n}(x)\ldots ))))\).

Question

Let \[ f(x)=\left\{ \begin{array}{c@{\qquad}ll} x^{2}\sin {\dfrac{1}{x}} & \hbox{if} & x\;≠ 0 \\ 0 & \hbox{if} & x\;=0 \end{array} \right. \]

Show that \(f^\prime (0)\) exists, but that \(f^\prime (x)\) is not continuous at \(0\).

Question

Define \(f\) by \[ f(x)=\left\{ \begin{array}{l@{\quad}ll} e^{-1/x^{2}} & \hbox{if}& x\;≠ 0 \\ 0 & \hbox{if}& x\;=0 \end{array} \right. \]

Show that \(f\) is differentiable on \((-\infty ,\infty )\) and find \(f^\prime (x) \) for each value of \(x\). [Hint: To find \(f^\prime (0)\), use the definition of the derivative. Then show that \(1< x^{2}e^{1/x^{2}}\) for \(x≠ 0\).]

Question

Suppose \(f( x) =x^{2}\) and \(g( x) =\left\vert x-1\right\vert .\) The functions \(f\) and \(g\) are continuous on their domains, the set of all real numbers.

1. Is \(f\) differentiable at all real numbers? If not, where does \(f^\prime \) not exist?
2. Is \(g\) differentiable at all real numbers? If not, where does \(g^\prime \) not exist?
3. Can the Chain Rule be used to differentiate the composite function \(( f\circ g) (x)\) for all \(x\)? Explain.
4. Is the composite function \(( f\circ g) (x)\) differentiable? If so, what is its derivative?

Question

Suppose \(f( x) =x^{4}\) and \(g( x) =x^{1/3}.\) The functions \(f\) and \(g\) are continuous on their domains, the set of all real numbers.

1. Is \(f\) differentiable at all real numbers? If not, where does \(f^\prime \) not exist?
2. Is \(g\) differentiable at all real numbers? If not, where does \(g^\prime \) not exist?
3. Can the Chain Rule be used to differentiate the composite function \(( f\circ g) (x)\) for all \(x\)? Explain.
4. Is the composite function \(( f\circ g) (x)\) differentiable? If so, what is its derivative?

Question

The function \(f(x)=e^{x}\) has the property \(f^\prime (x)=f(x)\). Give an example of another function \(g(x)\) such that \(g(x)\) is defined for all real \(x\), \(g^{\prime }(x)=g(x)\), and \(g(x)≠ f(x)\).

Question

Harmonic Motion The motion of the piston of an automobile engine is approximately simple harmonic. If the stroke of a piston (twice the amplitude) is \(10{\,{\rm{cm}}}\) and the angular velocity \(\omega \) is \(60\) revolutions per second, then the motion of the piston is given by \(s( t) =5\sin ( 120\pi t ) {\,{\rm{cm}}}.\)

1. Find the acceleration \(a\) of the piston at the end of its stroke \(\left(t=\frac{1}{240} {\rm second}\right)\).
2. If the piston weighs \(1{\,{\rm{kg}}}\), what resultant force must be exerted on it at this point? (Hint: Use Newton’s Second Law, that is, \(F=ma.)\)